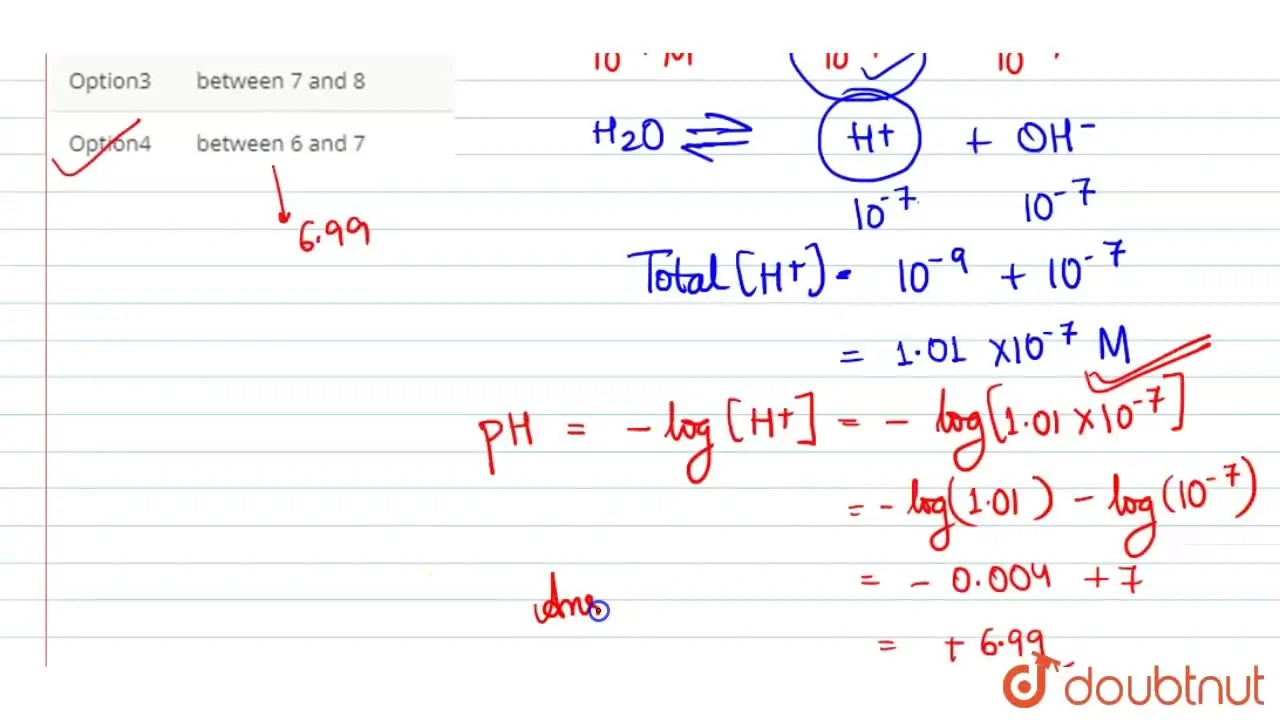
The pH of a 10−9M solution of HCl in water is :
A
8
B
-8
C
between 7 and 8
D
between 6 and 7
Video Solution
Text Solution
The correct Answer is:D
The solution is slightly acidic because concentration of HCl is too low. The concentration of H+ is contributed not only from HCl but also from water. pH is between 6 and 7
|
Updated on:21/07/2023
Similar Questions
The pH of 10−8M solution of HCl in water is
View SolutionThe pH of 10−8M solution of HCl in water is
View SolutionThe pH of a 10−8 molar solution of HCl in water is
View SolutionThe pH of a 10−8 molar solution of HCl in water is
View SolutionThe pH value of a 10 M solution of HCl is
View SolutionpH of a 1.0×10−8 M solution of HCl is
View SolutionCalculate the pH of 10−8 M HCl solution .
View SolutionpH of 10−9 M HCl is
View SolutionThe pH of 0.02 M solution of HCl is
View SolutionCalculate the pH of a 10−5MHCl solution if 1mL of it is diluted to 1000mL. Kw=1×10−14.
View Solution