Which of the following combinations is correct ?
Urms∝√TandUrms∝√M
Urms∝√TandUrms∝1√M
Urms∝1√TandUrms∝1√M
Urms∝1√TandUrms∝√M
The correct Answer is:B
To solve the question regarding which combination is correct concerning the Root Mean Square (RMS) velocity, we can follow these steps:
1. Understand RMS Velocity:
- The Root Mean Square (RMS) velocity is defined as the square root of the average of the squares of the velocities of gas molecules. It is represented by the formula:
vRMS=√3RTM
where R is the universal gas constant, T is the absolute temperature, and M is the molar mass of the gas.
2. Analyze the Relationship:
- From the formula vRMS=√3RTM, we can derive the relationships:
- Directly Proportional to Temperature (T): vRMS is directly proportional to the square root of the temperature, √T.
- Inversely Proportional to Molar Mass (M): vRMS is inversely proportional to the square root of the molar mass, √M.
3. Evaluate the Options:
- Now, we need to evaluate the given options based on the derived relationships:
- Option 1: Both are directly proportional (Incorrect).
- Option 2: Directly proportional to √T and inversely proportional to √M (Correct).
- Option 3: Both are inversely proportional (Incorrect).
- Option 4: Opposite of the correct relationship (Incorrect).
4. Conclusion:
- Based on the analysis, the correct combination is Option 2: vRMS is directly proportional to √T and inversely proportional to √M.
Final Answer:
The correct combination is Option 2.
- The Root Mean Square (RMS) velocity is defined as the square root of the average of the squares of the velocities of gas molecules. It is represented by the formula:
vRMS=√3RTM
where R is the universal gas constant, T is the absolute temperature, and M is the molar mass of the gas.
- From the formula vRMS=√3RTM, we can derive the relationships:
- Directly Proportional to Temperature (T): vRMS is directly proportional to the square root of the temperature, √T.
- Inversely Proportional to Molar Mass (M): vRMS is inversely proportional to the square root of the molar mass, √M.
- Now, we need to evaluate the given options based on the derived relationships:
- Option 1: Both are directly proportional (Incorrect).
- Option 2: Directly proportional to √T and inversely proportional to √M (Correct).
- Option 3: Both are inversely proportional (Incorrect).
- Option 4: Opposite of the correct relationship (Incorrect).
- Based on the analysis, the correct combination is Option 2: vRMS is directly proportional to √T and inversely proportional to √M.
The correct combination is Option 2.
Similar Questions
Knowledge Check

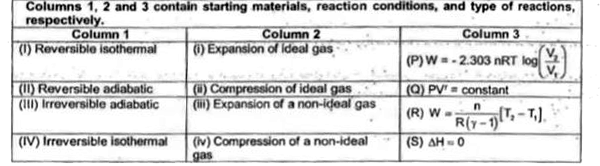
Which of the following combination is correct:AI-b, II-c, III-d, IV-aBI-c, II-b, III-d, IV-aCI-b, II-C, III-a, IV-dDI-d, II-b, III-c, IV-a
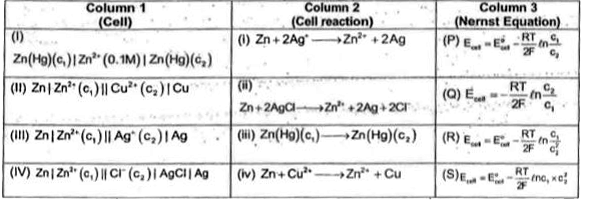
Which of the following combination is correct:AI-b, II-c, III-d, IV-aBI-c, II-b, III-d, IV-aCI-d, II-c, III-c, IV-aDI-d,II-b, III-c, IV-a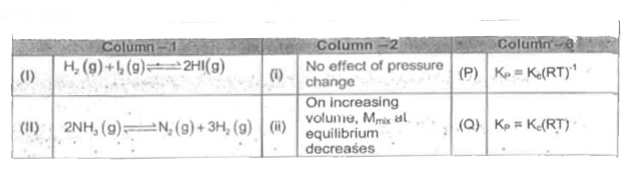

Which of the following combination is correct ?A(I)(iv) (R )B(II) (ii) (R)C(II)(ii) (S)D(III) (ii) (S)
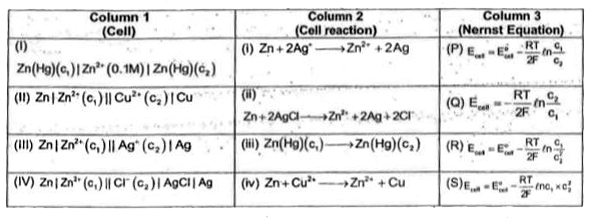
Which of the following combination is correct?
View SolutionModern peroidic table helps classify elements according to their similarities.Consider the columns mentioning certain details about elements with comments on their important features.
Answer the question that follow :
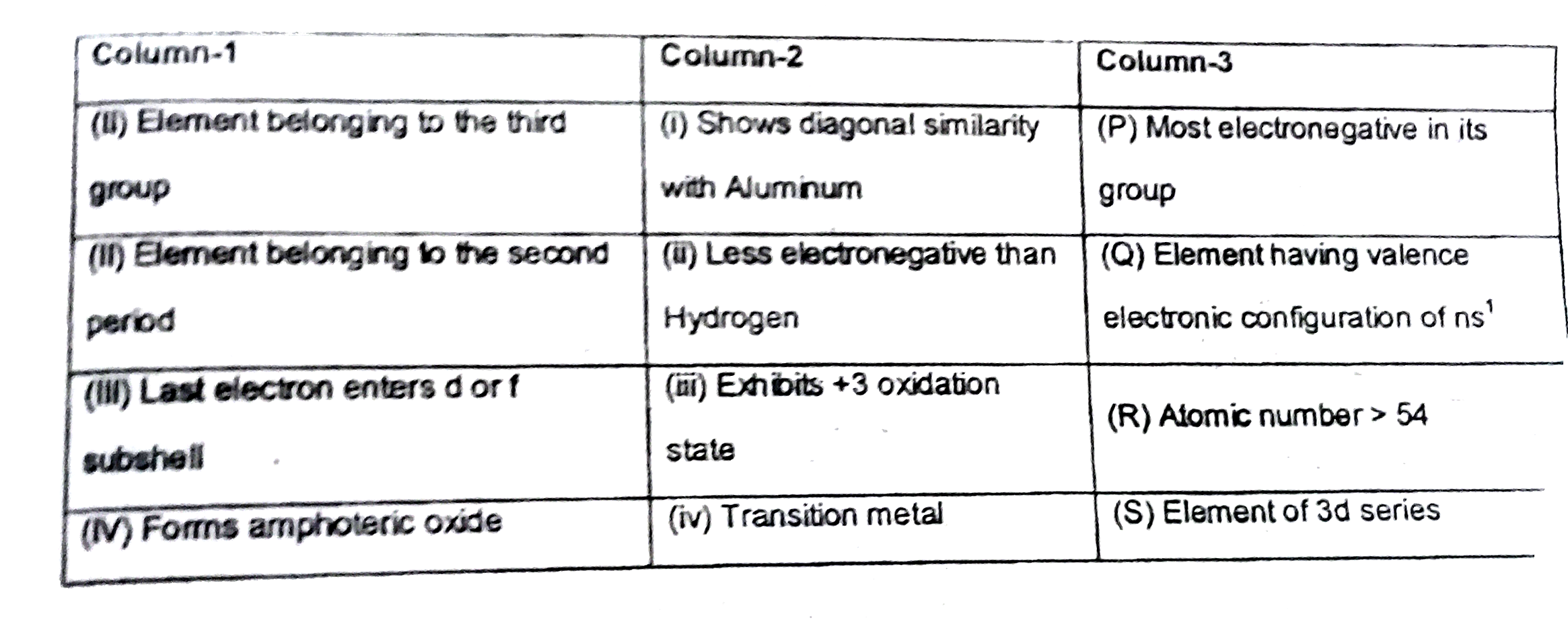
Which of the following combinations is correct for Chromium but not for Zinc ?
View SolutionColumn 1: real valued function, Column-2: domain of the function, Column 3: range of the function. Match the following Column(s) (where [.] denotes the greatest integer function and {.} denotes fractional part function)
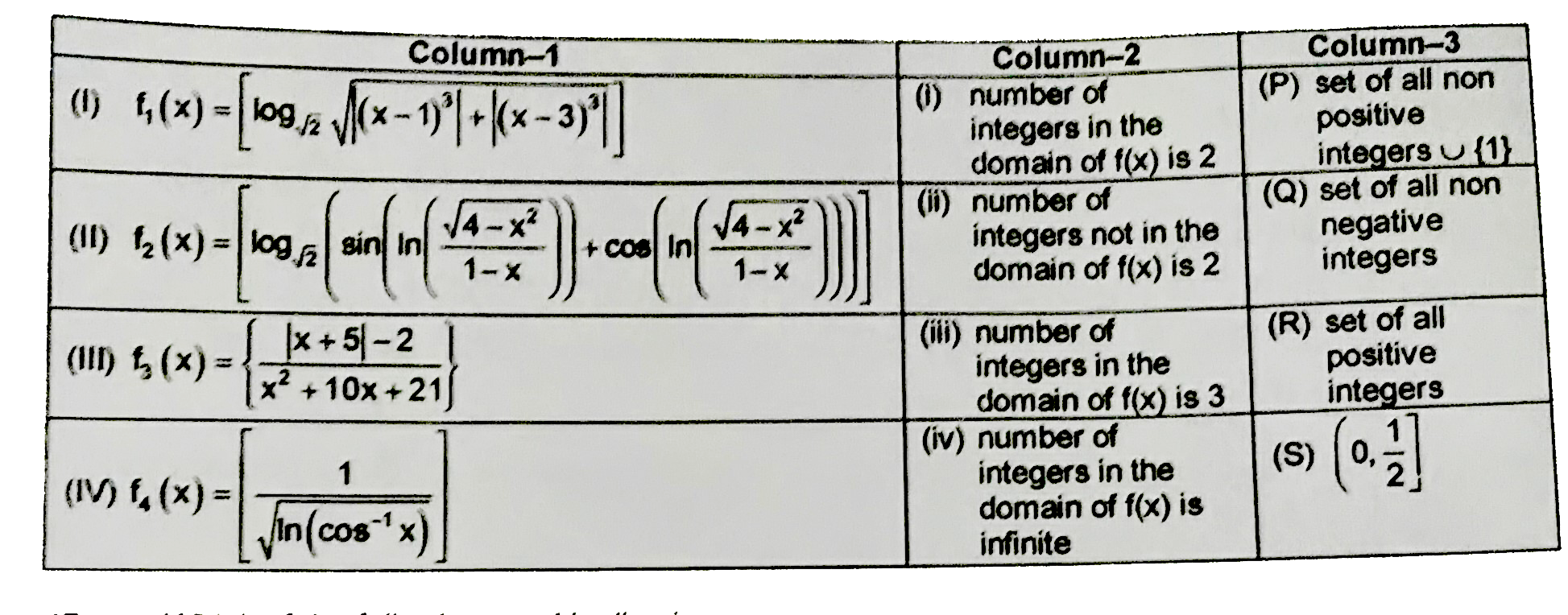
Which of the following combination is correct?
View SolutionColumn 1: real valued function, Column-2: domain of the function, Column 3: range of the function. Match the following Column(s) (where [.] denotes the greatest integer function and {.} denotes fractional part function)
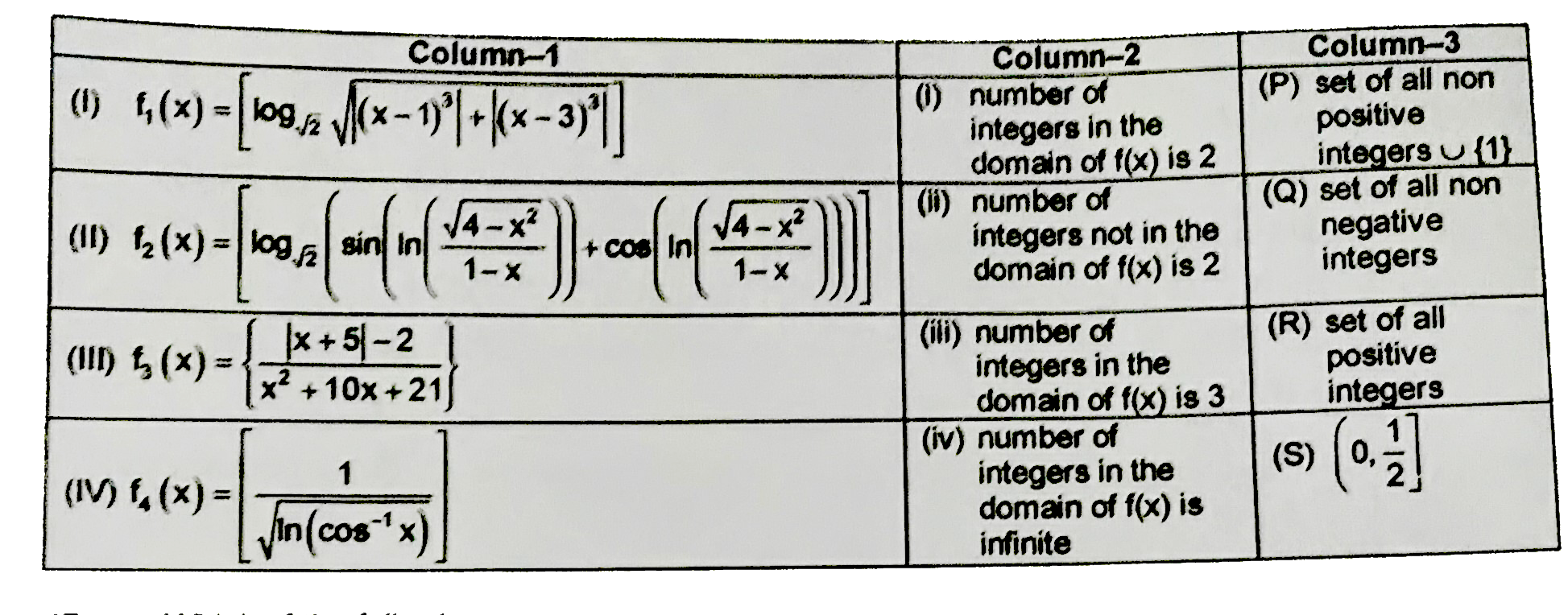
Which of the following combination is correct?
View SolutionColumn 1: real valued function, Column-2: domain of the function, Column 3: range of the function. Match the following Column(s) (where [.] denotes the greatest integer function and {.} denotes fractional part function)
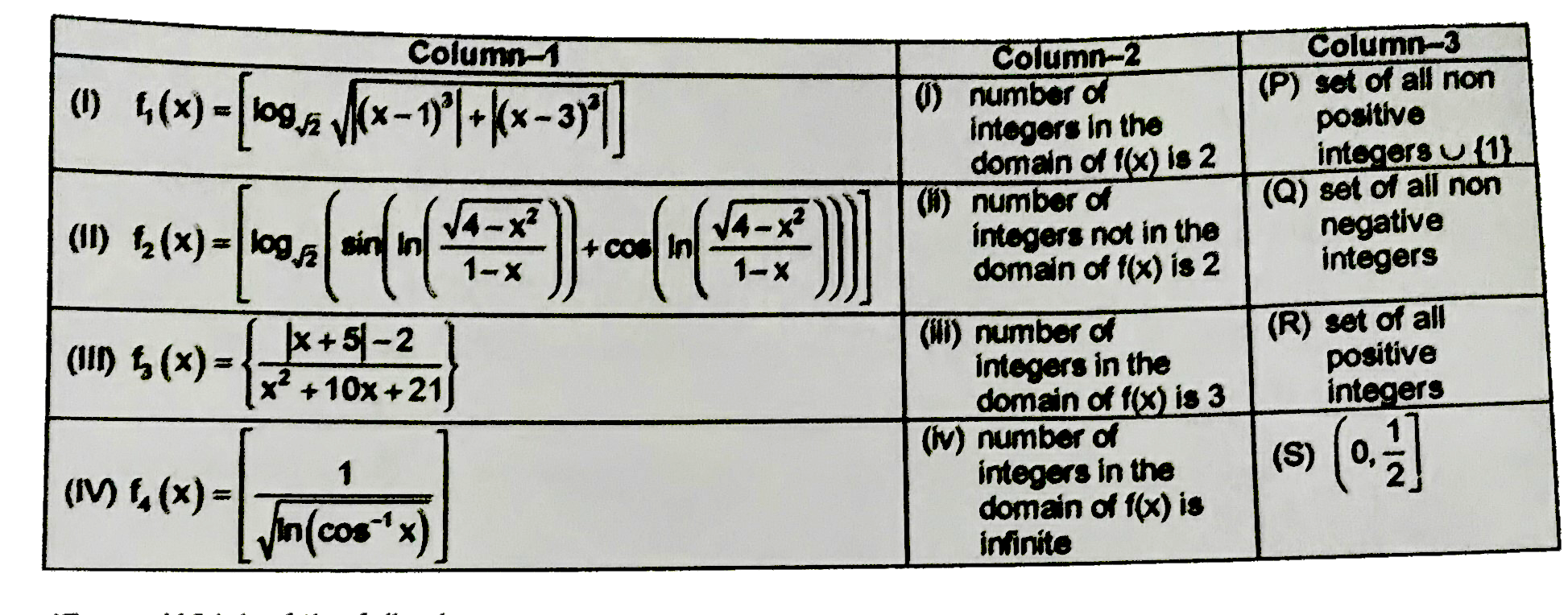
Which of the following combination is correct?
View Solution