The vertices of a triangle are
[at1t2,a(t1+t2)],[at2t3,a(t2+t3)], [at3t1,a(t3+t1)].
Find the orthocentre of the triangle.
The correct Answer is:[−a,a(t1+t2+t3+t1t2t3)]
Step by step video & text solution for The vertices of a triangle are [at_(1)t_(2),a(t_(1)+t_(2))],[at_(2)t_(3),a(t_(2)+t_(3))], [at_(3)t_(1),a(t_(3)+t_(1))]. Find the orthocentre of the triangle. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
If O is the orthocentre of triangle ABC whose vertices are at A(at21,2at1,B(at22,2at2)andC(at23,2at3) then the coordinates of the orthocentreof ΔO'BC are
A(a(t21+t22+t23),2a(t1+t2+t3))B(−a,0)C(at21,2at1)D(0,a)In any progression, if t2t3t1t4=t2+t3t1+t4=3(t2−t3t1−t4), then t1,t2,t3,t4 are in
AA.P.BG.P.CH.P.Dnone
The vertices of a triangle are [at1t2,a(t1+t2)], [at2t3,a(t2+t3)], [at3t1,a(t3+t1)] Then the orthocenter of the triangle is (a) (−a,a(t1+t2+t3)−at1t2t3) (b) (−a,a(t1+t2+t3)+at1t2t3) (c) (a,a(t1+t2+t3)+at1t2t3) (d) (a,a(t1+t2+t3)−at1t2t3)
View SolutionFind the area of that triangle whose vertices are (at21,2at1),(at22,2at2)and(at23,2at3).
View SolutionFind the are of triangle whose vertex are: (at1,at1)(at2,at2)(at3,at3)
View SolutionFind the coordinates o the vertices of a triangle, the equations of whose sides are: y(t1+t2)=2x+2at1at2, y(t2+t3)=2x+2at2t3 and ,y(t3+t1)=2x+2at1t3.
View SolutionProve that the area of the triangle whose vertices are :
(at21,2at1), (at22,2at2), (at23,2at3) is a2(t1−t2)(t2−t3)(t3−t1).
View Solution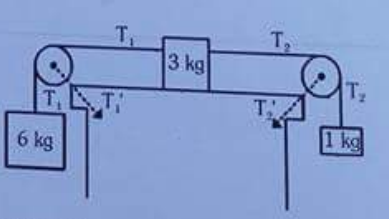
Calculate a, T1,T2,T1'&T2'.
View Solution