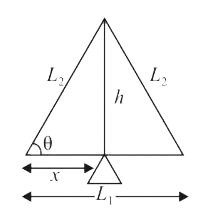
Two rods each of length L2 and coefficient of linear expansion α2 each are connected freely to a third rod of length L1 and coefficient of expansion α1 to form an isoscles triangle. The arrangement is supported on a knife-edge at the midpoint of L1 which is horizontal. what relation must exist between L1 and L2 so that the apex of the isoscles triangle is to remain at a constant height from the knife edge as the temperature changes ?
L1L2=α2α1
L1L2=√α2α1
L1L2=2α2α1
L1L2=2√α2α1
The correct Answer is:D
Here, h remains constant while the set-up is being heated.
but L22=h2+x2
2L2ΔL2=2XΔX for small increment
or L2ΔL2=L12ΔL12⇒4L2ΔL2=L1ΔL1
or,4L22α2ΔT=L21α1ΔTor,L1L2=2√α2α1
Similar Questions
Knowledge Check
If two rods of length L and 2L having coefficients of linear expansion α and 2α respectively are connected so that total length becomes 3L, the average coefficient of linear expansion of the composite rod equals
A32αB52αC53αDNone of theseThere are two rods of length l1 l2 and coefficient of linear expansions are α1 and α2 respectively.
Find equivalent coefficient of thermal expansion for their combination in series.Aα1+α22Bα1l1+α2l2α1+α2C(α1l1+α2l2)/(l1+l2)D√aplha1α2A rod of length l and coefficient of linear expansion α. Find increase in length when temperature changes from T to T+ ΔT
AlαΔTBl(1+αΔT)Cl(1+2αΔT)Dnone of these
A cube of edge (L) and coefficient of linear expansion (α) is heated by 10C. Its surface area increases by
View SolutionTwo metallic rods of length l and 3l have coefficient of linear expansion α and 3α respectively. The coefficient of linear expansion ofr their series combinations, is
View SolutionAn isosceles triangle is formed with a thin rod of length l1 and coefficient of linear expansion α1, as the base and two thin rods each of length l2 and coefficient of linear expansion α2 as the two sides. The distance between the apex and the midpoint of the base remain unchanged as the temperature is varied. the ratio of lengths l1l2 is

View SolutionTwo metal rods of lengths L1 and L2 and coefficients of linear expansion α1 and α2 respectively are welded together to make a composite rod of length (L1+L2) at 0∘C. Find the effective coefficient of linear expansion of the composite rod.
View SolutionTwo rods having length l1andl2 made of materials with the linear expansion coefficient α1andα2 were soldered together. The equivalent coefficients of linear expansion for the obtained rod
View Solution
