A large container (with open top) of negligible mass and uniform cross-sectional area A has a small hole of cross-sectional area a in its side wall near the bottom. The container contains a liquid of density ρ and mass m. If the liquid starts flowing out of the hole at time t = 0, the velocity of efflux of the liquid when 75% of the liquid has drained out is:
√3mg4Aρ
√2mgAρ
2√mgAρ
√mg2Aρ
The correct Answer is:D
To find the velocity of efflux of the liquid when 75% of the liquid has drained out of the container, we can follow these steps:
Step 1: Understand the Problem
We have a large container with a small hole at the bottom. The container is filled with a liquid of density ρ and mass m. We need to determine the velocity of the liquid flowing out of the hole when 75% of the liquid has drained out.
Step 2: Determine the Remaining Volume of Liquid
When 75% of the liquid has drained out, 25% of the liquid remains. If the initial volume of the liquid is V, the remaining volume V′ can be calculated as:
V′=14V
Step 3: Relate Volume to Height
The volume of liquid in the container can be expressed in terms of the cross-sectional area A and the height h of the liquid:
V=A⋅h
Thus, when 75% of the liquid is drained, the height of the remaining liquid h′ is:
V′=A⋅h′=14(A⋅h)
From this, we can derive:
h′=h4
Step 4: Apply Torricelli's Law
According to Torricelli's Law, the velocity v of efflux of a fluid under the force of gravity through a small hole is given by:
v=√2gh′
Substituting h′ from the previous step:
v=√2g⋅h4=√2gh4=√gh2
Step 5: Relate Height to Mass and Density
We know that the mass m of the liquid is related to its density ρ and volume V:
m=ρ⋅V=ρ⋅(A⋅h)
From this, we can express height h as:
h=mρA
Step 6: Substitute Height into Velocity Equation
Substituting h back into the velocity equation:
v=√g⋅mρA2=√gm2ρA
Conclusion
Thus, the velocity of efflux of the liquid when 75% has drained out is:
v=√gm2ρA
We have a large container with a small hole at the bottom. The container is filled with a liquid of density ρ and mass m. We need to determine the velocity of the liquid flowing out of the hole when 75% of the liquid has drained out.
When 75% of the liquid has drained out, 25% of the liquid remains. If the initial volume of the liquid is V, the remaining volume V′ can be calculated as:
V′=14V
The volume of liquid in the container can be expressed in terms of the cross-sectional area A and the height h of the liquid:
V=A⋅h
Thus, when 75% of the liquid is drained, the height of the remaining liquid h′ is:
V′=A⋅h′=14(A⋅h)
From this, we can derive:
h′=h4
According to Torricelli's Law, the velocity v of efflux of a fluid under the force of gravity through a small hole is given by:
v=√2gh′
Substituting h′ from the previous step:
v=√2g⋅h4=√2gh4=√gh2
We know that the mass m of the liquid is related to its density ρ and volume V:
m=ρ⋅V=ρ⋅(A⋅h)
From this, we can express height h as:
h=mρA
Substituting h back into the velocity equation:
v=√g⋅mρA2=√gm2ρA
Thus, the velocity of efflux of the liquid when 75% has drained out is:
v=√gm2ρA
Similar Questions
A large open top container of negligible mass and uniform cross-sectional area A has a small hole of croos-sectional area A100 in its side wall near the bottom.The container is kept on a smooth horizontal floor and contains a liquid of density ρ and mass m0. Assuming that the liquid starts flowing out horizontally through the hole at t=0, The acceleration of the container is x10m/s2 than x is -
View SolutionA large open top container of negligible mass and uniform cross sectional area A a has a small hole of cross sectional area A/100 in its side wall near the bottom. The container is kept on a smooth horizontal floor and contains a liquid of density ρ and mass M0. Assuming that the liquid starts flowing out horizontally through the hole at t=0, calculate a the acceleration of the container and its velocity when 75% of the liquid has drained out.
View Solution
Knowledge Check
A large open top container of negligible mass and uniform ross sectional area A has a small uniform cross sectional area a in its side wall near the bottom. The container is kept over a smooth horizontal floor and contains a liquid of density ρ and mass m0. Assuming that the liqudi starts flowing through the hole A the acceleration of the container will be
A2agABagAC2AgaDAgaThere are two holes, each of cross-sectional area a, on the oppisite side of a wide rectangular tank containing a liquid of density ρ. When the liquid flows out of the holes the net force on the tank is [h is the vertical distance between the two holes].
A2αρghB4αρghC0.5αρghDαρghIn streamline flow of liquid through a pipe of uniform cross sectional area, all stremlines are
Adivided into plane layers.Bdivided into reactangular blocks.Cparallel to the axis of the tube.Dcircular in shape.
There are two holes, each of cross-sectional area a, on the opposite side of a wide rectangular tank containing a liquid of densityρ. When the liquid flows out of the holes, find the net force on the tank. Given h is the vertical distance between two holes.
View SolutionA large container contains a liquid of density p, mass m, and placed on a smooth horizontal floor. The mass of container is negligible and cross sectional area is A. Now at the bottom of the container, there is small hole of cross section area A/200 in the side wall through which the liquid starts flowing horizontally at t=0. What will be the velocity of efflux when half of the liquid is drained out. Also, find the acceleration of the liquid flowing out.
View SolutionFor the area a of the hole is much lesser than the area of the base of a vessel of liquid, velocity of efflux v of the liquid in an accelerating vessel is :
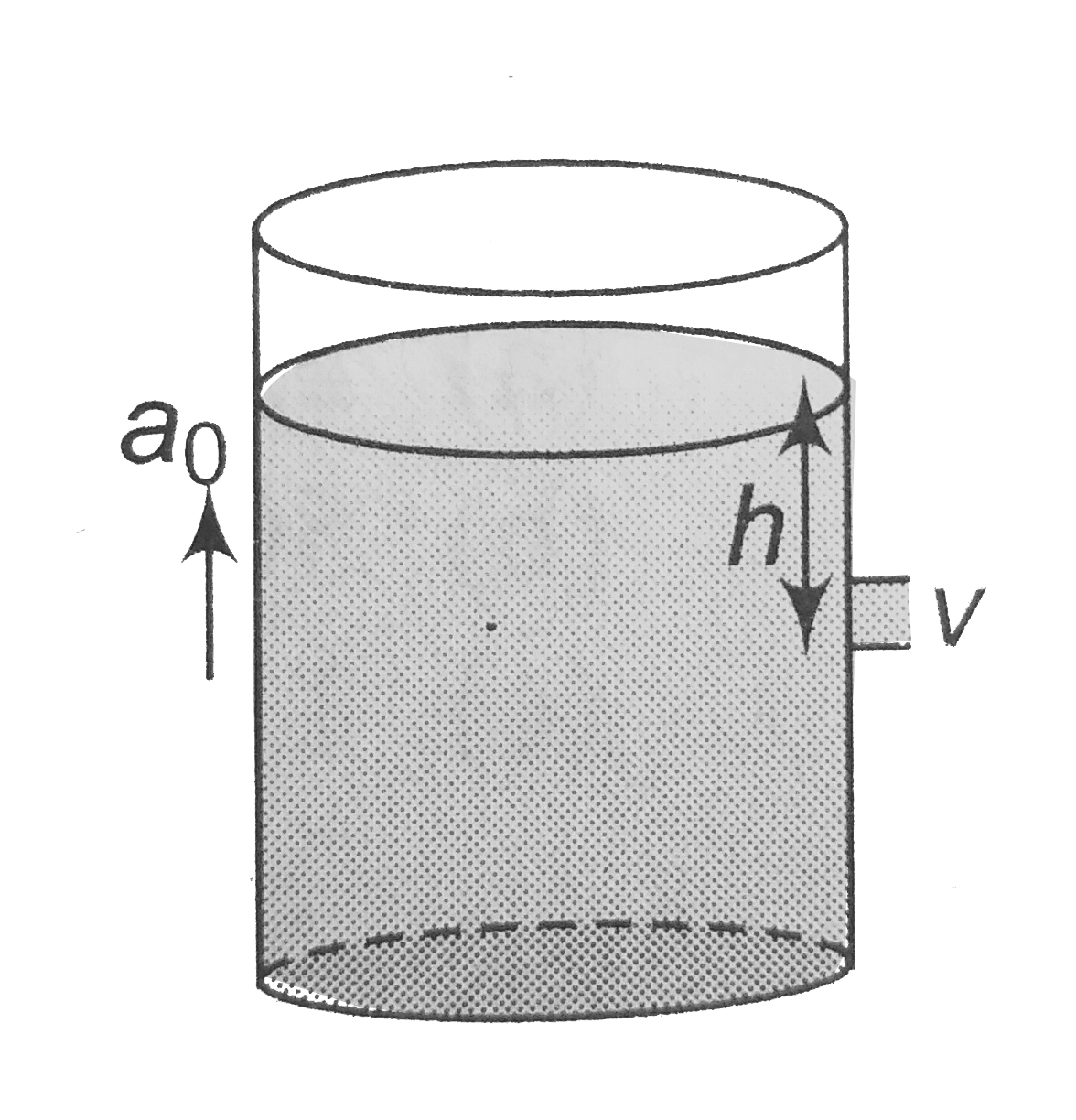
View SolutionThere is a small hole in the bottom of a fixed container containing a liquid upto height 'h'. The top of the liquid as well as the hole at the bottom are exposed to atmosphere. As the liquid come out of the hole. (Area of the hole is 'a' and that of the top surface is 'A') :
View SolutionA liquid flows through a pipe of non-uniform cross-section. If A1andA2 are the cross-sectional areas of the pipe at two points, the ratio of velocities of the liquid at these points will be
View Solution
