The pressure gauge shown in figure has a spring for which k=60N/m and the area of the piston is 0.50cm2. Its right end is connected to a closed container of gas at a gauge pressure of 30kPa. How far will the spring be compressed if the region containing the spring is (a) in vacuum and (b) open to the atmosphere? Atmospheric pressure is 101kPa.
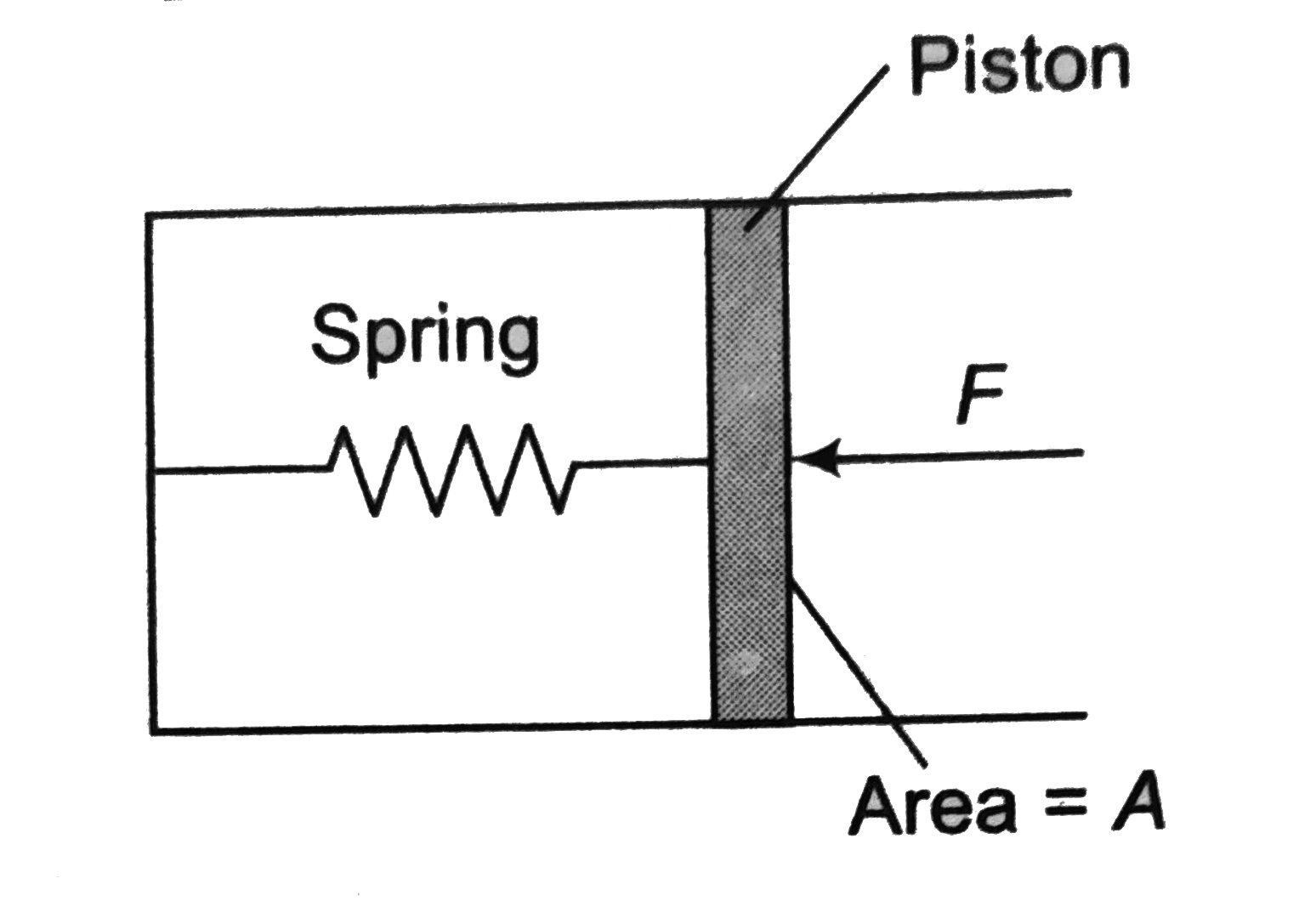
(i) 10.9 cm (ii) 2.5 cm
(i) Pressure to the right of piston, P =(30+101)kPa=131kPa
Compressive force, F =PA=(131×103Pa)(0.50×10−4m2)=6.55N
As F=kx,x=Fk=6.55N60N/m=0.109m=10.9cm
(ii) The force is now due to the gauge pressure (30 kPa) acting on the piston area and is given by
F'=(30×103Pa)(0.50×10−4m2)=1.5N,x=F'k=1.5N60N/m=0.025m=2.5cm
Similar Questions
A smooth air-tight piston connected to a spring of force constant k and unstretched length l separates two regions of a tube as shown in figure. Region A is evacuted and region B is open to the atmosphere. How will you use this set up to determine the atmospheric pressure ?

View SolutionA manometer is connected to a gas containing bulb. The level of mercury in open arm is 2.6 cm lower than that in the other arm of the manometer. What is the pressure of the gas if the atmospheric pressure is 752 mm Hg?
View Solution
Knowledge Check
A U - tube filled with mercury is connected to bulb containing a gas as shown . If atmospheric pressure is 1.013×105 Pa and H=0.05 m , then
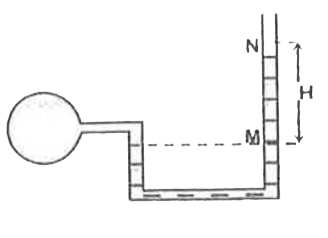 Apressure at M, N and inside bulb are same .Bgauge pressure at N = 0Cgauge pressure at M is 6.8×103 PaDabsolute pressure in bulb is 1.08×05 Pa
Apressure at M, N and inside bulb are same .Bgauge pressure at N = 0Cgauge pressure at M is 6.8×103 PaDabsolute pressure in bulb is 1.08×05 PaA manometer is connected to gas container. Then the mercury level rises by 2 cm in the arm of the manometer which is not connected to the container.
If the atmospheric pressure is 76 cm of mercury, then the pressure of the gas is ________ cm of mercury.A80B76C72D78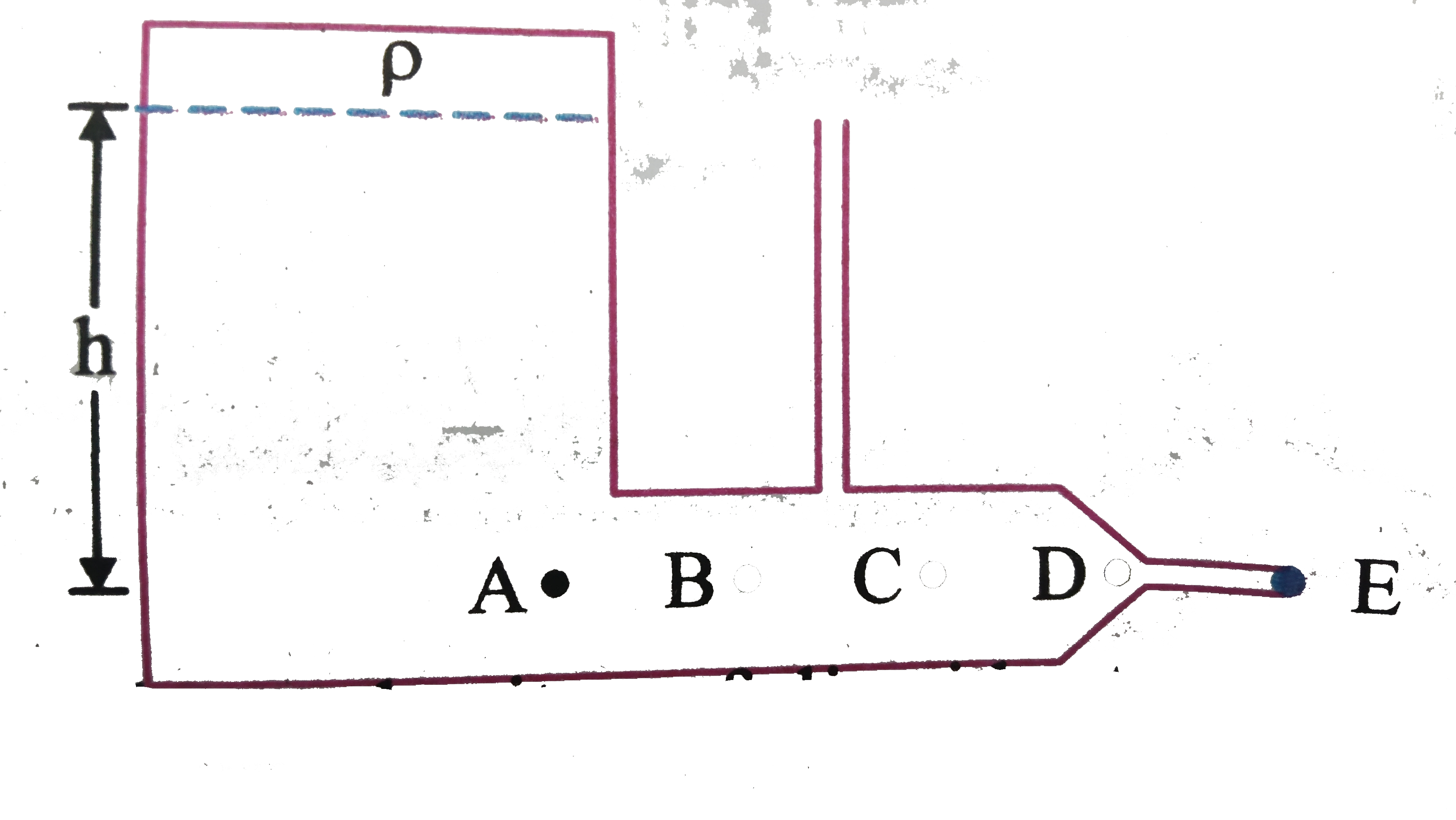
As shown in figure, a liquid of density ρ is standing in sealed container to a height h. The container contains compressed air at a gauge pressure of p.The horizontal outlet pipe has a cross-sectional area A at C and D. The cross-sectional area is A/2 at E. Find correct options:AThe velocity of liquid at C will be [(P+ρgh)4ρ]1/2BThe velocity of liquid at C will be [2(P+ρgh)ρ]1/2Cthe discharge rate is given by A2ρ(p+ρgh)1/2DThe discharge rate is given by A2√ρ(p+ρgh)1/2
Air is contained in a vertical piston – cylinder assembly fitted with an electric heater. The piston has a mass of 50 kg and cross sectional area of 0.1m2. Mass of the air inside the cylinder is 0.3 kg. The heater is switched on and the volume of the air slowly increases by 0.045m3. It was found that the internal energy of the air increased by 32.2kJ/kg and that of the piston increased by 0.06 kJ/kg. Assume that the container walls and outer surface of the piston are well insulated and there is no friction. The atmospheric pressure is 100 kPa. Determine the heat transferred by the heater to the system consisting of air and the piston.
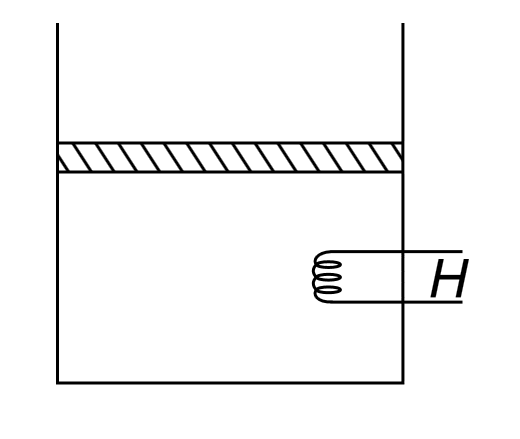
View SolutionA cylinder fitted with a spring loaded piston, shown in the figure, contains 0.01(m)3 gas at a pressure of 105Pa. The cross-sectional area of the piston is 0.05m2. Initially, the spring does not touch the piston but atmospheric pressure of 105Pa acts on the piston. The gas is slowly heated till the volume is increased to three times the original value. If the forcé constant of the spring is 25kNm, calculate the work done (in kJ ) by the gas.
'(##CEN_KSR_PHY_JEE_C15_E01_031_Q19##)'
View SolutionA L Shaped tube containing liquid of density pis accelerated horizontally with acceleration as shown in the figure. The end of tube B is closed while end A is open. If the atmospheric pressure is Po. Then the pressure at B is :
View SolutionA container has a tight fitting movable piston which can slide without friction. The compartment containing spring has vacuum and to the left of the piston there is diatomic gas. If vacuum is created in the right compartment also the piston touches the right wall and the spring is relaxed. Find the heat capacity of the system neglecting the heat capacities of the material of spring, container and the piston. Express your answer in terms of P0,V0 and T0.
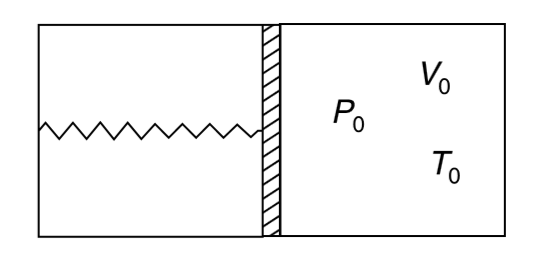
View Solution2.00 mol of a monatomic ideal gas (U=1.5nRT) is enclosed in an adiabatic, fixed , vertical cylinder fitted with a smooth, light adiabatic piston.the piston is connected to a vertical spring of spring constant 200Nm−1 as shown in .the area of cross section of the cylinder is 20.0cm2. initially, the spring is at its natural length and the temperature of the gas is at its natural length and the temperature of the gas is 300 K. the atmosphere pressure is 100 kPa. the gas is heated sloewly for some time by means of an electric heater so as to move the poston up through 10 cm. find (a) the work done by the gas (b) the final temperature of the gas and (c ) the heat supplied by the heater.
View Solution
