An elastic collision takes place between two smooth, rubber balls of same radius as shown. Initially, one ball is at rest and the other is moving with velocity u. At maximum compression :
I. Ratio of potential to initial kinetic energy of the system is cos2θ2
II. Ball B is moving along direction a
III. Ball A is moving along direction d
Evaluate the above statements and choose the correct option from the following:
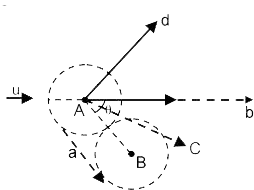
Statements I, II are true and III is false
Statements I, III are false and II is true
All statements are true
All statements are false
The correct Answer is:A
At the time of maximum compression velocities along common normal becomes equal.
Let it be V.
⇒ along common normal: mucosθ=2mv⇒v=ucosθ2
Total KE at this moment
=12{(ucosθ2)2+(usinθ)2}+12m(ucosθ2)2
=12mu2{cos2θ2+sin2θ}=12mu2{1−cos2θ2}
⇒PE=12mu2−12mu2{1−cos22}=12mu2cos2θ2⇒ ratio of PE to intial KE = cos2θ2.
Absolute velocity vector of A lies between common tangent and common normal and velocity vector of B lies along common normal.
Similar Questions
A ball of mass m moving with velocity v collides head on elastically with another identical ball moving with velocity - V. After collision
View SolutionThe figure below shows three identical balls A , B and C. Initially , the baiis B and C are at rest and the ball A while moving with a veiocity v, collides with ball B. if the collision is perfectiy elastic , then after the collision
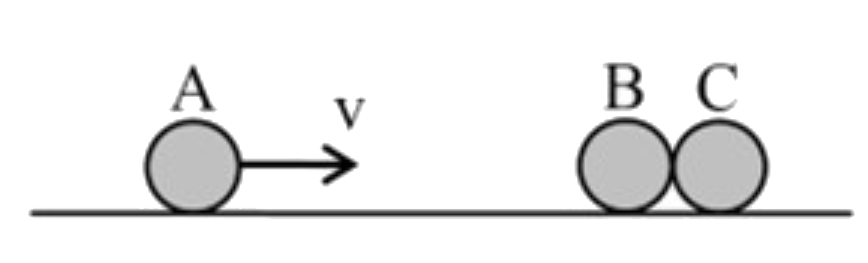
View Solution
Knowledge Check
Two perfectly elastic balls of same mass m are moving with velocities u1 and u2. They collide elastically n times. The kinetic energy of the system finally is:
A12m∪21B12mu(u21+u22)C12m(u21+u22)D12mn(u21+u22)A ball A of mass M collides elastically with a similar ball B at rest as shown in figure. Initially velocity of ball A is u m/s. After collision,
 ASpeed of ball B is u sinθBVelocity of ball A is usin2θˆi+usinθcosθˆjCSpeed of ball A is ucosθDVelocityofballBisu cos theta sin theta hati + u cos^2 theta hatj
ASpeed of ball B is u sinθBVelocity of ball A is usin2θˆi+usinθcosθˆjCSpeed of ball A is ucosθDVelocityofballBisu cos theta sin theta hati + u cos^2 theta hatjA ball impinges directly on another ball at rest and is reduced to rest to rest b y impact. If half the initial kinetic energy is lost by impact, then the coefficient of elasticity is
A1/4B1/3C3/4DNone of these
The mass of a ball A is double the mass of another ball B. The ball a moves at half the speed of the ball. B. Calculate the ratio of the kinetic energy of A to the kinetic energy of B,
View SolutionA ball of mass m moving with vel. V strikes head on elastically with a number of balls of same mass at rest in a line. Only one ball from other side moves with same velocity. Explain wy not two balls move simultaneously, each with ve. v/2 ?
View SolutionA ball of mass m moving with velocity V, makes a head on elastic collision with a ball of the same moving with velocity 2V towards it. Taking direction of V as positive velocities of the two balls after collision are.
View SolutionA ball of mass moving with a velocity u collides head on with a ball B of mass m at rest. If the coefficient of restitution is e. the ratio of final velocity of B to the initial velocity of A is
View SolutionA ball of mass '2m' moving with velocity uˆi collides with another ball of mass 'm' moving with velocity −2uˆi. After the collision, mass 2m moves with velocity u2(ˆi−ˆj). Then the change in kinetic energy of the system ( both balls ) is
View Solution
