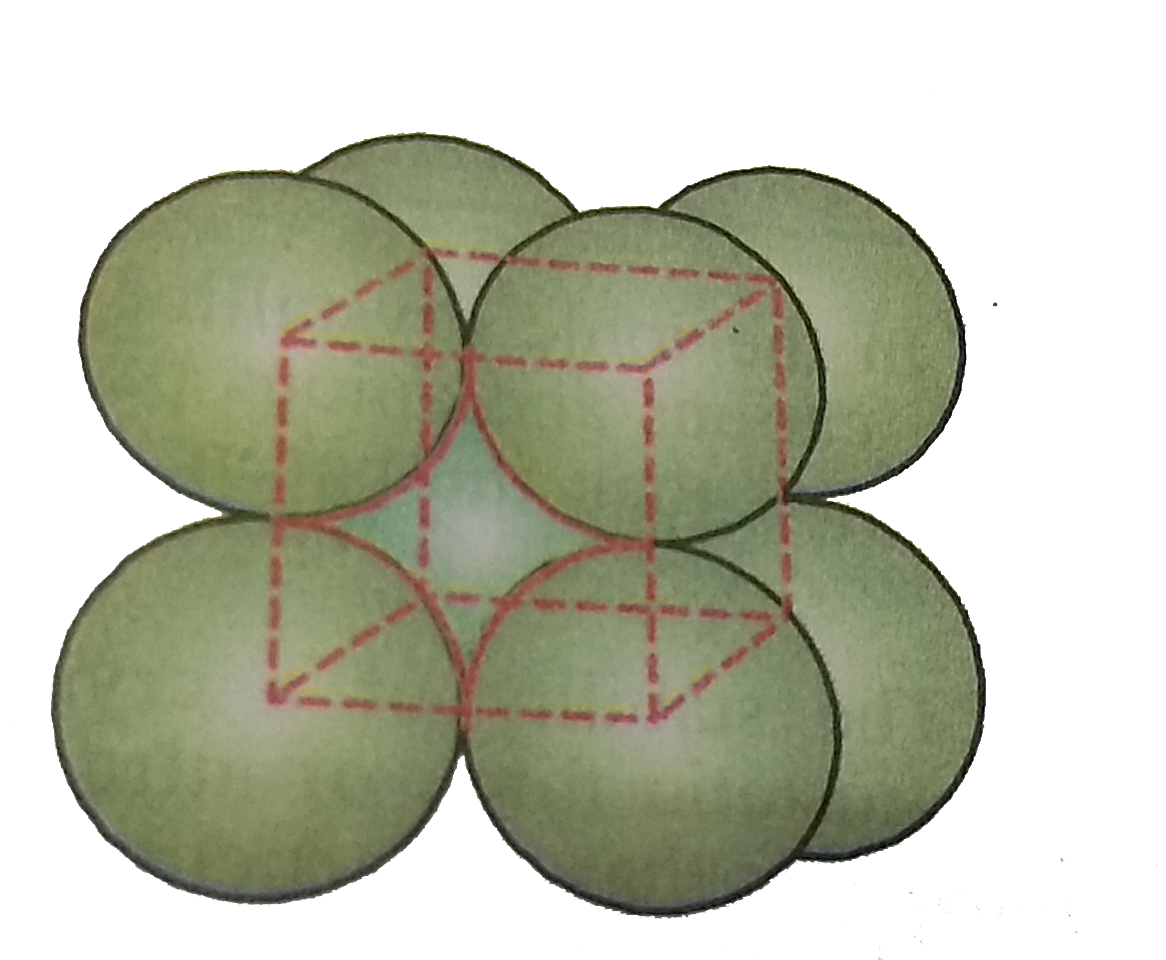
The figure exhibits how to calculate the amount of space used by the atoms in a single cubic structure.
(a) What is the volume of the entire cube?
(b) What is the volume of one sphere?
(c) What fraction of any one sphere sticks into the cube rather than outside it?
(d) Given that there are eight spheres in all, how much space will they take up in the cube ?
Video Solution
Text Solution
(a) Let the ratius of the sphere = a
Edge length = 2a
Volume of the entire cube = (2a)3=8a3
(b) Volume of one sphere =43πa3
(c) Each sphere at the corner is shared by eight cubes. Hence the fraction of any sphere sticking to the cube =18
(d) Space occupied by all the spheres in the cube =8×18×(43πa3)=43πa3
|
Topper's Solved these Questions
- SOLID STATEBOOK - DINESH PUBLICATIONCHAPTER - SOLID STATEEXERCISE - N.C.E.R.T
77 Videos
- SOLID STATEBOOK - DINESH PUBLICATIONCHAPTER - SOLID STATEEXERCISE - N.C.E.R.T.
16 Videos
- REDOX REACTIONSBOOK - DINESH PUBLICATIONCHAPTER - REDOX REACTIONSEXERCISE - Ultimate Preparation
9 Videos
- SOLUTIONS BOOK - DINESH PUBLICATIONCHAPTER - SOLUTIONS EXERCISE - ULTIMATE PREPARATORY PACKAGE
10 Videos
Similar Questions
Knowledge Check
Simple cubic structure represented below .
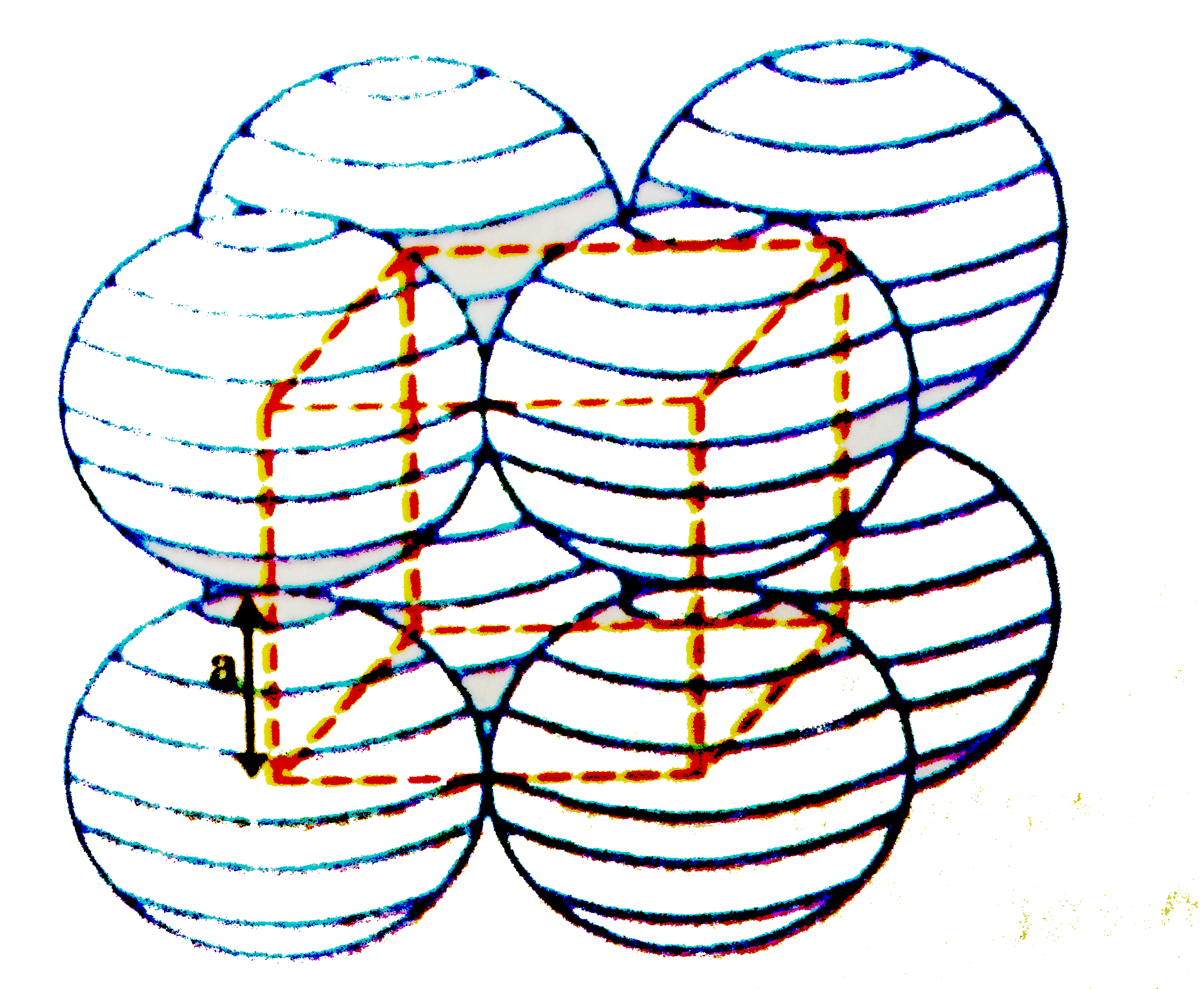
Give that there are eight spheres in all , how much space do they take up in the cube ?A4πa3B4πa3/2C4πa3/3D4πa3/8Simple cubic structure represented below .
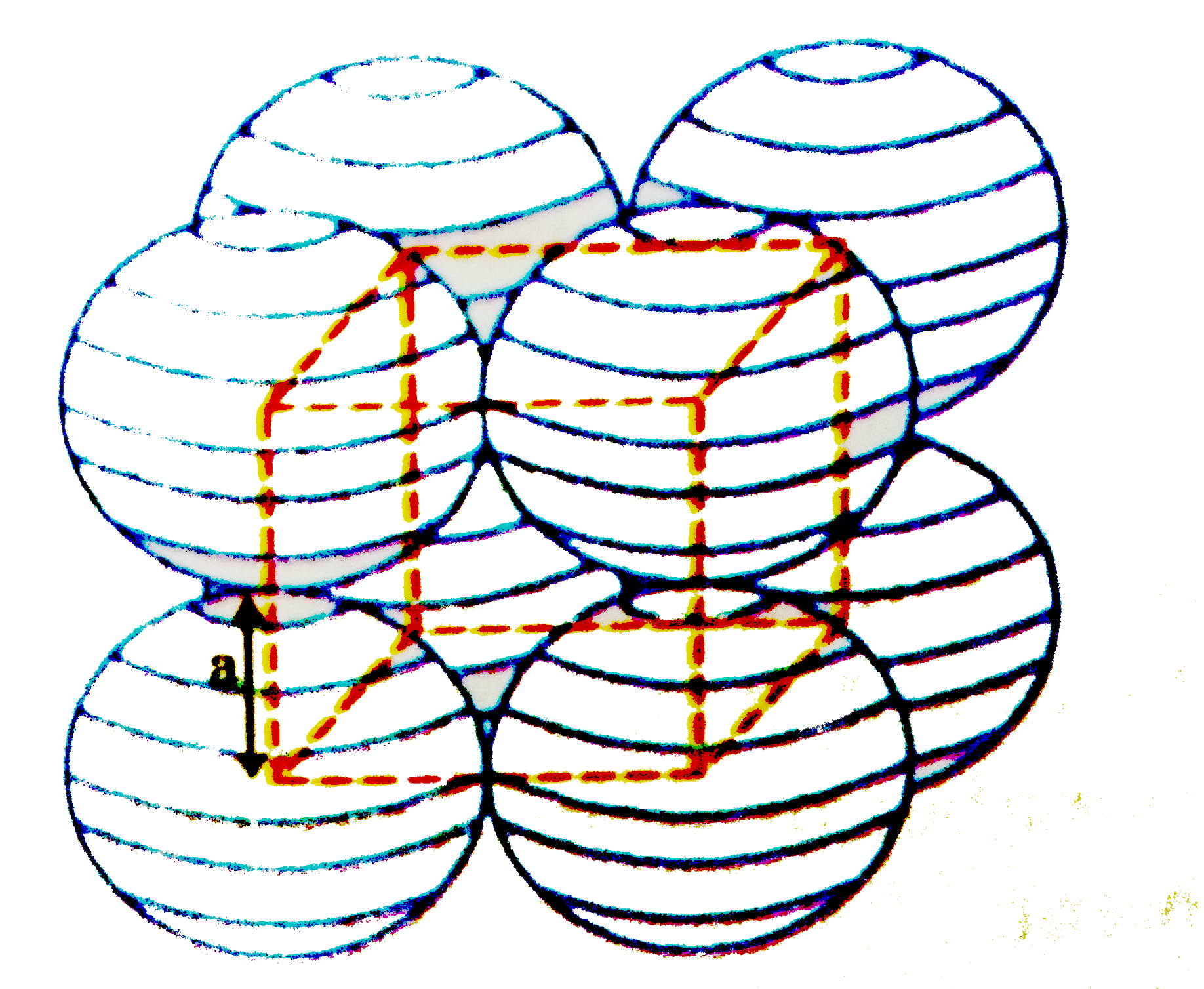
What is the volume of the entire cube ?A2a3B3a3C4a3D8a3What is the volume of the largest sphere that can be curved out of a cube of edge 3 cm?
A9tccm3B6tccm3C4.571cm3D37icm3
What is the volume of the largest sphere that can be carved out of a cube of edge 3 cm?
View SolutionIf the radius of one sphere is twice the radius of second sphere, then find the ratio of their volumes.
View SolutionIn a sphere is inscribed in a cube, find the ratio of the volume of cube to the volume of the sphere.
View SolutionIf a sphere is inscribed in a cube, then prove that the ratio of the volume of the cube to the volume of the sphere will be 6:π .
View SolutionIf the volume of a cube is 729 cubic cm, what is the length of its diagonal?
View Solution
