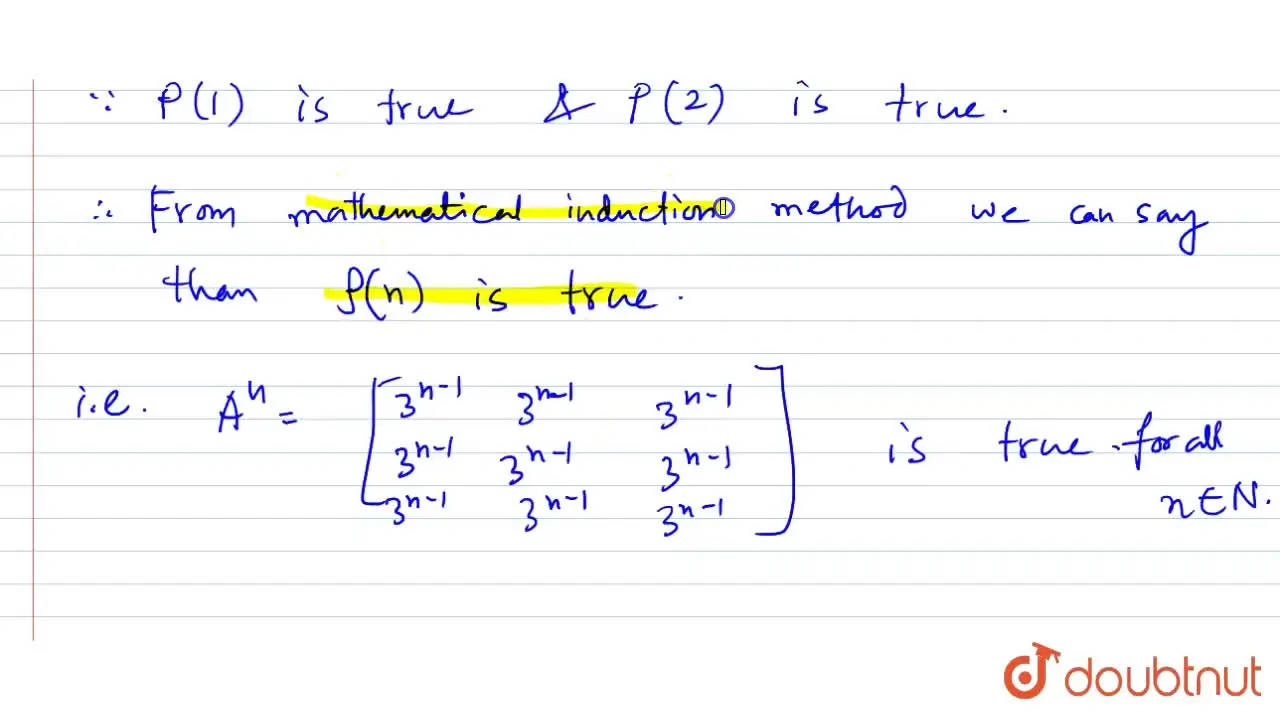
if A=⎡⎢⎣111111111⎤⎥⎦, prove by mathematical induction that,
An=⎡⎢
⎢⎣3n−13n−13n−13n−13n−13n−13n−13n−13n−1⎤⎥
⎥⎦ for every positive integer n.Solution in Bengali
Step by step video & image solution for if A={:[(1,1,1),(1,1,1),(1,1,1)]:}, prove by mathematical induction that, A^(n)={:[(3^(n-1),3^(n-1),3^(n-1)),(3^(n-1),3^(n-1),3^(n-1)),(3^(n-1),3^(n-1),3^(n-1))]:} for every positive integer n. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
If A=⎡⎢⎣111111111⎤⎥⎦, prove that An=⎡⎢ ⎢⎣3n−13n−13n−13n−13n−13n−13n−13n−13n−1⎤⎥ ⎥⎦,n∈N.
08:00View SolutionIf A=⎡⎢⎣111111111⎤⎥⎦, prove that An=⎡⎢ ⎢⎣3n−13n−13n−13n−13n−13n−13n−13n−13n−1⎤⎥ ⎥⎦,n∈N.
05:02View SolutionIf A=⎡⎢⎣111111111⎤⎥⎦, prove that An=⎡⎢ ⎢⎣3n−13n−13n−13n−13n−13n−13n−13n−13n−1⎤⎥ ⎥⎦,n∈N.
04:36View SolutionIf A = [[1,1,1],[1,1,1],[1,1,1]] , Then prove that A^n = [[3^(n-1),3^(n-1),3^(n-1)],[3^(n-1),3^(n-1),3^(n-1)],[3^(n-1),3^(n-1),3^(n-1)]], n in N
08:07View SolutionProve by mathematical induction. 1.1!+2.2!+3.3!+...+n.n!=(n+1)!−1,where n ∈ N.
08:05View SolutionIf A=⎡⎢⎣111111111⎤⎥⎦, prove that An=⎡⎢ ⎢⎣3n−13n−13n−13n−13n−13n−13n−13n−13n−1⎤⎥ ⎥⎦ n∈N.
09:25View Solutionનીયોના આપેલા પ્રશ્ર્ન નંબર 9 થી 14 ની માગ્યા મુજખ ગણતરી કરી જવાખ આપો ( દરેકના 3 ગુણ છે.)
જો A=⎡⎢⎣111111111⎤⎥⎦ હોય ,તો સાખિત કરો કે An=⎡⎢ ⎢⎣3n−13n−13n−13n−13n−13n−13n−13n−13n−1⎤⎥ ⎥⎦,n∈N=07:32View Solution