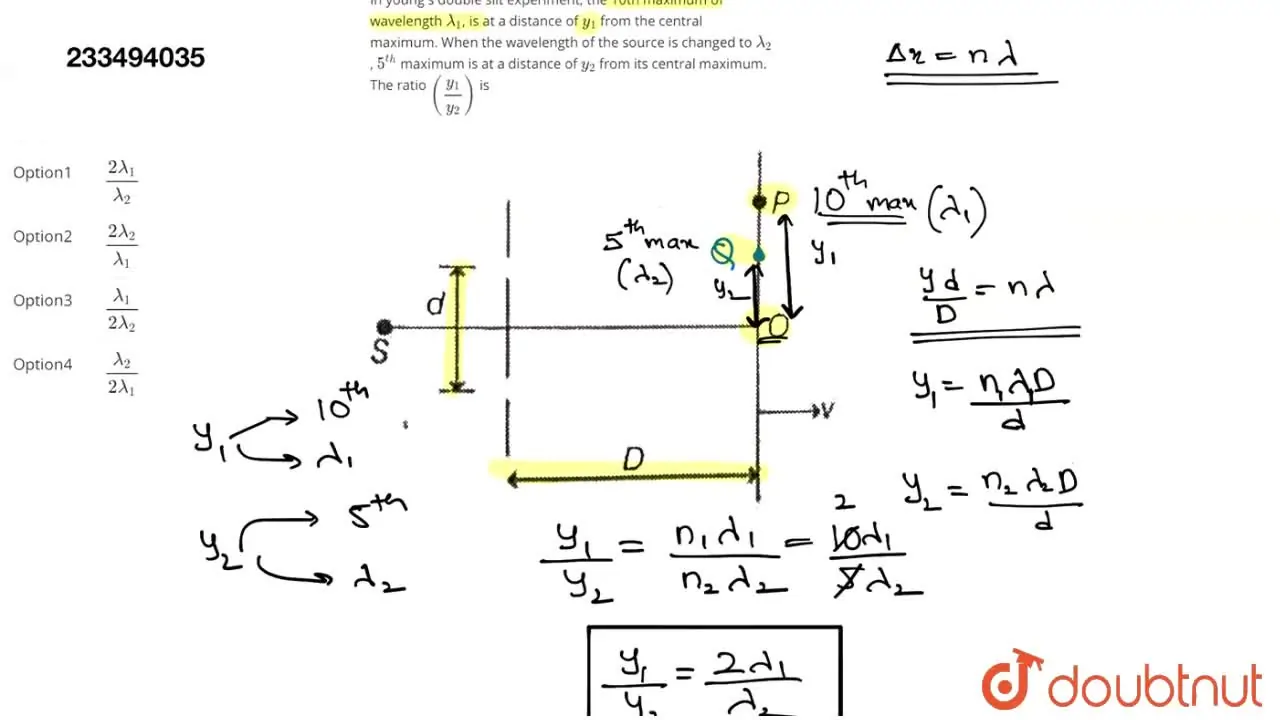
In young's double slit experiment, the 10th maximum of wavelength λ1, is at a distance of y1 from the central maximum. When the wavelength of the source is changed to λ2, 5th maximum is at a distance of y2 from its central maximum. The ratio (y1y2) isSolution in Telugu
2λ1λ2
2λ2λ1
λ12λ2
λ22λ1
The correct Answer is:a
The condition to form a bright band at a point is given by,y=nλDd
For y1:Y1=nλ1Dd(∵n=10)
⇒y1=10λ1Dd
For y2:Y2=nλ2Dd(∵n=5)
⇒y2=5λ2Dd
⇒y1y2=(10λ1d)5λ2Dd
∴y1y2=2λ1λ2
Related Playlists
Similar Questions
A tanserve wave is repsemted by the equation y=2sin(30t−40x) and the measerments of distances are in meters, then the velocity of propagation is
02:56View SolutionA man of weight 50 kg carries an object to a height of 20m in a time of 10 sec. The power used by the man in this process is 2000W, then find the weight of the object carried by the man [assume g=10ms−2]
02:45View SolutionIn Young's double slit experiment, the 8th maximum with wavelength λ1 is at a distance d1 from the central maximum and the 6th maximum with a wavelength λ2 is at a distance d2. Then (d1/d2) is equal to
View SolutionIn Young's double slit experiment, the 10th maximum of wavelength lambda_(1) is at distance of y_(1) from the central maximum. When the wavelength of the source is changed to lambda_(2) , 5th maximum is at a distance of y_(2) from its central masximum. Then (y_(1))/(y_(2)) is
02:07View SolutionIn Young’s double slit experiment, the 8 th maximum with wavelength lambda_(1) is at a distance d_(1) from the central maximum and the 6 th maximum with a wavelength lambda_(2) is at a distance d_(2) . Then (d_(1)//d_(2)) is equal to
03:21View SolutionIn Young's double slit experiment, the 10th maximum of wavelength λ1 is at a distance y1 from its central maximum and the 5th maximum of wavelength λ2 is at a distance y2 from its central maximum. The ratio y1/y2 will be
05:34View SolutionIn Young's double slit experiment, wavelength λ=5000Å the distance between, the slits is 0.2mm and the screen is at 200cm from the slits. The central maximum is at x=0 The third maximum (Taking the central maximum as zeroth maximum) will be at x equal to
01:57View Solutionसिद्ध कीजिए की बिन्दुओं (x1,y1) और (x2,y2) से होकर जाने वाली रेखा की मुलबिन्दु से दुरी ∣∣ ∣ ∣∣x1y2−x2y1√(x2−x1)2+(y2−y1)2∣∣ ∣ ∣∣ है।
05:58View SolutionIn Fresnel's biprism experiment, the 5th maximum for wavelength lamda_(1) is at a distance d_(1) from the central bright bannd. If the 5th maximum for wavelength lamda_(2) is at a distance d_(2) from the central bright band, then the ratio d_(1)/d_(2) is
View SolutionIn Young's double slit experiment the 7th maximum with wavelength lambda_(1) is at a distance d_(1) , and that with wavelength lambda_(2) is at distance d_(2) . Then d_(1)//d_(2) is
03:02View SolutionIn Young's double slit experiment, the 10th maximum of wavelength lambda_(1) is at a distance y_(1) from its central maximum and the 5th maximum of wavelength lambda_(2) is at a distance y_(2) from its central maximum. The ratio y_(1) // y_(2) will be:
03:02View SolutionIn young's double slit experiment, the 10th maximum of wavelength λ1, is at a distance of y1 from the central maximum. When the wavelength of the source is changed to λ2, 5th maximum is at a distance of y2 from its central maximum. The ratio (y1y2) is
03:02View SolutionIn young's double slit experiment, the 10th maximum of wavelength λ1, is at a distance of y1 from the central maximum. When the wavelength of the source is changed to λ2, 5th maximum is at a distance of y2 from its central maximum. The ratio (y1y2) is
02:34View SolutionIn young's double slit experiment, the 10th maximum of wavelength λ1, is at a distance of y1 from the central maximum. When the wavelength of the source is changed to λ2, 5th maximum is at a distance of y2 from its central maximum. The ratio (y1y2) is
03:20View SolutionIn Young's double slit experiment, the 10th maximum of wavelength λ1 is at a distance y1 from its central maximum and the 5th maximum of wavelength λ2 is at a distance y2 from its central maximum. The ratio y1/y2 will be
01:32View Solution