The air column in a pipe closed at one end is made to vibrate in its second overtone by a tuning fork of frequency 440Hz. The speed of sound in air is 330ms−1. End corrections may be neglected. Let P0 denote the mean pressure at any point in the pipe, and ΔP the maximum amplitude of pressure variation.
(a) What the length L of the air column.
(b) What is the amplitude of pressure variation at the middle of the column?
( c ) What are the maximum and minimum pressures at the open end of the pipe?
(d) What are the maximum and minimum pressures at the closed end of the pipe?
A-R,B-S,C-P,D-S
A-R,B-P,C-P,D-S
A-R,B-P,C-Q,D-Q
A-R,B-P,C-Q,D-S
The correct Answer is:C
A. The fundamental frequency of the closed organ pipe=V/4l. In closed organ pipe only odd harmonics are present.
Second overtone of pipe=5v/4L
Given 5v/4L=440
On solving, we get L=5v4×440=5×3304×440=1516m=0.9375m=93.75cm
B. The equation of variation of presure amplitude at any distance x from the node is
ΔP=ΔP0coskx
Pressure variation is maximum at a node and minimum (zero) at antinode
Distance of centre C from N2 is λ8
ΔP=ΔP0cos2πλ×λ8=ΔP0π4=ΔP0√2
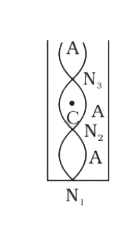
C & D : At antinode, the pressure variation is minimum (zero), therefore at antinode pressure remains equal to
P0 (Always).
Therefore, at antinode Pmax=Pmin=P0.
Similar Questions
The air column in a pipe closed at one end is made to vibrate in its second overtone by a tuning fork of frequency 440Hz. The speed of sound in air is 330ms−1. End corrections may be naglected. Let P0 denote the mean pressure at any point in the pipe, and ΔP the maximum amplitude of pressure variation.
(a) Find the length L of the air column.
(b) What is the amplitude of pressure variation at the middle of the column?
( c ) What are the maximum and minimum pressures at the open end of the pipe?
(d) What are the maximum and minimum pressures at the closed end of the pipe?
View SolutionThe air in a pipe closed at one end is made to vibrate in its second overtone by a tuning fork of frequency 440Hz. The speed of sound in a 330m/s. End correction may be neglected . Let P0 denotes the mean pressure of any point in the pipe and ΔP0 the maximum amplitudes of pressure variation.
a. Find the length L of the air column.
b. What is the amplitude of pressure variation at the middle of the column
c. What are maximum and minimum pressures at the open end of the pipe ?
View Solution
Knowledge Check
A resonance air column of length 20 cm resonates with a tuning fork of frequency 250 Hz . The speed of sound in air is
A300m/sB200m/sC150m/sD75m/sAn air column in a pipe, which is closed at one end will be in resonance with a vibrating tunning fork of frequency 264 Hz, If the length of column is :-
A37.25B62.50C93.75D125An air column in a pipe which is closed at one end will be in resonance with a vibrating tuning fork of frequency 264 Hz if the length of the air column in cm is (Speed of sound in air = 340 m/s)
A31.25B62.50C93.75D125
The air column in a pipe closed at one end is made to vibrate in its second overtone by a tuning fork of frequency 440 Hz. The speed of sound in air is 330 m/s. find the length of air column.
View Solution(i) Two cylindrical pipes are each of length L=30cm. One of them contains hydrogen and the other has oxygen at the same temperature. The ends A, B, C and D of the pipes are fitted with flexible diaphragms. The diaphragms A and C are set into oscillations simultaneously using the same source having frequency f=600Hz. Calculate the difference in phase of oscillations of the diaphragms D and B if it is known that the speed of sound in hydrogen at the temperature concerned is 1200m/s.

(ii) The air column in a pipe closed at one end is made to vibrate in its second overtone by a tuning fork of frequency 440Hz. The speed of sound in air is 330m/s. P0 is mean pressure in the pipe and ΔP0 is maximum amplitude of pressure variation. Neglect end correction.
(a) Find the length L of air column.
(b) What is amplitude of pressure variation at the middle of the column?
(c) What is maximum and minimum pressure at the closed end?
View SolutionAn air columbn in pipe, which is closed at one end, will be in resonance with a vibrating tuning fork of frequency 264Hz if the length of the column in cm is :
View SolutionThe air in a pipe closed at one end is in resonance with a vibrating tuning fork of frequency 170 Hz. If the velocity of sound in air is 340 m/s, the length of the vibrating air column is
View SolutionIf the air column in a pipe which is closed at one end, is in resonance with a vibrating tuning fork at a frequency 260 Hz, then the length of the air column is
View Solution