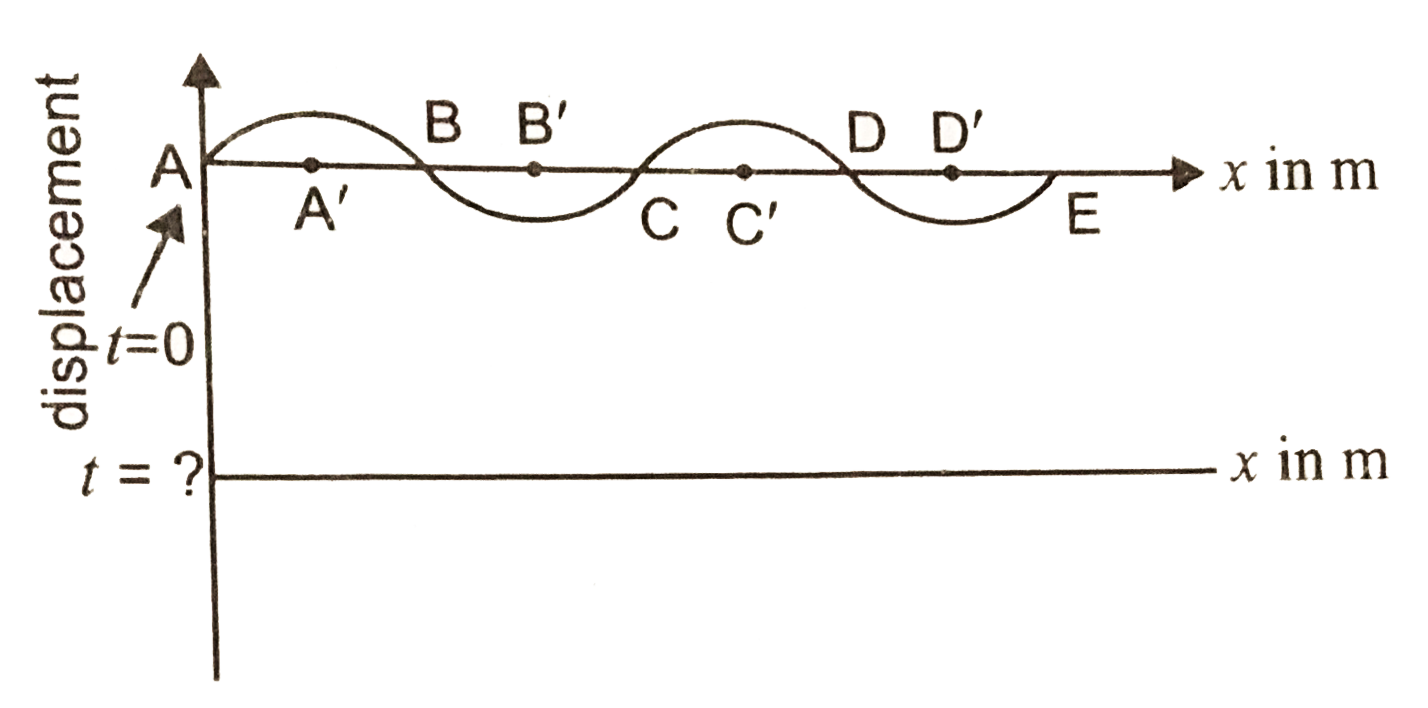
The pattern of standing waves formed on a stretched string at two in -stants of time are shown of time are shown in figure. The velocity of two waves superim-posing to form stationary waves is 360ms−1 and their frequencies are 256 Hz.

(a) Calculate the time at which the second curve is plotted.
(b) Mark nodes and antinodes on the curve.
(c) Calculate the distance between A' and C'
(i) Here, v=360ms−1,v=256Hz
λ=vu=360256=1.406m
AA'=λ4=1,4064=0.3516m
Time (t) at which the second curve is plotted AA'v=0.3516360=9.8×10−4s
(ii) Nodes A,B,C,D,E, antinodes A',C'
(iii) Distance between A' and C', λ=1.406m=1.41m.
Similar Questions
The pattern of standing waves formed on a stretched strinig at two instants of time are shown in figure. The velocity of two waves superimposing to form stationary wave is 360ms−1 and their frequencies are 256 Hz.
(a) Calculate the time at which the second curve is plotted. ltbr> (b) Mark nodes and antinodes on the curve.
(c) Calculate the distance between A′ and C′.
View SolutionA light wave is reflected from a mirror. The incident and reflected waves superimpose to form stationary waves. But no nodes and antinodes are seen. Why?
View Solution
Knowledge Check
At nodes, the velocity of stationary wave is
Azero.Bmaximum.Cminimum but not zero.Ddepends on amplitude.Disturbances of two waves are shown as a function of time in the following figure. The ratio of their intensities will be -
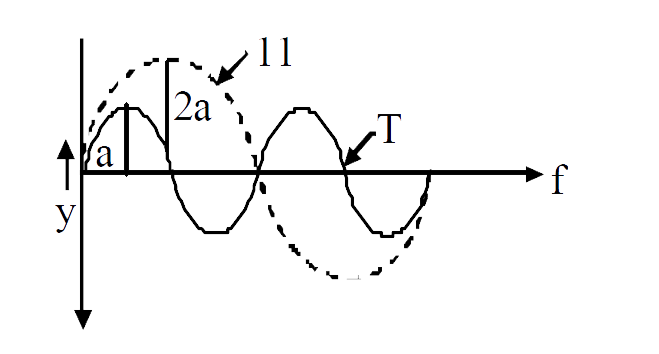 A1:4B4:1C1:2D1:1
A1:4B4:1C1:2D1:1
Stationary waves
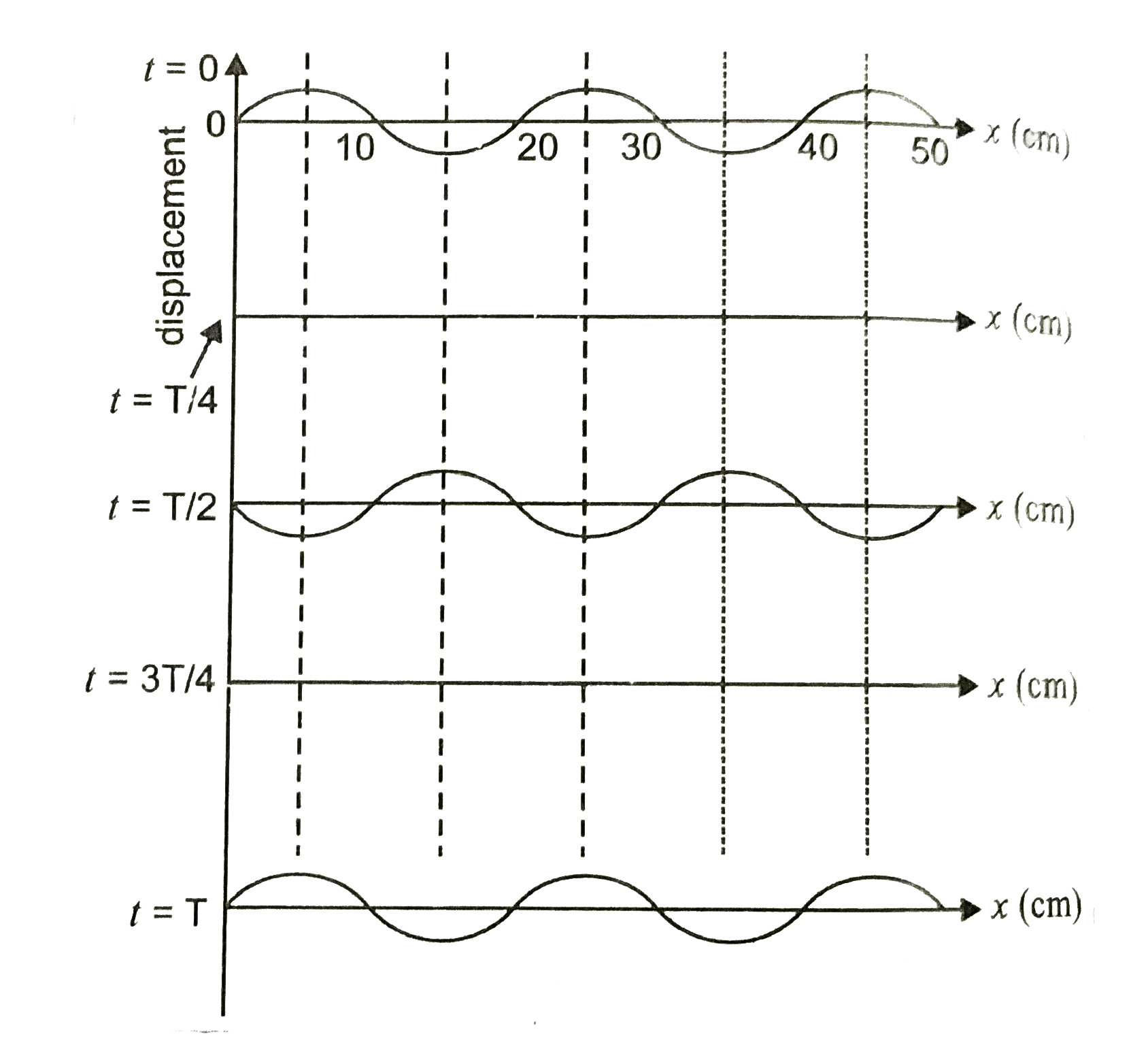
View SolutionThe wave pattern on a stretched string is shown in figure . Interpret what kind of wave this is and find its wavelength
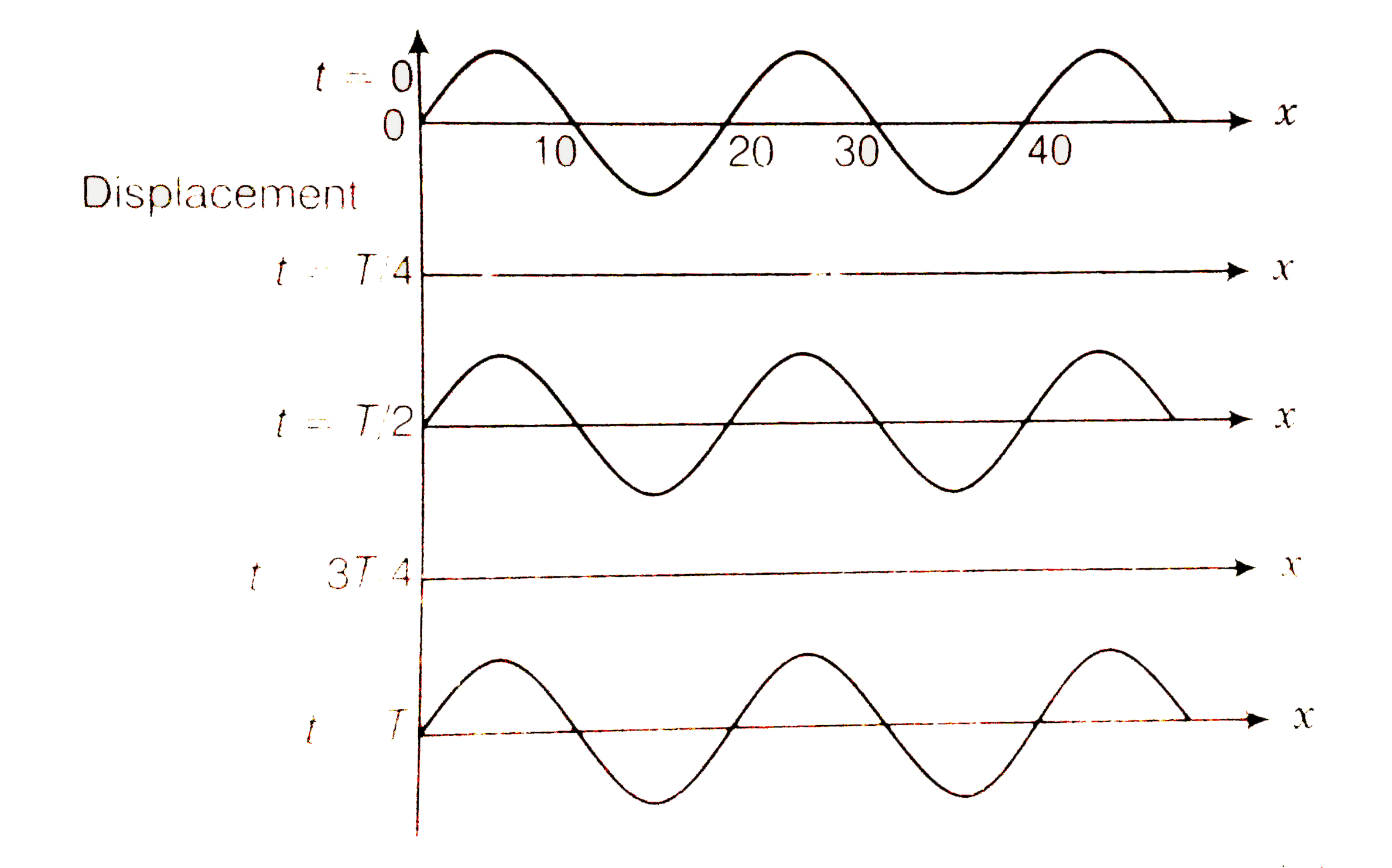
View SolutionThe wave pattern on a stretched string is shown in figure. Interpret what kind of wave this is and find its wavelength.
View SolutionDisturbances of two waves are shown as a function of time in the following figure. The ratio of their intensities will be -
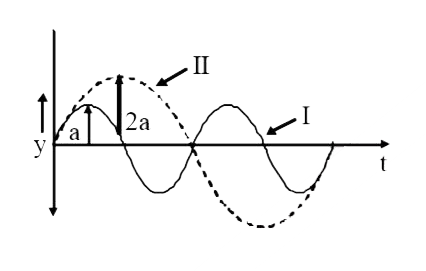
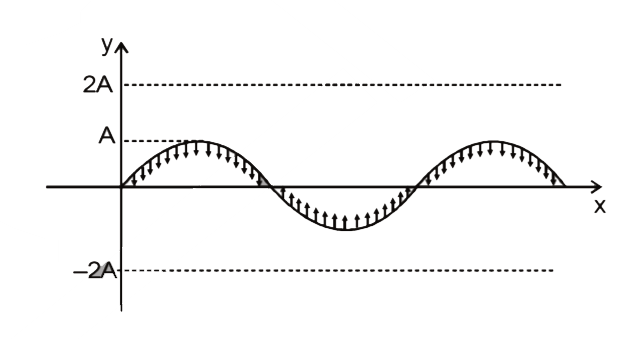
View SolutionFigure shows the standing waves pattern in a string at t = 0. Find out the equation of the standing wave where the amplitude of antinode is 2A.
View SolutionSpeed of a sound wave in water is 1600ms−1 and its frequency is 4000 Hz. Find time period of the wave.
View Solution
