A particle is moving in a circel of radius R=1m with constant speed v=4m/s. The ratio of the displacement to acceleration of the foot of the perpendicular drawn from the particle onto the diameter of the circel is
1/16(second)2
1/2(second)2
2(second)2
16(second)2
The correct Answer is:A
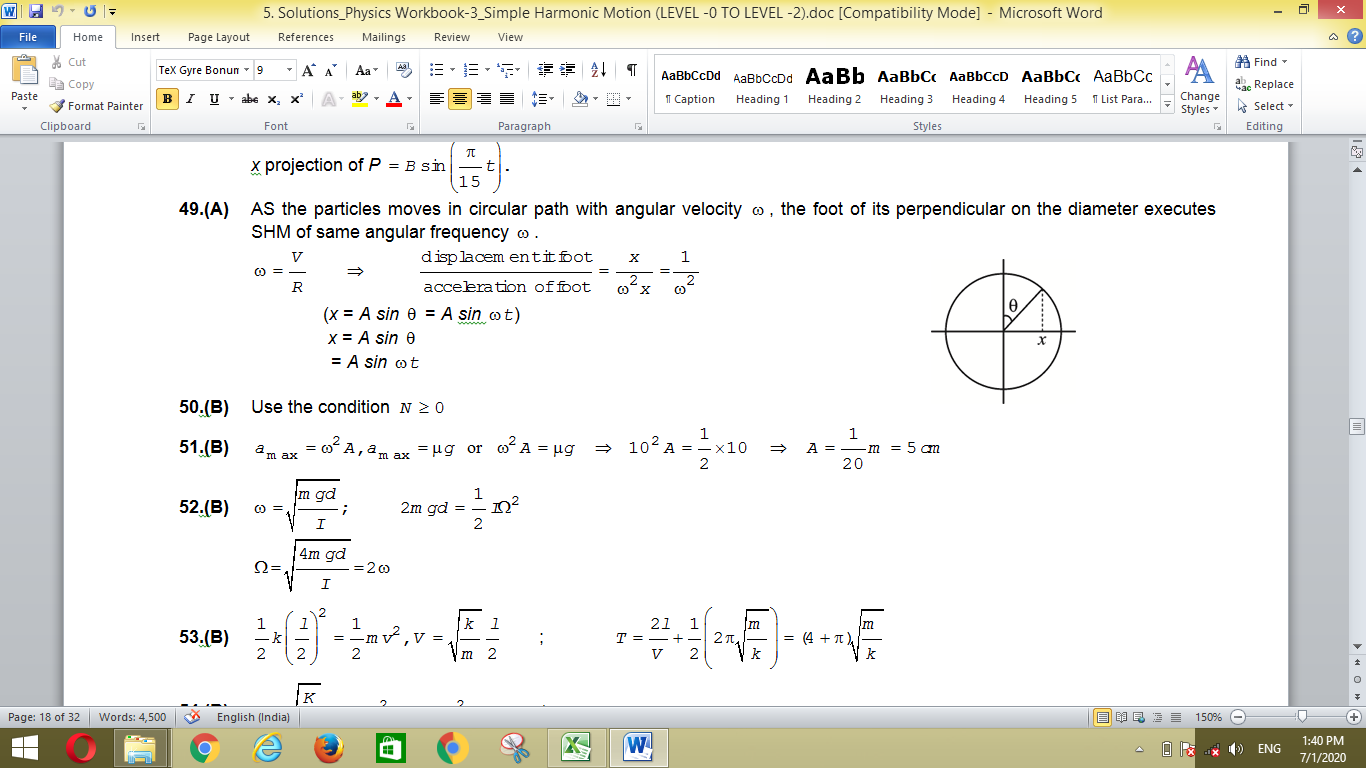
As the particles moves in circular path with angular velocity ω, the foot of its perpendicular on the dimeter executes SHM of same angular frequency ω.
ω=VR⇒displacement it footacceleration of foot=xω2x=(1)(ω2)
(x=Asinθ=Asinωt)
x=Asinθ)
Asinωt
Similar Questions
A particle is revolving in a circular path of radius 2 m with constant angular speed 4 rad/s. The angular acceleration of particle is
View SolutionA particle is revolving in a circular path of radius 25m with constant angular speed 12 rev/min.Then the angular acceleration of particle is
View Solution
Knowledge Check
If a particle is moving along a circle of radius 3 m with a constant speed 9m/s, then it covers a quarter of the circle in time of
Aπ/3sBπ/6sCπ/2sDπ/8sA particle is moving on a circular path of radius R with constant speed v. During motion of the particle form point A to point B
 AAverage speed is v/2BThe magnitude of average velocity is v/πCThe magnitude of average acceleration is 2v2πRDAverage velocity is zero.
AAverage speed is v/2BThe magnitude of average velocity is v/πCThe magnitude of average acceleration is 2v2πRDAverage velocity is zero.A particle is revoiving in a circular path of radius 25 m with constant angular speed 12 rev/min. then the angular acceleration of particle is
A2π2 rad/s2B4π2rad/s2Cπ2 rad/s2Dzero
A particle is revolving in a circular path of radius 25m with constant angular speed 12 rev/min.Then the angular acceleration of the particle is?
View SolutionA particle moves in a circle of radius 4m with a linear speed of 20m/s. Find the angular speed.
View SolutionA particle is moving in a circle of radius 1 m with speed varying with time as v=(2t)m/s. In first 2 s
View SolutionA particle is moving in a circle of radius 1 m with speed varying with time as v = (2t) m/s. In first 2 sec:
View SolutionA Particle of mass 'M' moves in a uniform circular path of radius 'r' with a constant speed 'v' then its centripetal acceleration is .
View Solution