A spring of spring constant k is cut into n equal parts, out of which r parts are placed in parallel and connected with mass M as shown in figure. The time period of oscillatory motion of mass M is:
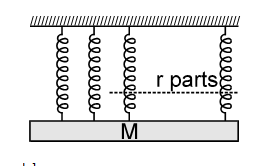
T=2π√MrnK
T=2π√nrMK
T=2π√nMnK
T=2π√nMrK
The correct Answer is:A
K.I=k'×In constant
new spring constand for each part is k'=nK
Now r part are taken in parallel.
so Keq=nrk, Time period =2π√MKeq=2π√MnrK
Similar Questions
A spring is of spring constant k=15N/cm. It is cut into 3 equal parts, which are joined in parallel. What is the spring constant of the combination ?
View SolutionA spring of force constant 20 N/m is cut into four equal parts, All the four parts are now connected in parallel. Find the force constant of the combination.
View Solution
Knowledge Check
A spring of spring constant K is cut equal parts of which t part are places in particle and connected will mass m shown in figure The time period of oscilation motion of mass m is
AT=2π√mnrkBT=2π√nrmKCT=2π√rmnKDT=2π√nmrKA spring of spring constant k is cut in three equal pieces. The spring constant of each part will be
Ak3B3kCkDk6A spring of force constant k is cut into three equal parts. The force constant of each part would be
Ak/3B3kCkD2k
A spring of force constant k is cut into four equal parts. The force constant of each part will be
View SolutionA spring of force constant k is cut into there equal part what is force constant of each part ?
View SolutionA spring (spring constant=k) is cuttend into 4 equal parts and two parts are connected in parallel. What is the effective spring constant?
View SolutionTwo springs of force constant k and 2k are connected to a mass shown in figure the frequency of oscillation of the mass is

View SolutionTwo springs of force constant k and 2k are connected to a mass shown in figure the frequency of oscillation of the mass is

View Solution
