The position of a particle executing SHM is given by A sin(wt) Write the expression for the velocity of the particle.
Since, x=sinωt, velocity of the particle is given by v=dxdt=ddt(Asinωt)=Aωcosωt.
Similar Questions
Knowledge Check
The acceleration of a particle executing S.H.M. is
AAlways directed towards the equilibrium positionBAlways towards the one endCContinuously changing in directionDMaximum at the mean positionIf the displacement of a particle executing S.H.M. is given by x = 0.24 sin (400 t + 0.5)m, then the maximum velocity of the particle is
A24m/sB48m/sC96m/sD72m/sThe potential energy of a particle (Ux) executing SHM is given by
AUx=K2(x−x0)2BUx=K1x+K2x2+K3x3CUx=Ae−bxDUx= constant
The kinetic energy of a particle executing S.H.M. is 16 J when it is at its mean position. If the mass of the particle is 0.32 kg , then what is the maximum velocity of the particle
View SolutionThe displacement of a particle executing SHM is given by
Y=5 sin (4t+π3)
If T is the time period and the mass of the particle is 2g, the kinetic energy of the particle When t=T4 is given by-
View SolutionThe displacement of a particle executing SHM is given by y=0.5 sint cm . The maximum speed of the particle is
View Solution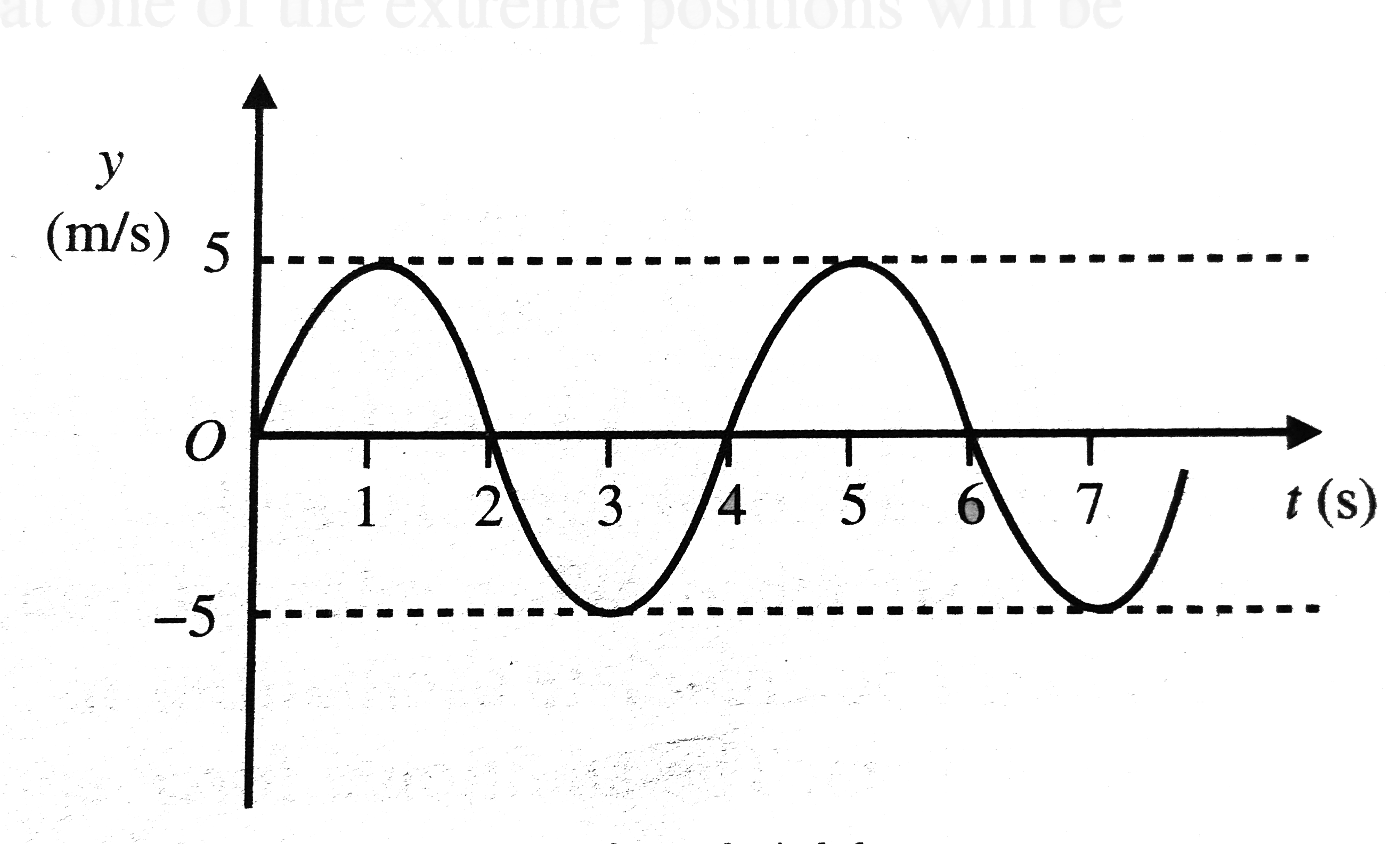
The variation of velocity of a particle executing SHM with time is shown is fig. The velocity of the particle when a phase change of π6 takes place from the instant it is at one of the extreme positions will be
View SolutionThe instantaneous acceleration of a particle executing SHM given by y=asinωt is
View Solution