Ideal mono-atomic gas is taken through process such that dQ = 3dU. The molar heat capacity for process is:
3R
4.5R
4R
2R
The correct Answer is:B
To find the molar heat capacity for the process where dQ=3dU for an ideal monoatomic gas, we can follow these steps:
Step 1: Understand the relationship between heat, internal energy, and temperature change.
The molar heat capacity C is defined as:
C=dQdT
where dQ is the heat added to the system and dT is the change in temperature.
Step 2: Use the given relationship dQ=3dU.
From the problem, we know:
dQ=3dU
This implies that the heat added to the system is three times the change in internal energy.
Step 3: Relate internal energy change to temperature change.
For an ideal monoatomic gas, the change in internal energy dU is given by:
dU=f2nRdT
where f is the degrees of freedom. For a monoatomic gas, f=3. Therefore:
dU=32nRdT
Step 4: Substitute dU into the equation for dQ.
Substituting dU into the equation for dQ:
dQ=3dU=3(32nRdT)=92nRdT
Step 5: Substitute dQ into the molar heat capacity equation.
Now, substituting dQ into the molar heat capacity equation:
C=dQdT=92nRdTdT=92nR
Since we are considering one mole of gas (n=1):
C=92R
Step 6: Simplify the result.
Thus, the molar heat capacity for the process is:
C=4.5R
Final Answer:
The molar heat capacity for the process is 92R or 4.5R.
---
The molar heat capacity C is defined as:
C=dQdT
where dQ is the heat added to the system and dT is the change in temperature.
From the problem, we know:
dQ=3dU
This implies that the heat added to the system is three times the change in internal energy.
For an ideal monoatomic gas, the change in internal energy dU is given by:
dU=f2nRdT
where f is the degrees of freedom. For a monoatomic gas, f=3. Therefore:
dU=32nRdT
Substituting dU into the equation for dQ:
dQ=3dU=3(32nRdT)=92nRdT
Now, substituting dQ into the molar heat capacity equation:
C=dQdT=92nRdTdT=92nR
Since we are considering one mole of gas (n=1):
C=92R
Thus, the molar heat capacity for the process is:
C=4.5R
The molar heat capacity for the process is 92R or 4.5R.
Similar Questions
Knowledge Check
Ideal gas is taken through process shown in figure
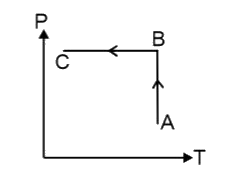 Aln process AB, work done by system is positiveBIn process AB, heat is rejected out of the system.CIn process AB, internal energy increasesDIn process AB internal energy decreases and
Aln process AB, work done by system is positiveBIn process AB, heat is rejected out of the system.CIn process AB, internal energy increasesDIn process AB internal energy decreases and
in process BC internal energy increasesThe molar heat capacity for an ideal gas cannot
Abe negativeBbe equal to either CV or CPClie in the range CV≤C≤CPDit may have any value between −∞ and +∞The molar heat capacity of a gas in a process
Ais either CVorCPBlies between CVorCPCmay range from zero to infinityDcan have any real value
Ideal gas is taken through a process as shown in figure
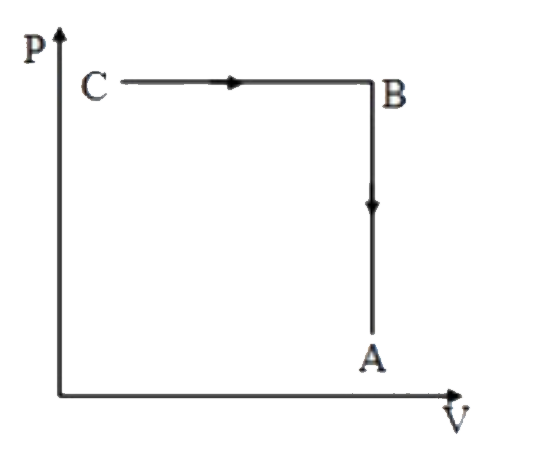
View SolutionIdeal gas is taken through process shown in figure :
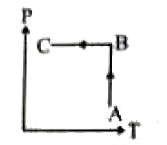
View SolutionIdeal gas is taken through the process shown in the figure :
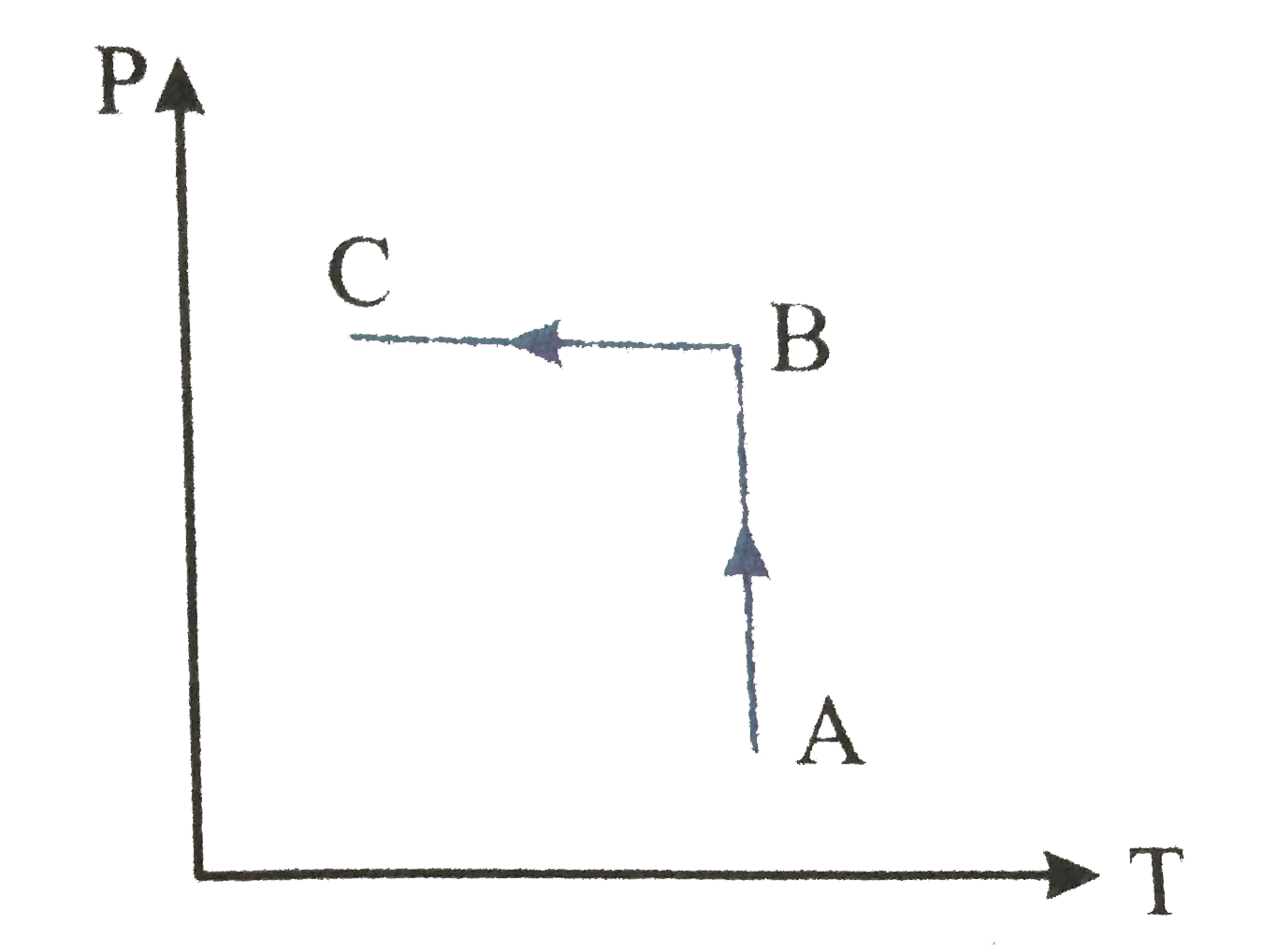
View SolutionThe molar heat capacity for the process shown in fig. is

View SolutionThe molar heat capacity for the process shown in fig. is
View Solution