Three particles, each of the mass m are situated at the vertices of an equilateral triangle of side a. The only forces acting on the particles are their mutual gravitational forces. It is desired that each particle moves in a circle while maintaining the original mutual separation a. Find the initial velocity that should be given to each particle and also the time period of the circular motion. (F=Gm1m2r2)
The correct Answer is:(i)1(ii)
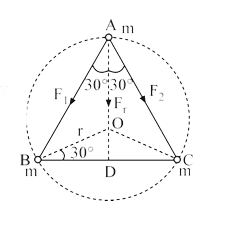
In the figure three particles located at vertices A, B and C of equilateral triangle of side AB = BC = CA = a.
These particle move in a circle with O as the centre and radius
r=OA=OB=OC where
r=BDcos30∘=a/2√3/2=a√3
The gravitational attraction force acting on a particle, say due to particle at B is F1=Gmma2 and due to particle at C is F2 .
Here F1=F2=F (Say) =Gm2a2
Therefore, the resultant force on the particles at A is
=2Fcos30∘=2Gm2a2√32=√3Gm2a2
Fr is directed along AO..... Thus the net force on particle at A is radial. Similarly, the net force on particle at B and C at C is Fr, each directed towards centre O. This force provides the necessary centripetal force. If v is the required initial velocity of each particle , then mv2r=√3Gm2a2 or v2=√3Gmra2
Since r=a√3, we have v2=√3(Gm)(√3a)=Gma⇒v=√Gma
Time period T = 2πrv=2π×a/√3√Gma=2π(a33Gm)1/2
Similar Questions
Three parties, each of mass m, are situated at the vertices of an equilateral triangle of side length a . The only forces acting on the pariclaes are th eir mutual gravitational forces. It is desired that each particles moves ina a circle while maintaining the original mutual separation a. Find the initial velocity that should be given to each particle and also the time period of teh circular motion.
View SolutionThree particles, each of mass m, are situated at the situated at the vertices of an equilateral triangle of side 'a'. The only forces acting on the particles are their mutual gravitational forces. It is intended that each particle moves along a circle while maintaining their original separation 'a'. Determine the initial velocity that should be given to each particle and the time period of the circular motion. The resultant force on particle at A due to other two particles is
View Solution
Knowledge Check
Three particles each of mass m are kept at vertices of an equilateral triangle of side L. The gravitational field at centre due to these particle is
AzeroB3GML2C9GML2D12GM√3L2Three particles each of mass m are kept at the vertices of an euilateral triangle of side L. The gravitational field at the centre due to these particle is
AzeroB3GML2C9GML2D2GML2Three particles of equal mass 'm' are situated at the vertices of an equilateral triangle of side L. The work done in increasing the side of the triangle to 2L is
A2G2m2LBGm22LC3Gm22LD3Gm2L
Three particles, each of mass m, are situated at the vertices of equilateral triangle of side length a. The only forces. It is desired that each particle moves in a circle while maintaining the original mutual speration a. Find the intial velocity that should be given to each particle and also the time period of the circular motion.
View SolutionThree particles, each of mass m are fixed at the vertices of an equilateral triangle of side length a. The only forces acting on the particles are their mutual gravitational forces. Then answer the following questions.
The gravitational potential at O is
View SolutionThree particles, each of mass m are fixed at the vertices of an equilateral triangle of side length a. The only forces acting on the particles are their mutual gravitational forces. Then answer the following questions.
Force acting on particle C, due to particle A and B
View SolutionThree particles each of mass m are palced at the corners of an equilateral triangle of side b. The gravitational potential energy of the system of particle is
View SolutionThree particles each of mass m are kept at the vertices of an euilateral triangle of side L. The gravitational field at the centre due to these particle is
View Solution
