_E01_134_Q01.png)
Figure elliptical path abcd of a planet around the sun S such that the area of Delta csa is 14 the area of the ellipse, (see figure) with db as the semimajor axis, and ca as the semiminor axis if t1 is the time taken for planet to go over path abc and t2 for path taken over cda then:
t1=t2
t1=2t2
t1=3t2
t1
The correct Answer is:C
Let area of ellipse = A & areal velocity = VA
then , t1=A/2+A/4VA=(34A)×1VA and t2=A/2−A/4V4=(14A)×1VA⇒t1=3t2
Similar Questions
Figure shows the elipticla path of a planet about the sun. The two shaded parts have equal area. If t1andt2 be the tim taken by the planet to go from a to b and from c to d respectively
View Solution_S01_025_Q01.png)
The figure shows elliptical orbit of a planet m about the sun S. the shaded area SCD is twice the shaded area SAB. If t1 be the time for the planet to move from C to D and t2 is the time to move from A to B, then:
View Solution
Knowledge Check
Figure shows the orbit of a planet P round the sun S. AB and CD are the minor and major axed of the ellipse.
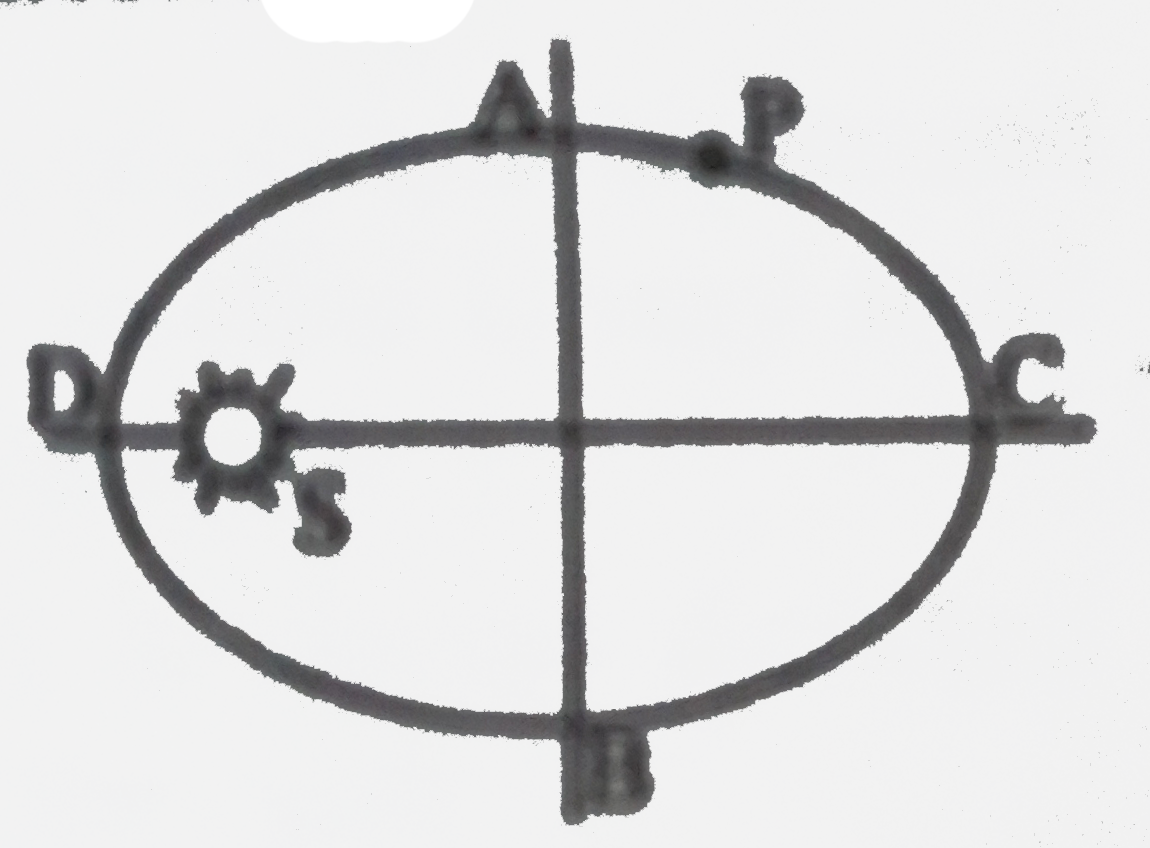
If t1 is the time taken by the planet to travel along ACB and t2 the time along BDA, thenAt1=t2Bt1>>t2Ct1<t2Dnothing can be concludedFigure shows the motion of a planet around the Sun S in an elliptical orbit with the Sun at the focus. The shaded areas A and B are also shown in the figure which can be assumed to be equal. If t1 and t2 represent the time taken for the planet to move from a to b and c to d, respectively then
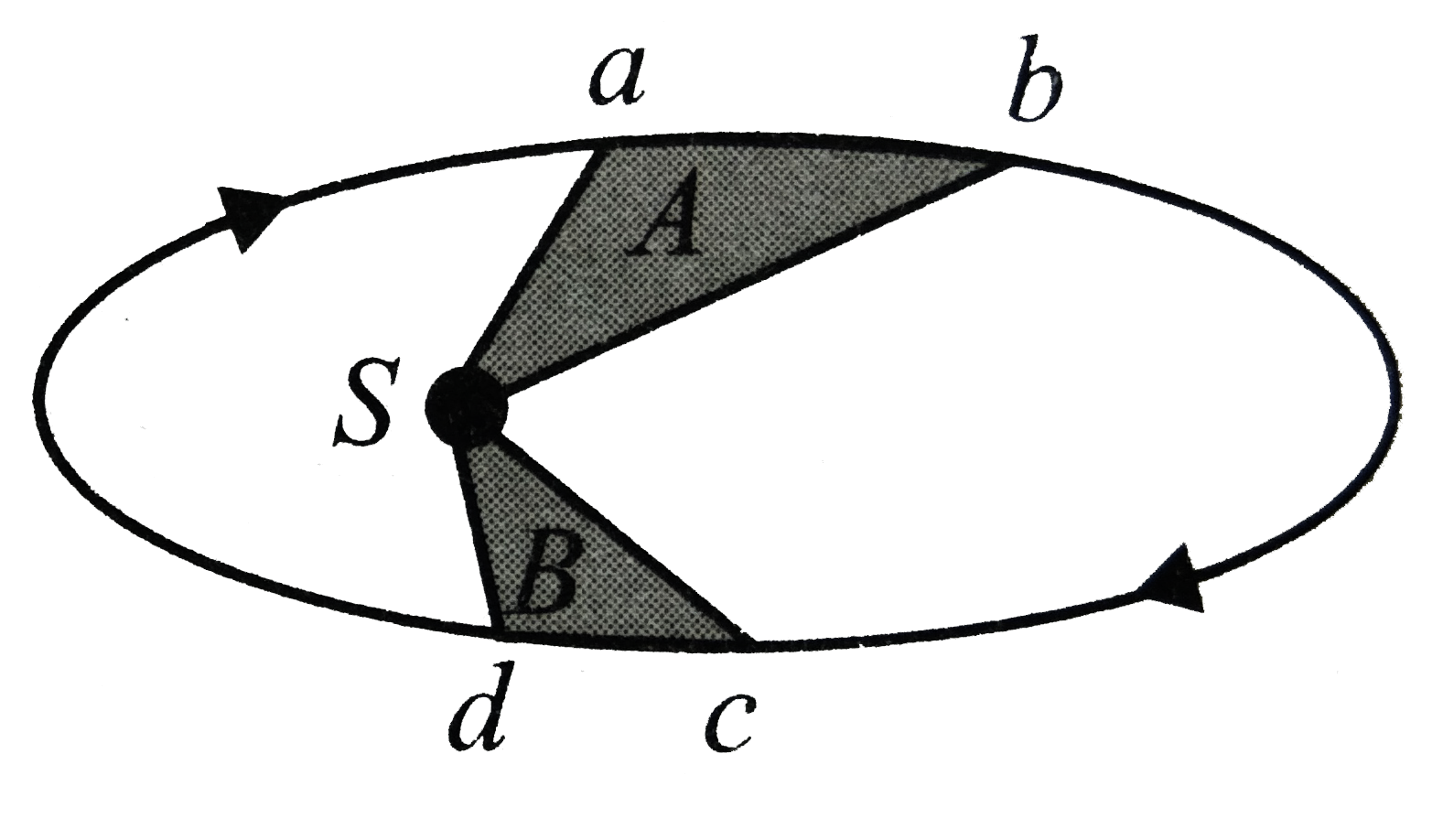 At1<t2Bt1>t2Ct1=t2Dfrom the given information the relation between t1 and t2 cannot be determined
At1<t2Bt1>t2Ct1=t2Dfrom the given information the relation between t1 and t2 cannot be determined_S01_025_Q01.png)
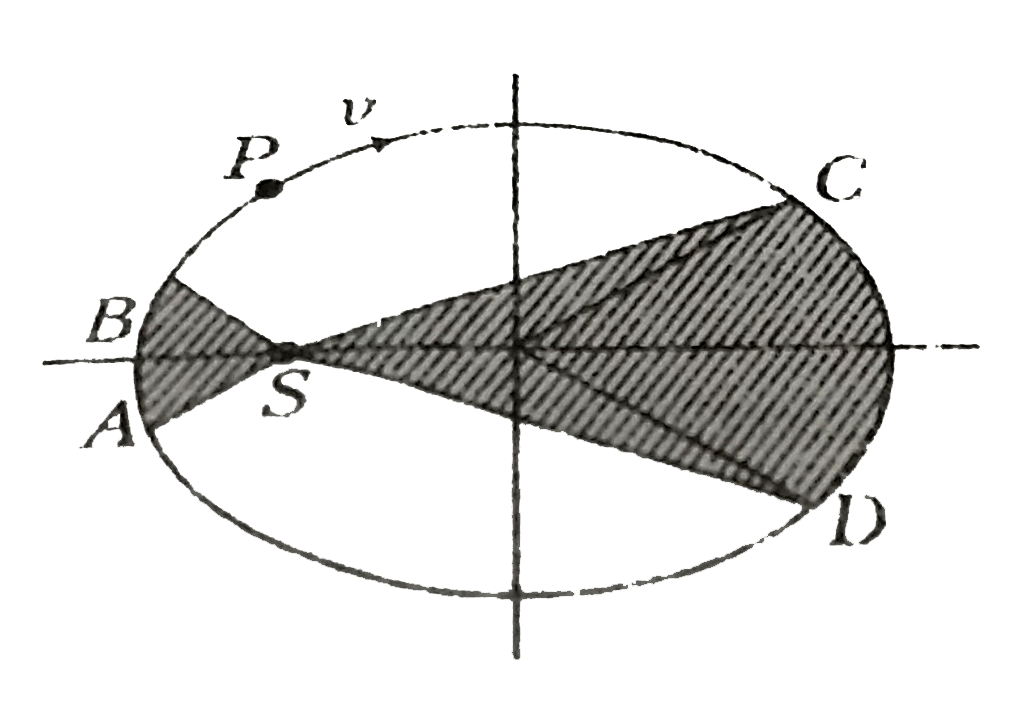
The figure shows elliptical orbit of a planet m about the sun S. the shaded area SCD is twice the shaded area SAB. If t1 be the time for the planet to move from C to D and t2 is the time to move from A to B, then:At1=t2Bt1=8t2Ct1=4t2Dt1=2t2
The figure shows elliptical orbit of a plant P about the sun S. The shaded area CSD is twice tha shaded area ASB. If t1 is the time taken by the planet to move from C to D and t2 is the time to move from A to B, determine the ratio t1/t2
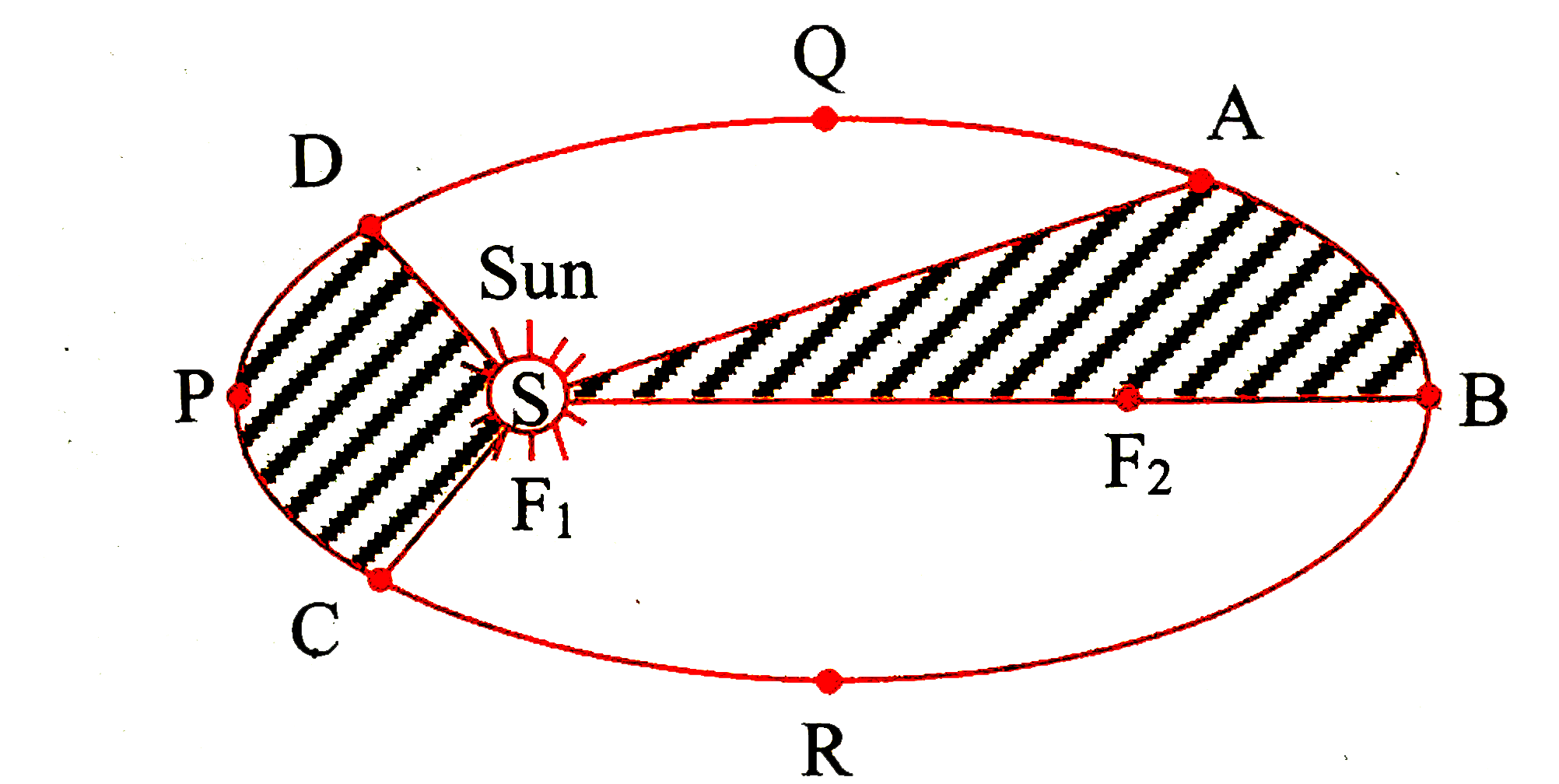
View SolutionThe figure shows the elliptical orbit of a planet about the sun S. An ellipse is the curve obtained when a cone is cut by an inclined plane. It has two focal points. The sum of the distances to the two focal points from every point on the curve is constant. F1 and F2 are the two focal points of the ellipse . The shaded area CF1D is twice the shaded area AF1B.t1 is the time taken by the planet to move from C to D and t2 is the time to move from A to B
State the law regarding areas swept by the line joining the planet and the sun
View SolutionThe figure shows the elliptical orbit of a planet about the sun S. An ellipse is the curve obtained when a cone is cut by an inclined plane. It has two focal points. The sum of the distances to the two focal points from every point on the curve is constant. F1 and F2 are the two focal points of the ellipse . The shaded area CF1D is twice the shaded area AF1B.t1 is the time taken by the planet to move from C to D and t2 is the time to move from A to B
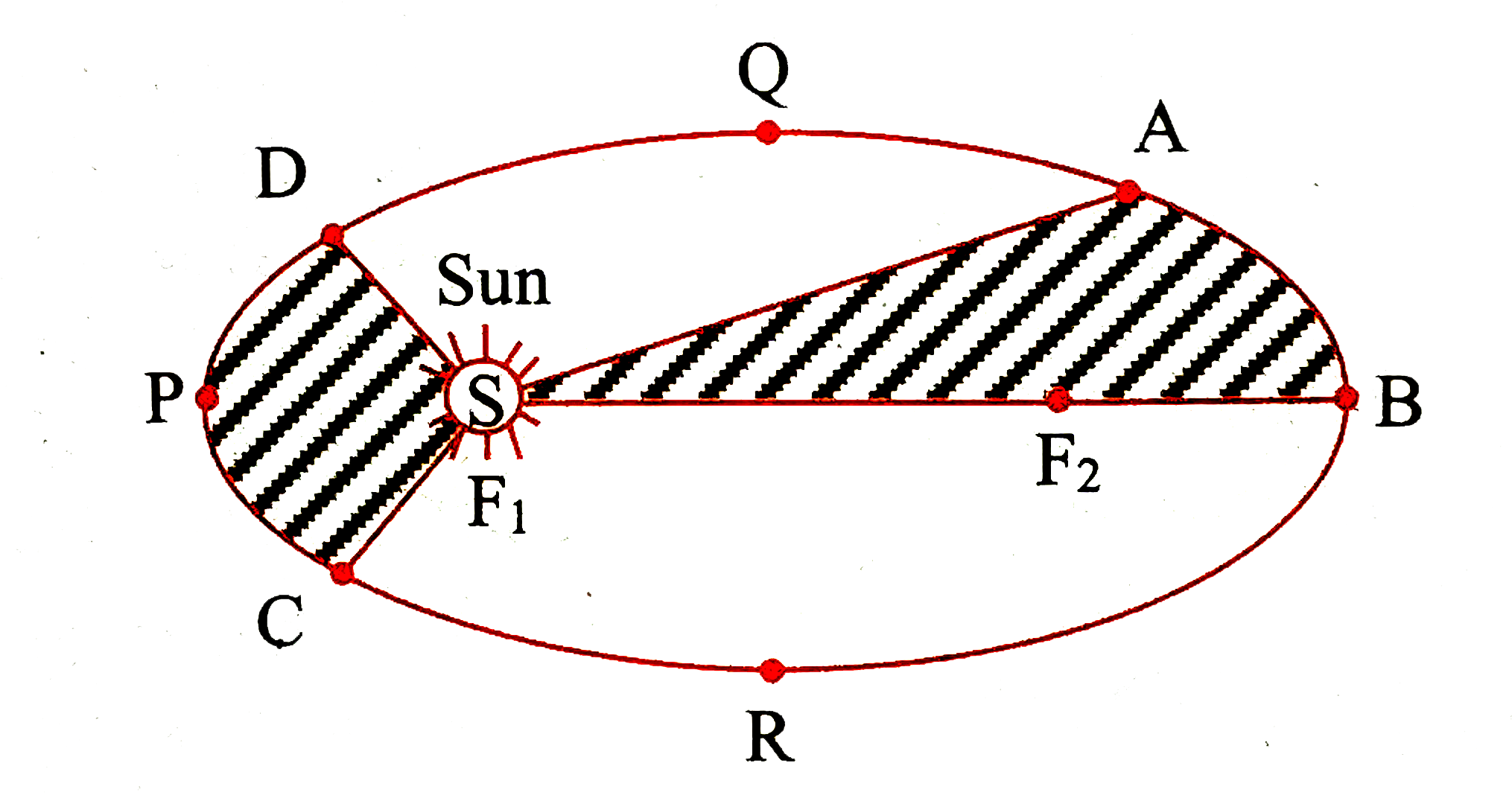
State the law regarding the time period of revolution of a planet .
View SolutionA planet revolves around the sun in an elliptical orbit with the sun at one focus as shown in the figure. The shaded areas are equal. If t1andt2 are the times taken by the planet to move from a to b and from c to d, respectively, then ………….. .
View Solution_S01_025_Q01.png)
The figure shows elliptical orbit of a planet m about the sun S. the shaded area SCD is twice the shaded area SAB. If t1 be the time for the planet to move from C to D and t2 is the time to move from A to B, then:
View Solution
