In the relation F=GMm/d2, the quantity G
Depends on the local value of g
Is used only when Earth is one of the two masses
is greatest at the surface of Earth
is a universal constant of nature
The correct Answer is:D
F=Gm1m2r2 , G is the universal gravitational constant
F∝m1m2 and F1∝1r2⇒F∝m1m2r2⇒F=Gm1m2r2
Hence G will be same for all pair of masses.
Similar Questions
Knowledge Check
The harmonic mean of two numbers is 4. Their arithmetic mean A and the geometric mean G satisfy the relation 2A+G2=27. Find two numbers.
A6, 3B5, 4C5, -2.5D−3,1The quantities AandB are related by the relation A/B=m, where m is the linear mass density and A is the force , the dimensions of B will be
ASame as that of pressureBSame as that of workCThat of momentumDSame as that of learnt heatThe quantities AandB are related by the relation, m=A/B, where m is the linear density and A is the force. The dimensions of B are of
ApressureBlatent heatCworkDNone of these
The harmonic mean between two numbers is 21/5, their A.M. 'A' and G.M. 'G' satisfy the relation 3A+G2=36. Then find the sum of square of numbers.
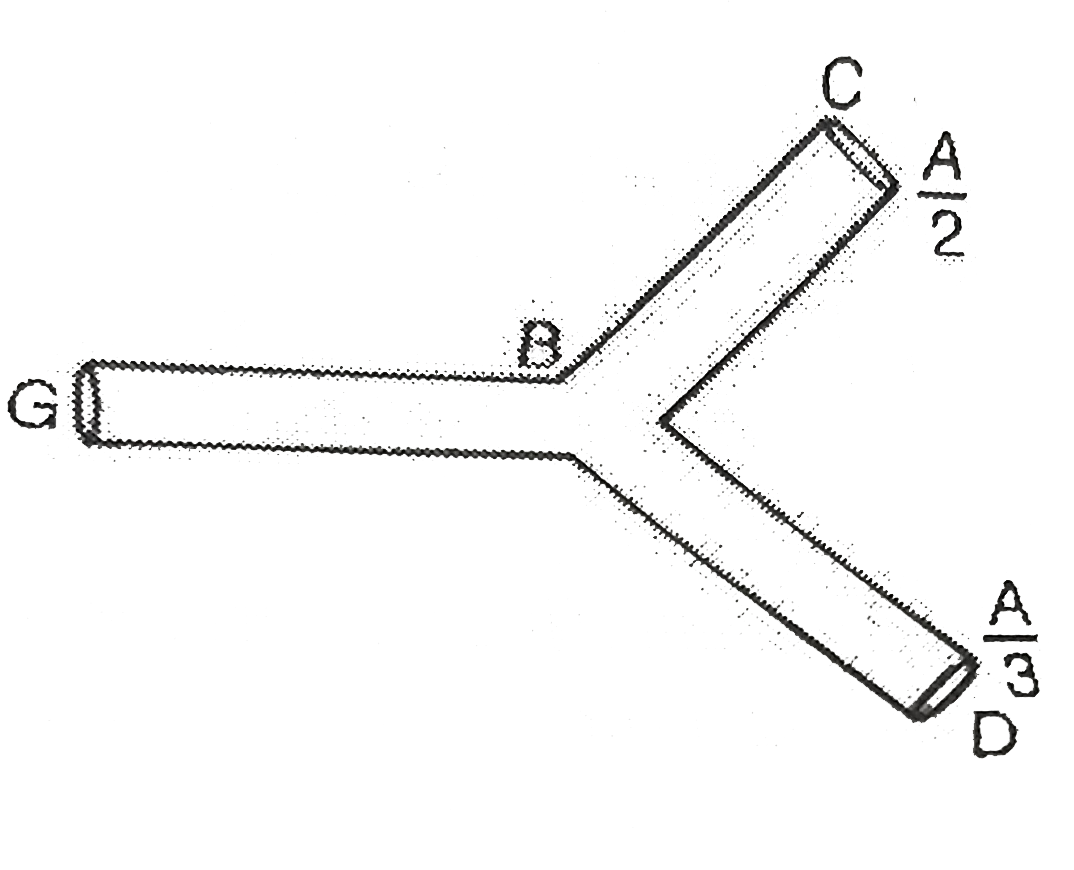
View SolutionA pipe GB is fitted withb two pipes C and D as shown in the figure. A=24m2 at G and velocity of water at G is 10 m/s and at C is 6 m/s . The velocity of water at D is
View SolutionIn the expression P=EI2m−5G9(−2), E, m, I and G denote energy, mass, angular momentum and gravitational constant, respectively. Show that P is a dimensionless quantity.
View SolutionIf R is a set of real numbers and f :R→R is given by the relation f (x) = sin x, x∈R and mapping g: R→R by the relation g(x)=x2,X∈R, then prove that fog≠gof.
View SolutionGiven that de,dm are densities of the Earth and moon, respectively, De,Dm are the diameters of the Earth and the moon, respectively. geandgm are the acceleration due to gravity on the surface of the Earth and moon, respectively. Find the ratio of gmandge
View Solution