What is the amount of work done in bringing a mass from the surface of Earth on one side to a point diametrically opposite on the other side?
To find the amount of work done in bringing a mass from one point on the surface of the Earth to a diametrically opposite point, we can follow these steps:
Step 1: Understanding the Concept of Work Done
Work done in moving an object in a gravitational field is related to the change in gravitational potential energy (U). The work done (W) can be calculated as the difference in potential energy at the two points.
Step 2: Identify the Initial and Final Points
Let’s denote:
- The initial point on the surface of the Earth as Point 1.
- The diametrically opposite point as Point 2.
Step 3: Calculate the Potential Energy at Point 1
The gravitational potential energy (U) at a point on the surface of the Earth is given by the formula:
U=−GMmR
Where:
- G = gravitational constant,
- M = mass of the Earth,
- m = mass of the object,
- R = radius of the Earth.
Thus, the potential energy at Point 1 (U_initial) is:
Uinitial=−GMmR
Step 4: Calculate the Potential Energy at Point 2
Since Point 2 is also on the surface of the Earth, the potential energy at Point 2 (U_final) is the same:
Ufinal=−GMmR
Step 5: Calculate the Work Done
The work done (W) in moving the mass from Point 1 to Point 2 is given by:
W=Ufinal−Uinitial
Substituting the values we found:
W=(−GMmR)−(−GMmR)
W=−GMmR+GMmR
W=0
Conclusion
The amount of work done in bringing a mass from the surface of the Earth to a point diametrically opposite on the other side is zero.
---
Work done in moving an object in a gravitational field is related to the change in gravitational potential energy (U). The work done (W) can be calculated as the difference in potential energy at the two points.
Let’s denote:
- The initial point on the surface of the Earth as Point 1.
- The diametrically opposite point as Point 2.
The gravitational potential energy (U) at a point on the surface of the Earth is given by the formula:
U=−GMmR
Where:
- G = gravitational constant,
- M = mass of the Earth,
- m = mass of the object,
- R = radius of the Earth.
Uinitial=−GMmR
Since Point 2 is also on the surface of the Earth, the potential energy at Point 2 (U_final) is the same:
Ufinal=−GMmR
The work done (W) in moving the mass from Point 1 to Point 2 is given by:
W=Ufinal−Uinitial
Substituting the values we found:
W=(−GMmR)−(−GMmR)
W=−GMmR+GMmR
W=0
The amount of work done in bringing a mass from the surface of the Earth to a point diametrically opposite on the other side is zero.
Similar Questions
Knowledge Check
The work done to raise a mass m from the surface of the earth to a height h, which is equal to the radius of the earth, is :
A32mgRBmgRC2mgRD12mgRThe work done to raise a mass m from the surface of the earth to a height h, which is equal to the radius of the earth is
AmgRB2mgRC12mgRD32mgRThere are three identical point mass bodies each of mass m locted at the vertices of an equilateral triangle with side r. They are experting gravitational force of attraction on each other, which can be given by Newton's law of gravitaiton. Each mass body produces its gravitational field in the surrounding region. the magnitude of gravitational field at a point due to a point mass body is the measure of gravitaitonal intensity at that point. The gravitational potential at a point in a gravitational field is the amount of workdone in bringing a unit mass body infinity to the given point without acceleration.
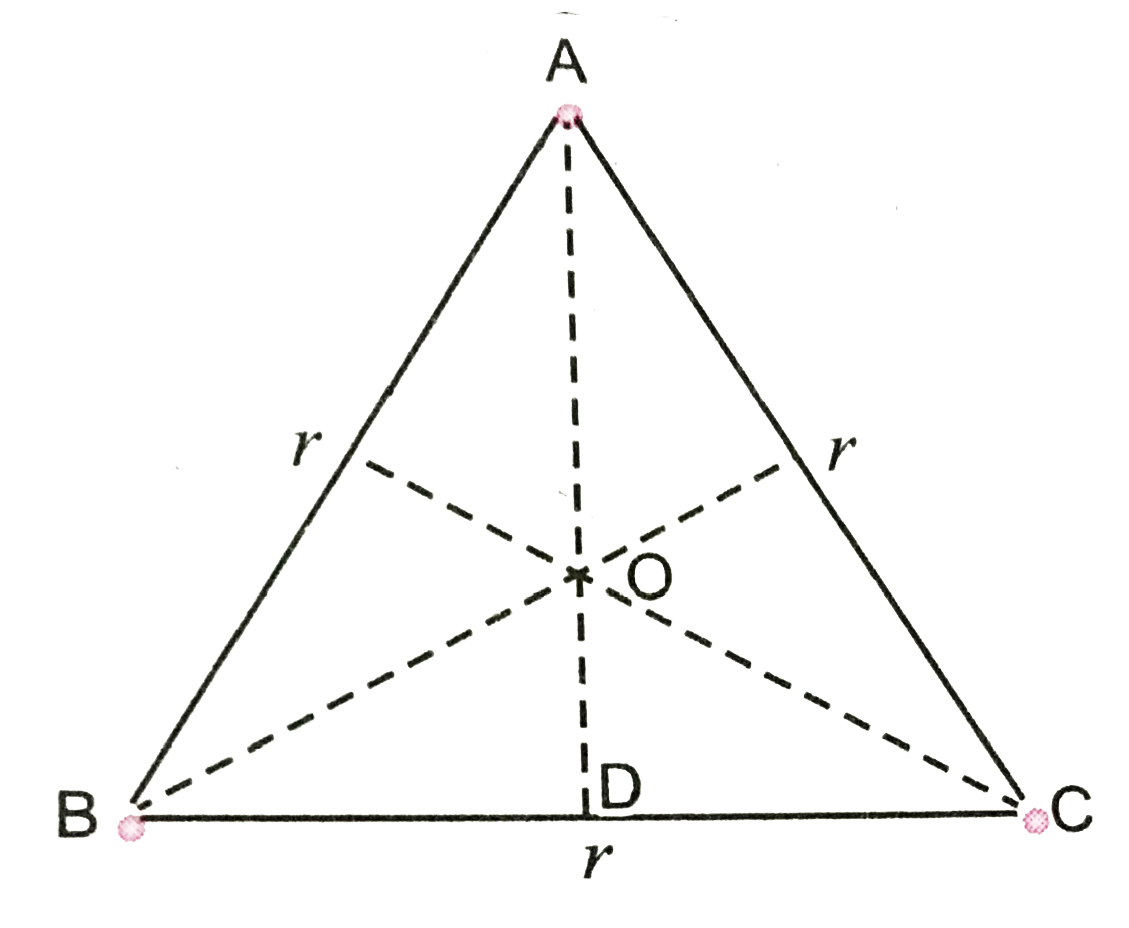
Answer the following questions :
Work done in taking one body far away from the other two bodies isA−Gm2rBGm2rC2Gm2rD−2Gm2r
The work done to take a particle of mass m from surface of the earth to a height equal to 2R is
View SolutionIn the previous problem, calculate the amount of work done in moving a charge of 0.1 nC from (i) centre of the square to the midpoint of side AB (ii) centre of the square to the midpoint of side BC.
View SolutionFrom the Earth, only one side of the moon is visible because it:
View SolutionAssertion : The work done in bringing a body down from the top to the base along a frictionless inclined plane is the same as the work done in bringing it down along the vertical side .
Reason : The gravitational force on the body along the inclined plane is the same as that along the vertical side.
View SolutionThe work done liftting a particle of mass 'm' from the centre of the earth to the surface of the earth is
View Solution
