The inclined surface is rough . For different values of m and M system slides down or up the plane or remains stationary. Match the appropriate entries of column-1 with those of column-2
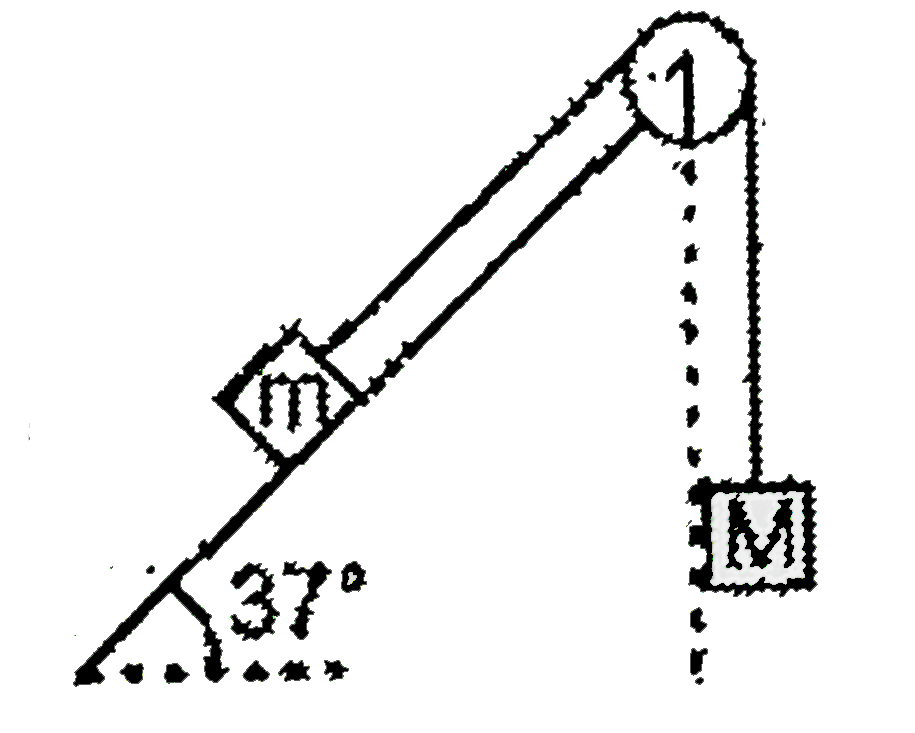
The correct Answer is:A, B, C, D
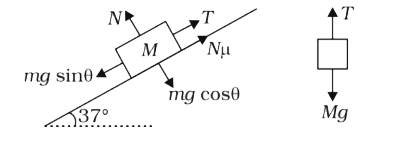
(B) 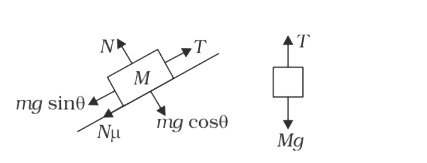
(C ) vertical component of acceleration of m
(D ) 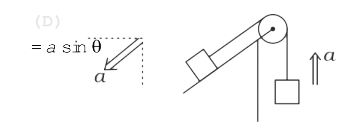 And vertical acc of M = a
And vertical acc of M = a
ratio
Similar Questions
Knowledge Check
Two blocks of mass 2m and m slide down the slope AB having same friction coefficient Then:
 Athe heavier mass reaches the bottom in a shorter timeBboth the masses have the same kinetic energy on arriving at BCthe heavier mass has twice the momentum than that of the other when reaching BDthe lighter mass reaches the bottom in a shorter time
Athe heavier mass reaches the bottom in a shorter timeBboth the masses have the same kinetic energy on arriving at BCthe heavier mass has twice the momentum than that of the other when reaching BDthe lighter mass reaches the bottom in a shorter timeA block of mas m begins to slide down on an inclined plane of inclination θ. The force of friction will be
AmgBmgcosθCmgsinθDmgtanθA body of mass m slides down a rough plane of inclination α . If μ is the coefficient of friction, then acceleration of the body will be
AgsinαBμcosαCg(sinα−μcosα)Dg(cosα−μsinα)
A body weighing 20 kg just slides down a rough inclined plane that rises 5 m in every 13 m. What is the coefficient of friction?
View SolutionTrajectory of a particle in a projectile motion is given by y=x−x280 where x and y are in meters. Match the column-1 and column-2.
Column-IColumn-II(A)x coordinate at height of 15 m(P) 20 m(B)vertical distnce of particle frompoint of projection at x= 100 m(Q) 80 m(C)Horizontal range(R) 60 m(S) 25 m
View SolutionThe coefficent of friction between the masses 2m and m is 0.5. All other surfaces are frictionless and pulleys are massless. Column I given the different values of m1 and column II gives the possible acceleration of 2m and m. Match the following :
Column IColumn II(A)m1=2m(P)Accelerations of 2m and m are same(B)m1=3m(Q)Acceleration of 2m and m are different(C)m1=4m(R)Acceleration of 2m is greater than m(D)m1=6m(S)Acceleration of 2m is less than 0.6 g(T)Acceleration of 2m is greater than 0.6 g
View SolutionMatch the corresponding entries of column 1 with column 2. [Where m is the magnification produced by the mirror.
Column 1Column 2(A)m=−2(a) Convex mirror(B)m=−12(b) Concave(C)m=+2(c) Real image(D)m=+12(d) Virtual image
View SolutionMatch the corresponding entries of column 1 with column 2. [where m is the magnification produced by the mirror

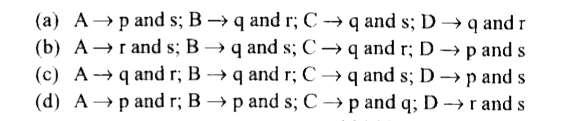
View Solution