What is the effect on the acceleration of a particle if the net force on the particle is doubled?
To solve the problem, we will use Newton's Second Law of Motion, which states that the net force acting on an object is equal to the mass of the object multiplied by its acceleration. The equation can be expressed as:
F=m⋅a
Where:
- F is the net force,
- m is the mass of the particle,
- a is the acceleration of the particle.
1. Identify the Initial Condition:
Let the initial net force acting on the particle be F and the initial acceleration be a. According to Newton's second law, we can express this as:
F=m⋅a
2. Doubling the Net Force:
If the net force is doubled, we have:
F′=2F
where F′ is the new net force.
3. Express the New Acceleration:
We can express the new acceleration a′ using the new net force:
F′=m⋅a′
Substituting the expression for F′:
2F=m⋅a′
4. Relate New Acceleration to Initial Acceleration:
Now, we can substitute the expression for F from the initial condition into this equation:
2(m⋅a)=m⋅a′
This simplifies to:
2a=a′
5. Conclusion:
Therefore, the new acceleration a′ is:
a′=2a
This means that if the net force on the particle is doubled, the acceleration of the particle will also be doubled.
Final Answer:
If the net force on the particle is doubled, the acceleration of the particle will also be doubled.
- F is the net force,
- m is the mass of the particle,
- a is the acceleration of the particle.
Let the initial net force acting on the particle be F and the initial acceleration be a. According to Newton's second law, we can express this as:
F=m⋅a
If the net force is doubled, we have:
F′=2F
where F′ is the new net force.
We can express the new acceleration a′ using the new net force:
F′=m⋅a′
Substituting the expression for F′:
2F=m⋅a′
Now, we can substitute the expression for F from the initial condition into this equation:
2(m⋅a)=m⋅a′
This simplifies to:
2a=a′
Therefore, the new acceleration a′ is:
a′=2a
This means that if the net force on the particle is doubled, the acceleration of the particle will also be doubled.
If the net force on the particle is doubled, the acceleration of the particle will also be doubled.
Similar Questions
A person says that he mesured the acceleration of a particle to be nonzero while no force was acting on the particle
View SolutionThe displacement x of a particle varies as
√x=t−2
(i) What is the velocity and acceleration of the particle at t=0?
(ii) When will the velocity of the particle become zero?
(iii) What is the displacement of the particle when its velocity is zero?
(iv) Is the motion of the particle uniformly accelerated or not?
View Solution
Knowledge Check
The acceleration of a particle is found to be non zero when no force acts on the particle. This is possible if the measurement is made from
Ainertial frameBnon inertial frameCbothDsome times inertial (or) some times non inertialThe acceleration of a particle is found to be non zero when no force acts on the particle. This is possible if the measurement is made from
Ainertial frameBnon inertial frameCbothDsome times intertial (or) some times non inertialIf a particle of mass m start from along curved circular path shown in figure with constant tangential acceleration a, then net force at B on the particle has the magnitude :
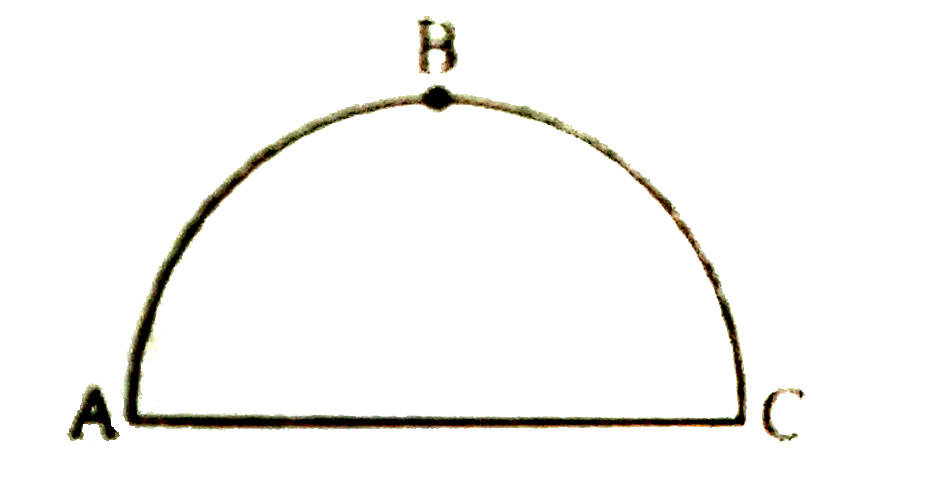 AzeroB2ma√1+π2Cma√1+π2Dmaπ√1+π
AzeroB2ma√1+π2Cma√1+π2Dmaπ√1+π
In fig. a central particle of charge −q is surrounded by two circular rings of charged particle of radii r and R, with Rgtr. what is the magnitude and dirction of the net electrostatic force on the central particle due to the other particles?

View SolutionTwo particles having charge Q1 and Q2 , when kept at a certain distance exert a force F on each other . If the distance between the two particles is reduced to half and the charge on each particle is doubled , the force between the particles would be
View SolutionChoose the correct combination of true (T) or fale (F) for the statements.
STATEMENT-1: A particle may be accelerating in a reference frame even if net extermal force on the particle is zero.
STATEMENT-2: Newton's laws are valid only in frames at rest.
STATEMENT-3: Work done by a force depends on reference frame.
View SolutionThe position at which the net force on the oscillating particle is zero, the postion is
View SolutionGraph shows the acceleration of a 3kg particles as an applied force moes it from rest along x axis. The total work done by the force on the particle by the time the particle reaches x=6m , is equal to
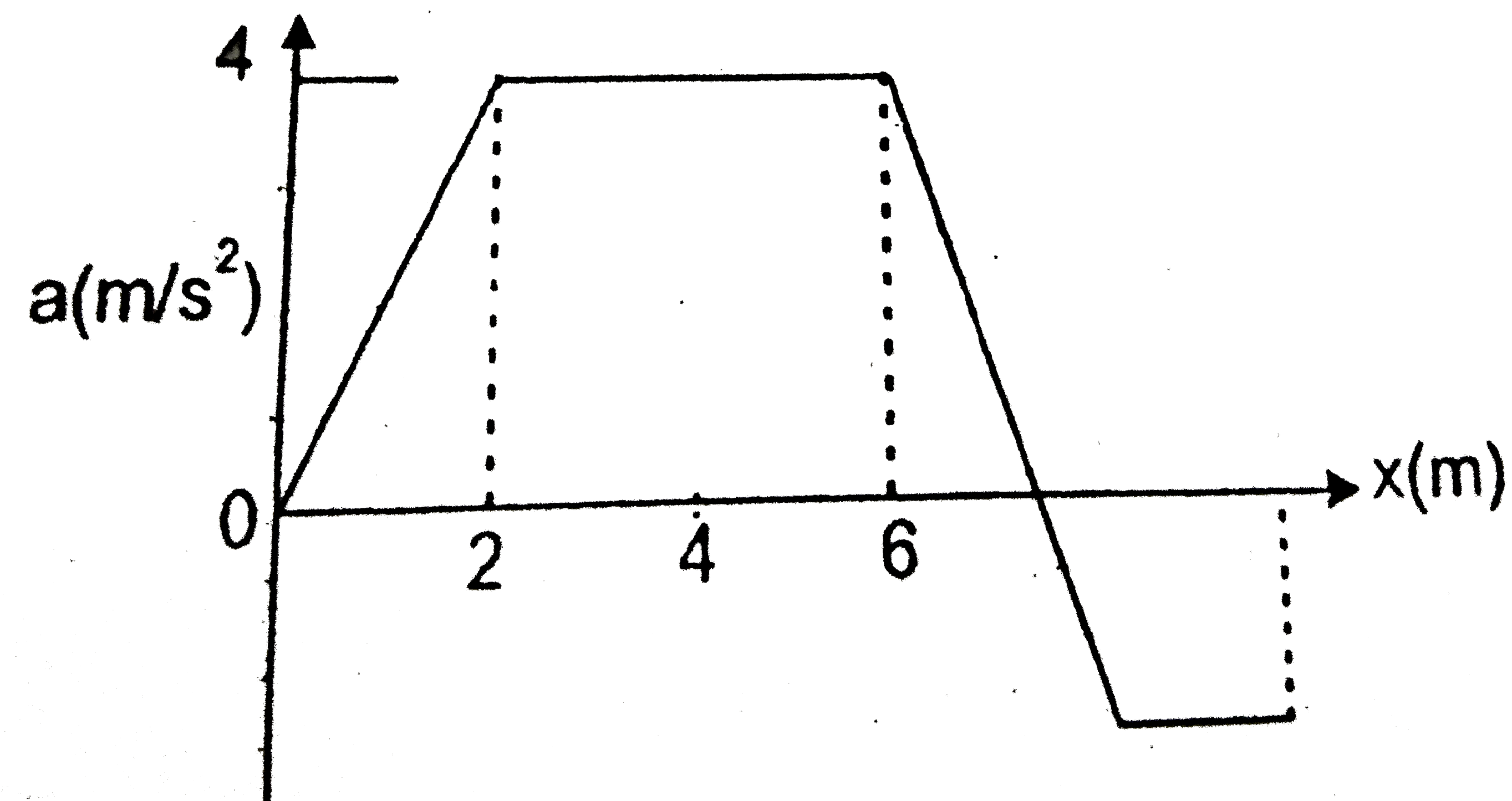
View Solution
