The time of flight of the projectile is:
a√2bg
a√bg
√2abg
√a2bg
The correct Answer is:A
To find the time of flight of a projectile given the equation of its path, we can follow these steps:
1. Understand the Equation of Motion:
The equation of the projectile's path is given as y=ax−bx2. Here, a and b are constants.
2. Determine the Maximum Height:
To find the maximum height (hmax), we need to find the value of x at which y is maximum. We do this by differentiating the equation with respect to x and setting the derivative to zero:
dydx=a−2bx=0
Solving for x:
2bx=a⇒x=a2b
3. Substitute x to Find Maximum Height:
Now, substitute x=a2b back into the original equation to find hmax:
h=a(a2b)−b(a2b)2
Simplifying this:
h=a22b−b(a24b2)=a22b−a24b=2a2−a24b=a24b
4. Calculate the Time of Flight:
The time of flight T for a projectile is given by the formula:
T=2√2hmaxg
Substituting hmax=a24b:
T=2
⎷2⋅a24bg=2√a22bg=2a√2bg
5. Final Expression:
Thus, the time of flight of the projectile is:
T=2a√2bg
The equation of the projectile's path is given as y=ax−bx2. Here, a and b are constants.
To find the maximum height (hmax), we need to find the value of x at which y is maximum. We do this by differentiating the equation with respect to x and setting the derivative to zero:
dydx=a−2bx=0
Solving for x:
2bx=a⇒x=a2b
Now, substitute x=a2b back into the original equation to find hmax:
h=a(a2b)−b(a2b)2
Simplifying this:
h=a22b−b(a24b2)=a22b−a24b=2a2−a24b=a24b
The time of flight T for a projectile is given by the formula:
T=2√2hmaxg
Substituting hmax=a24b:
T=2
⎷2⋅a24bg=2√a22bg=2a√2bg
Thus, the time of flight of the projectile is:
T=2a√2bg
Similar Questions
The vertical and horizontal displacements of a projectile thrown from a point on the ground at time t are given by y=6t−5t2 and x=8t respectively,where x and y are in meters and t is in seconds.The time of flight of the projectile in seconds is ?
View SolutionA ball is thrown with a speed u, at an angle θ with the horizontal. At the highest point of its motion, the strength of gravity is somehow doubled. Taking this change into account, the total time of flight of the projectile is
View Solution
Knowledge Check
The time of flight of a projectile is 10 s and range is 500m. Maximum height attained by it is [g=10m/s2]
A25mB50mC82mD125mThe trajectory of a projectile in a vertical plane is y=√3x−2x2. [g=10m/s2]

Time of flight of the projectile is :-A√310sB√103sC1sD2sNeglecting the air resistance, the time of flight of a projectile is determined by
AUverticalBUhorizontalCU=U2vertical+U2horizontalDU=U(U2vertical+Uhorizontal)1/2
The time of flight of a projectile is related to its horizontal range by the equation gT2=2R.The angle of projection is?
View SolutionIn the figure shown, find
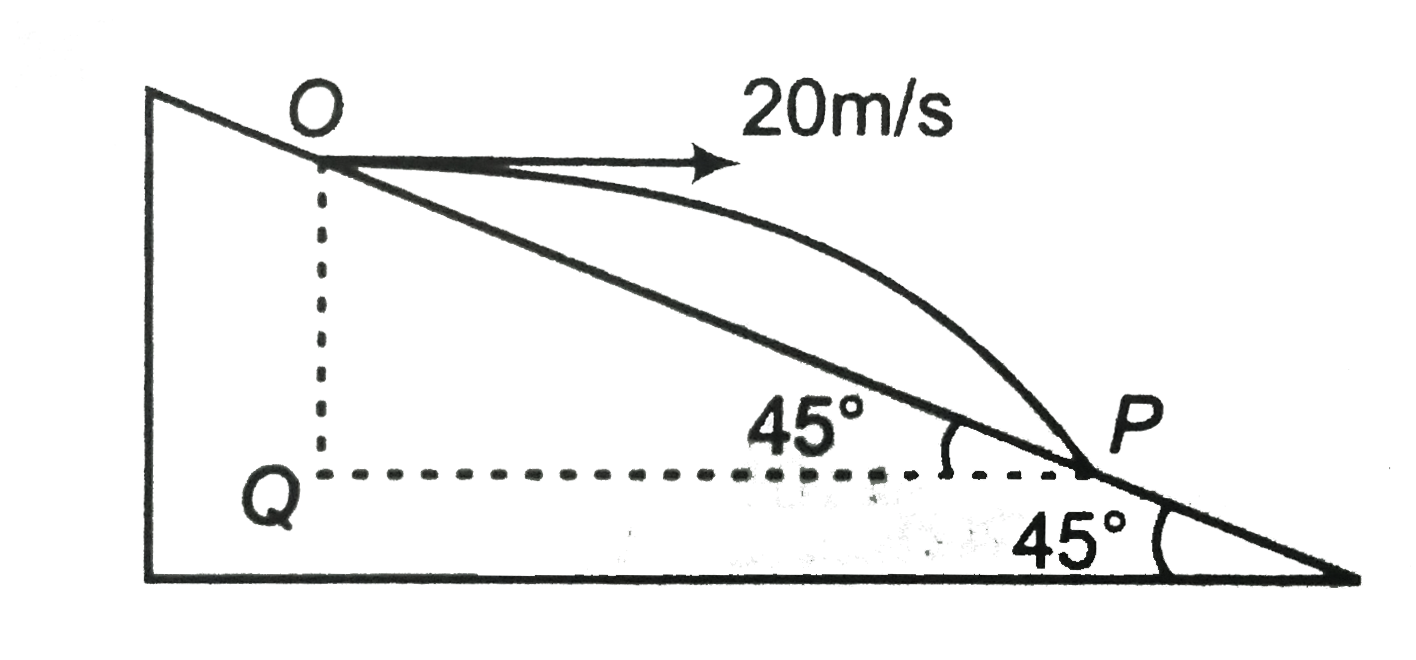 .
.
(a) the time of flight of the projectile over the inciled plane.
(b) range OP.
View SolutionIf the time of flight of a projectile is doubled, what happens to the maximum height at tained?
View SolutionThere are two values of time for which a projectile is at the same height. The sum of these two times is equal to (T = time of flight of the projectile)
View SolutionThe time of flight of a projectile on an upwardd inctined plane depends upon
View Solution
