In a triangle ABC , if B=300and c=√3 b, then A can be equal to 450 (b) 600 (c) 900 (d) 120^0
Step by step video & image solution for In a triangle A B C , if B=30^0a n d\ c=sqrt(3)\ b , then A can be equal to 45^0 (b) 60^0 (c) 90^0 (d) 120^0 by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - BASIC MATHS,LOGARITHIM, TRIGNOMETRIC RATIO AND IDENTITIES AND TRIGNOMETRIC EQUATION EXERCISE - EXERCISE (S-1)44videos
BASIC MATHS,LOGARITHIM, TRIGNOMETRIC RATIO AND IDENTITIES AND TRIGNOMETRIC EQUATION
- BOOK - ALLENCHAPTER - Solutions of Triangle & Binomial TheoremEXERCISE - Do yourself -64videos
Solutions of Triangle & Binomial Theorem
Similar Questions
In a parallelogram ABCD , if ∠DAB=75∘and ∠DBC=60∘, then ∠BDC=(a) 75∘ (b) 60∘ (c) 45∘ (d) 55∘
View SolutionIn ΔABC , if A=30∘,C=900,c=7√3 then a+b=
View SolutionIn a triangle ABC if a4+b4+c4=2c2(a2+b2), then angle C is equal to (A) 600 (B) 1200 (C) 450 (D) 1350
View SolutionIf A=30∘.C=900,c=7√3 then find a and b
View SolutionIf in a triangle ABC, b=√3, c=1 and B−C−900, then angle A is
View SolutionIn a ΔABC, if b=√3, c=1 and ∠A=300, then find a
View SolutionThe angles of a triangle are in the ratio 1:2:3. The measure of the largest angle is: 300 (b) 600 (c) 900 (d) 1200
View SolutionABC is an isosceles triangle such that AB=ACandAD is the median to base BC . Then, ∠BAD=
(a) 55∘
(b) 70∘
(c) 35∘
(d) 110∘
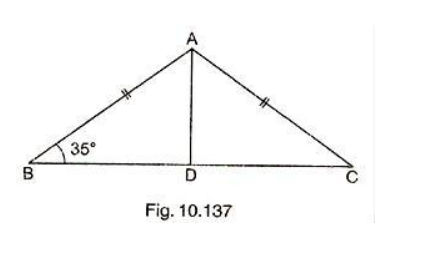 View Solution
View SolutionThe diagonals of a parallelogram ABCD intersect at O . If ∠BOC=90∘ and ∠BDC=50∘, then ∠OAB= 40∘ (b) 50∘ (c) 10∘ (d) 900
View SolutionIn triangle ABC , if b=3, c=4 and ∠B=π3, then number of such triangle is 1 (b) 2 (c) 0 (d) infinite
View Solution
