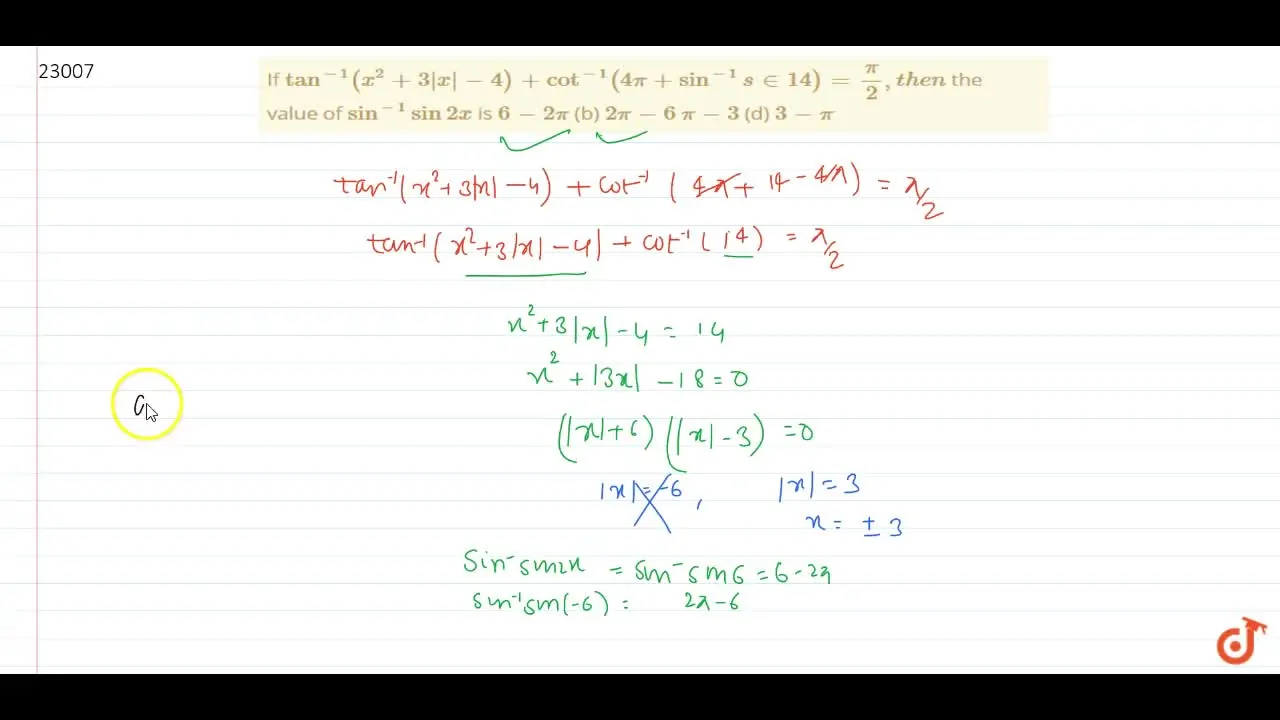
If tan−1(x2+3|x|−4)+cot−1(4π+sin−1sin14)=π2,then the value of sin−1sin2x is (a)6−2π (b) 2π−6 (c)π−3 (d) 3−π
Step by step video & image solution for If tan^(-1)(x^2+3|x|-4)+cot^(-1)(4pi+sin^(-1)sin 14)=pi/2, t h e n the value of sin^(-1)sin2x is (a)6-2pi (b) 2pi-6 (c)pi-3 (d) 3-pi by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
If tan−1(x2+3|x|−4)+cot−1(4π+sin−1sin14)=π2,then the value of sin−1sin2x is (a)6−2π (b) 2π−6 (c)π−3 (d) 3−π
View SolutionIf tan−1(x2+3|x|−4)+cot−1(4π+sin−1sin14)=π2, then the value of sin−1(sin2x) can be equal to
View SolutionIf tanx=−34 and 3π2<x<2π, then the value of sin2x is
View SolutionIf sin−1x+sin−1y=2π3 ,then the value of cos−1x+cos−1y is
(A) 2π3
(B) π3
(C) π2
(D) πView SolutionFind the value of ∫π3π4sin2x1−cos2xdx
View SolutionThe value of ∫π2−π2sin2x1+2xdx is: (A) π (B) π2 (C) 4π (D) π4
View SolutionFind the value of
sin−1(sin2π3)+cos−1(cos4π3)View Solutionsin−1(−12)
(a) π3
(b) −π3
(c) π6
(d) −π6View SolutionThe value of cos−1(−1)−sin−1(1) is: a. π b. π2 c. 3π2 d. −3π2
View SolutionIf sin−1(tanπ/4)−sin−1(√3/x)−π/6=0, then x is a root of the equation
View Solution