The positive integer value of n>3 satisfying the equation 1sin(πn)=1sin(2πn)+1sin(3πn)is
1sin(πn)=1sin(2πn)+1sin(3πn)
⇒1sin(πn)−1sin(3πn)=1sin(2πn)
⇒sin(3πn)−sin(πn)sin(3πn)sin(πn)=1sin(2πn)
⇒2sin(πn)cos(2πn)sin(3πn)sin(πn)⋅sin(2πn)=1
⇒2sin(2πn)cos(2πn)sin(3πn)=1
⇒sin(4πn)sin(3πn)=1
⇒sin(4πn)=sin(3πn)
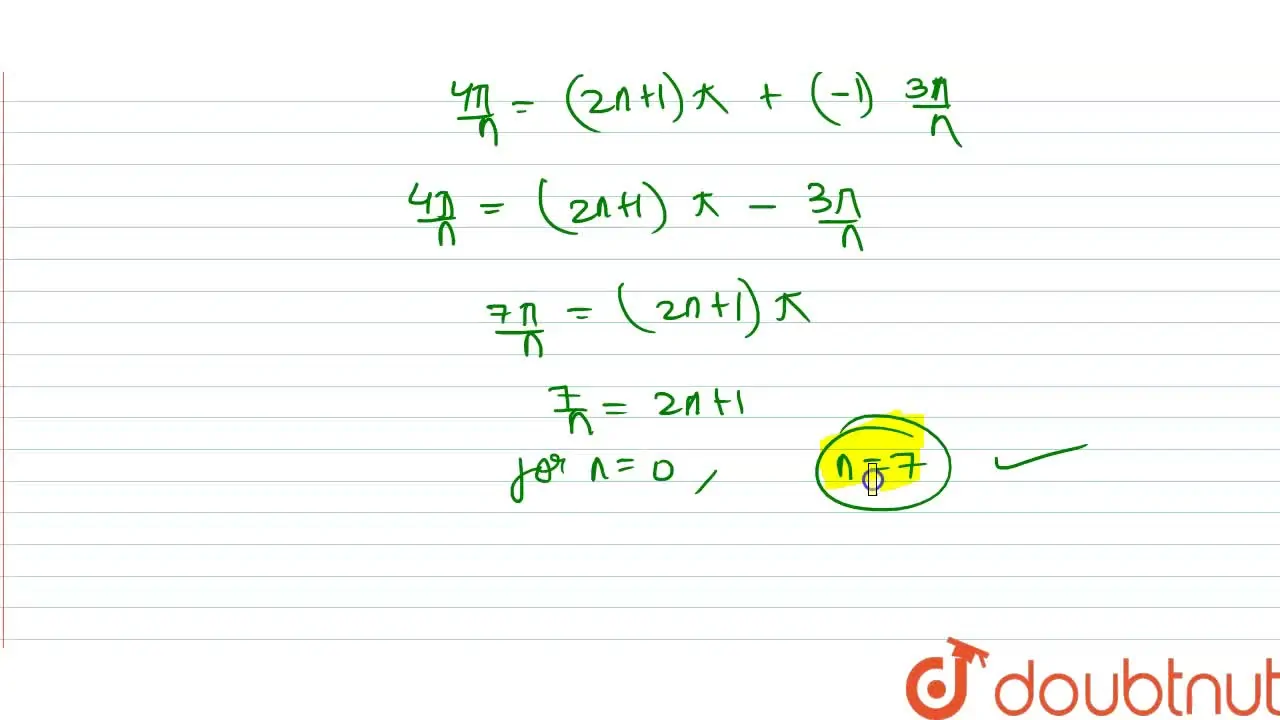
⇒sin(π−4πn)=sin(3πn)
⇒π−4πn=3πn
=π=7πn
⇒n=7.
Related Playlists
Similar Questions
The positive integer value of n>3 satisfying the equation 1sin(πn)=1sin(2πn)+1sin(3πn) is
View SolutionThe positive integer value of n>3 satisfying the equation 1sin(πn)=1sin(2πn)+1sin(3πn)is
View SolutionThe positive integer value of n>3 satisfying the equation 1sin(πn)=1sin(2πn)+1sin(3πn) is
View SolutionEvaluate sin(πn)+sin(3πn)+sin(5πn)+........ to n terms.
View SolutionThe smallest positive integral value of 'n' such that [1+sin π8+i cos π81+sin π8−i cos π8]n is purely imaginary if
View SolutionThe set of values of x satisfying the equation sin3α=4sinαsin(x+α)sin(x−α) is nπ±π4,∀n∈Z nπ±π3,∀n∈Z nπ±π9,∀n∈Z nπ±π12,∀n∈Z
View SolutionThe value of z satisfying the equation logz+logz2+.+logzn=0is cos(4mπ)n(n+1)+isin(4mπ)n(n+1),m=12 cos(4mπ)n(n+1)−isin(4mπ)n(n+1),m=12 sin(4mπ)n(n+1)+isin(4mπ)n(n+1),m=12 0
View SolutionWhich of the following set of values of x satisfies the equation 22sin2x−3sinx+1+22−2sin2x+3sinx=9? (a)x=nπ±π6,n∈I (b) x=nπ±π3,n∈I (c)x=nπ,n∈I (d) x=2nπ+π2,n∈I
View SolutionThe general values of θ satisfying the equation 2sin2θ−3sinθ−2=0 is (n∈Z). nπ+(−1)nπ6 (b) nπ+(−1)nπ2 nπ+(−1)n5π6 (d) nπ+(−1)n7π6
View SolutionThe number of positive integers which satisfy sin(π4n)+cos(π4n)=√n2 is -
View Solution