Using the method of integration find the area bounded by the curve |x|+|y|=1.[Hint: The required region is bounded by lines x+y=1,x−y=1,−x+y=1and−x−y=1].
Video Solution
Text Solution
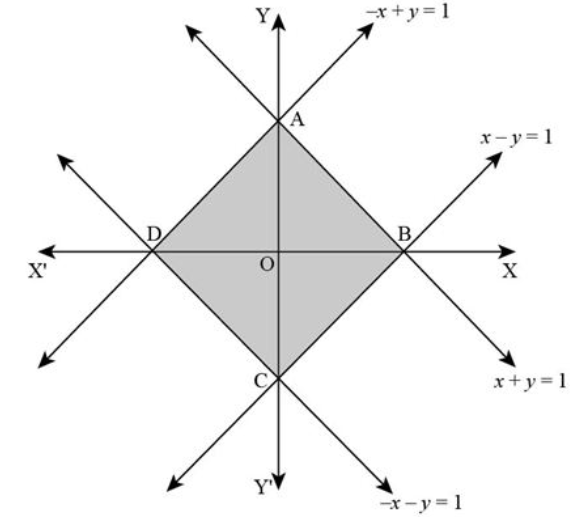
The given curve is |x|+|y|=1. The area of the region bounded by the given curve is represented by the shaded region ABC
The point of intersection of lines −x+y=1 and x+y=1 is (0,1).
The point of intersection of lines x−y=1 and x+y=1 is (1,0).
The point of intersection of lines −x−y=1 and x−y=1 is (0,−1).
The point of intersection of lines −x+y=1 and −x−y=1 is (−1,0).
So, the curve intersect the axes at points A(0,1),B(1,0),C(0,−1)andD(−1,0).
Since the values of x and y correspond to each other, then it is observed that the given curve is symmetrical about x-axis and y-axis.The area of the shaded region is
AreaABCD=4×AreaOABO
So the area of OBAO is,
AreaOBAO=∫10(1−x)dx
This gives the area of ABCD as,
Area of ABCD= 4∫10(1−x)dx=4(x−x22)10=2 sq. unit
|
Updated on:21/07/2023
Related Playlists
- BOOK - NCERTCHAPTER - APPLICATION OF INTEGRALSEXERCISE - SOLVED EXAMPLES15videos
APPLICATION OF INTEGRALS
- BOOK - NCERTCHAPTER - APPLICATION OF INTEGRALSEXERCISE - EXERCISE 8.27videos
APPLICATION OF INTEGRALS
- BOOK - NCERTCHAPTER - APPLICATION OF INTEGRALSEXERCISE - EXERCISE 8.27videos
APPLICATION OF INTEGRALS
- BOOK - NCERTCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - EXERCISE 6.118videos
APPLICATION OF DERIVATIVES
- BOOK - NCERTCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - QUESTION3videos
CONTINUITY AND DIFFERENTIABILITY
Similar Questions
Find the area bounded by the curves x+2|y|=1 and x=0 .
View SolutionUsing integration, find the area bounded by the curves y=|x−1| and y=3−|x|.
View SolutionFind the area bounded by the curve |x|+y=1 and axis of x.
View SolutionThe area bounded by the curve y=|x−1| and y=3−|x|
View SolutionThe area bounded by the curve y=|x|−1andy=−|x|+1 is
View SolutionUsing integration, find the area bounded by the lines x+2y=2, y−x=1 and 2x+y=7
View SolutionThe area bounded by the curves y=|x|−1andy=−|x|+1 is 1 b. 2 c. 2√2 d. 4
View SolutionFind the area bounded by curve y=x2−1 and y = 1 .
View SolutionArea bounded by the curves y=x2−1,x+y=3 is
View SolutionFind the area of the bounded by the curve y2=2x+1 and the line x-y=1
View Solution


