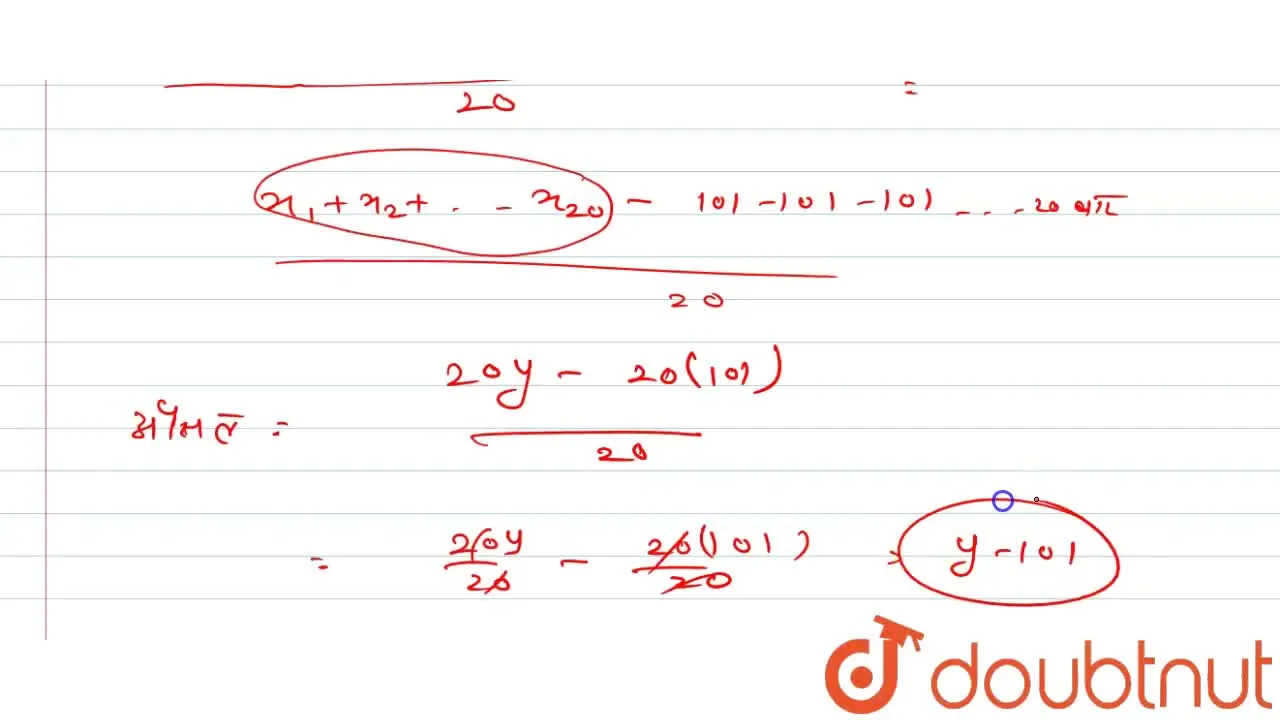
20, मापनो x1,x2,x3,...........x20 का औसत y, है। तब, (x1−101),(x2−101),(x3−101)......(x20−101) का औसत ज्ञात करे?
y−20
y−101
20y
101y
The correct Answer is:B
According to question
x1+x2+x3+x4+…..+x2020=y
x1+x2+x3+x4+....+x20=20y
⇒x1−101+x2−101+x3−101+.....x20−10120
⇒(x1+x2+x3+...+x20)−(20×101)20
⇒20y−20×10120
⇒ y-101
Related Playlists
Similar Questions
यदि a∗b=2a−3b+ab है तो 3∗5+5∗3 किसके बराबर होगा?
04:12View Solutionएक व्यक्ति धारा के प्रतिकूल 750 मीटर की दूरी 675 सेकण्ड में तय करती है तथा 712 मिनट में वापस आती है। शान्त जल में उसकी चाल (किलोमीटर प्रति घंटा) क्या है ?
02:52View SolutionThe number of solutions to the system of equations x1+x2+x3+x4+x5=20 and x1+x2=15 wher xk≥0
09:09View SolutionIf x1,x2,x20 are in H.P. and x1,2,x20 are in G.P., then 19∑r=1xrxr+1= 76 b. 80 c. 84 d. none of these
View SolutionFind the minimum value of log_(x_(1))(x_(2)-(1)/(4))+log_(x_(2))(x_(3)-(1)/(4))+......+log_(x_(20))(x_(1)-(1)/(4)) over all x_(1),x_(2),...x_(20)in((1)/(4),1)
05:52View SolutionConsider any set of observations x1,x2,x3,..,x101. It is given that x1<x2<x3<..Ltx100<x101, then the mean deviation of this set of observations about a point k is minimum when k equals
04:29View SolutionLet x1,x2,…..,xn be is an AP . If x1+x4+x9+x11+x20+x22+x27+x30=272, then x1+x2+x3+…+x30 is equal to
01:53View SolutionThe number of solutions of the equation x1+x2+x3+x4+x5=101, where xi's are odd natural numbers is :
08:00View SolutionConsider the observation x1=1,x2,x3=3,.............x100=100
x101,x102,x103,x104=104 Median of the given data is08:00View SolutionConsider the Observations x1=1,x2=2,x3=3,.....x100=100,x101=101,x102=102,x103=103,x104=104
Quarter deviation of the given data is05:00View SolutionConsider the Observations x1=1,x2=2,x3=3,.....x100=100,x101=101,x102=102,x103=103,x104=104
Mean deviation from the median of the given data is03:05View SolutionIf x_(1),x_(2),….x_(20) are in H.P then x_(1)x_(2)+x_(2)x_(3)+….+x_(19)x_(20) =
02:29View SolutionLet x1,x2,……,xn be in an A.P. If x1+x4+x9+x11+x20+x22+x27+x30=272, then x1+x2+x3+......+x30 is equal to
01:23View SolutionConsider any set of observations x1,x2,x3,..,x101. It is given that x1<x2<x3<..Ltx100<x101, then the mean deviation of this set of observations about a point k is minimum when k equals
03:17View SolutionIf average of 20 observation x1,x2,……x20 is y . then the average of x1−101,x2−101,x3−101,…….X20−101 is :
03:47View Solution