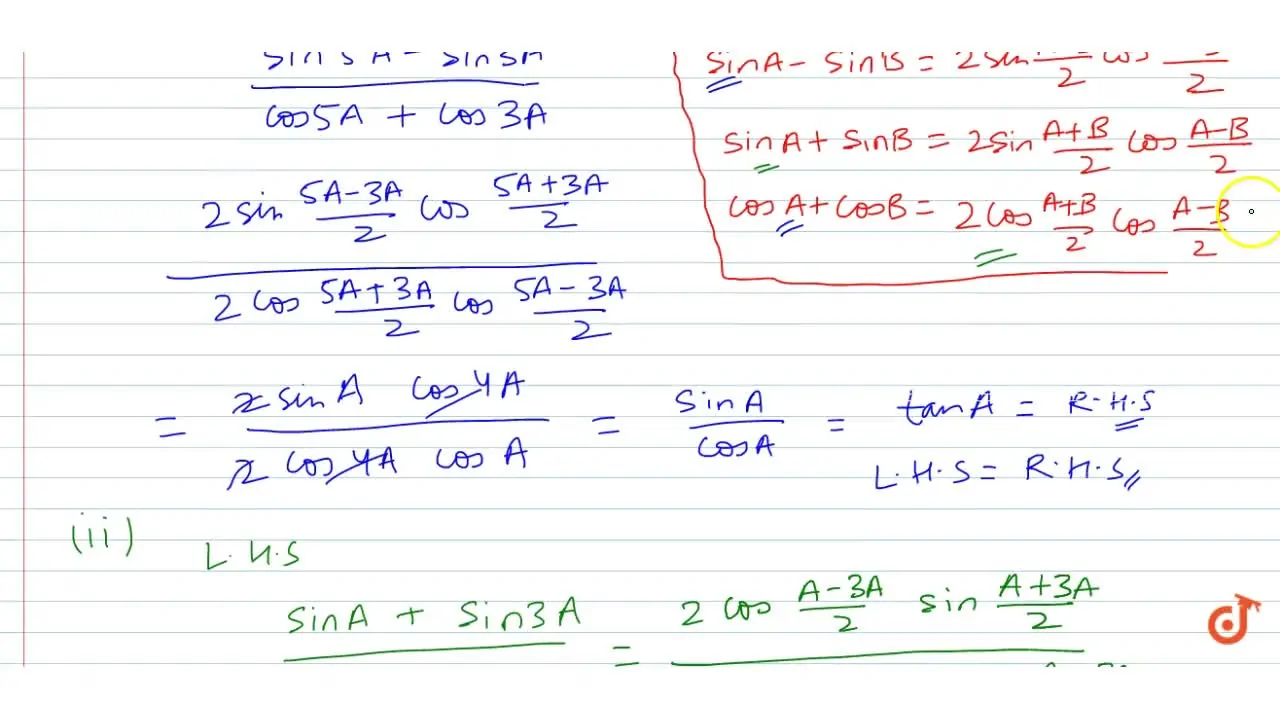
Prove that: sin5A−sin3Acos5A+cos3A=tanA sinA+sin3AcosA+cos3A=tan2A
Video Solution
Text Solution
1)=sin5A−sin3Acos5A+cos3A
=2sin(5A−3A2)cos(5A+3A2)2cos(5A+3A2)cos(5A−3A2)
=sinAcosA
=tanA
RHS
2)=sinA+sin3AcosA+cos3A
=2cos(A−3A2)sin(A+3A2)2cos(A+3A2)cos(A−3A2)
=2cos(−A)sin2A2cos2Acos(−A)
=sin2Acos2A
=tan2A
RHS
|
Updated on:21/07/2023
Related Playlists
Similar Questions
Prove that sin5A−sin3Acos5A+cos3A=tanA
View SolutionProve that
sin5A−sin3Acos5A+cos3A=tanAView SolutionsinA+sin3AcosA+cos3A=tan2A
View SolutionProve that: sin5A−sin3Acos5A+cos3A=tanA sinA+sin3AcosA+cos3A=tan2A
View SolutionProve that: sin5x+sin3xcos5x+cos3x=tan4x
View SolutionProve that:sin5x+sin3xcos5x+cos3x=tan4x
View Solutionsin5x+sin3xcos5x+cos3x=tan4x
View SolutionProve that: sinA−2sin3A2cos3A−cosA=tanA
View SolutionProve that sinA−2sin3A2cos3A−cosA=tanA
View SolutionProve that sin2A+sin5A−sinAcos2A+cos5A+cosA=tan2A
View Solution