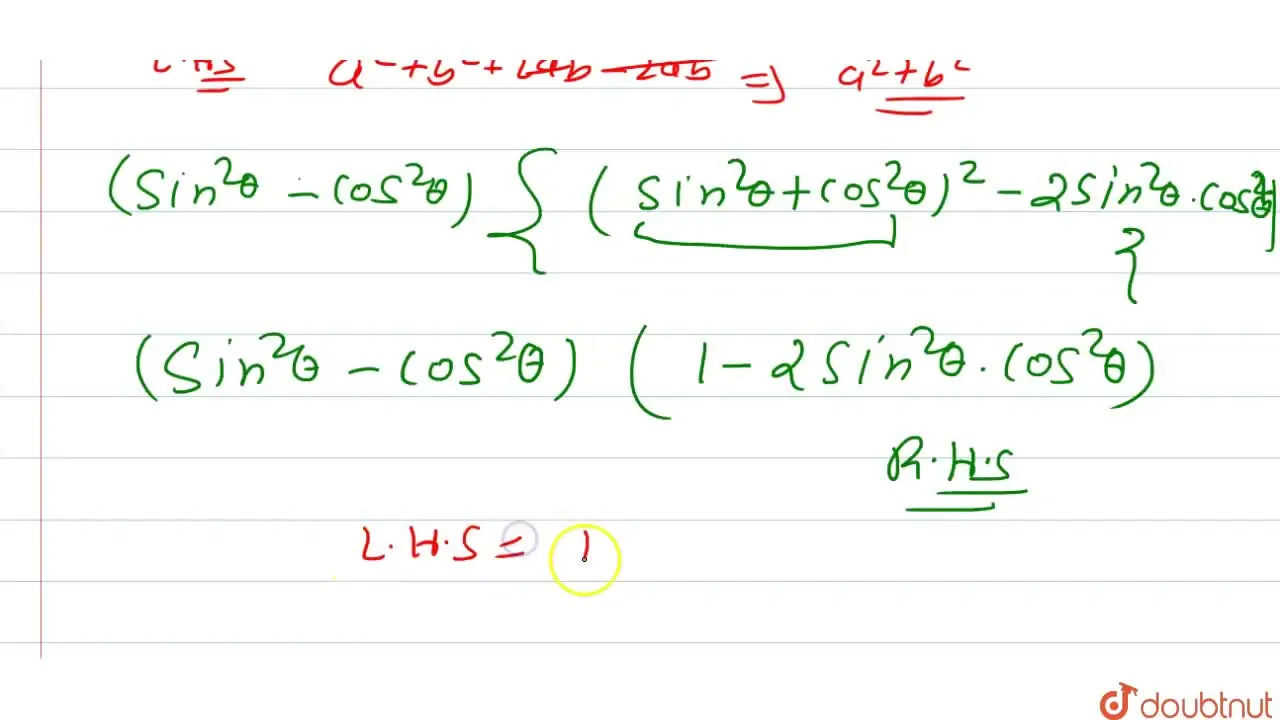निम्नलिखित को सर्वसमिकाओ की सहायता से सिद्ध कीजिए।
Q.sin8θ−cos8θ=(sin2θ−cos2θ)(1−2sin2θcos2θ)
Step by step video & image solution for निम्नलिखित को सर्वसमिकाओ की सहायता से सिद्ध कीजिए। Q.sin^8theta-cos^8theta=(sin^2theta-cos^2theta) (1-2sin^2theta cos^2theta) by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Related Playlists
- BOOK - SANJEEV PUBLICATIONCHAPTER - त्रिकोणमितीय सर्वसमिकाएँEXERCISE - प्रश्नमाला 7.228videos
त्रिकोणमितीय सर्वसमिकाएँ
- BOOK - SANJEEV PUBLICATIONCHAPTER - त्रिकोणमितीय सर्वसमिकाएँEXERCISE - अन्य महत्वपूर्ण प्रश्न (वस्तुनिष्ठ प्रश्न)9videos
त्रिकोणमितीय सर्वसमिकाएँ
- BOOK - SANJEEV PUBLICATIONCHAPTER - त्रिकोणमितीय अनुपात EXERCISE - अन्य महत्त्वपूर्ण प्रश्न (निबन्धात्मक प्रश्न )3videos
त्रिकोणमितीय अनुपात
- BOOK - SANJEEV PUBLICATIONCHAPTER - दो चर राशियों पर आधारित समस्याए EXERCISE - अन्य महत्वपूर्ण प्रश्न ( लघुत्तरत्मक प्रश्न )6videos
दो चर राशियों पर आधारित समस्याए
Similar Questions
निम्नलिखित के मान ज्ञात करो-
Q.cos37∘sin53∘03:25View Solutiontanθ√1+tan2θ बराबर है -
03:51View Solutionयदि sin(A+B)=1तथा cos(A−B)=√32यहाँ 0∘<(A+B)≤90∘,A>Bहो,तो A तथा B के मान ज्ञात कीजिय।
03:06View Solutionकिसी नगर में टैक्सी का किराया पहले किलोमीटर का 9 रूपए, उसके बाद के लिए 6 रूपए है। यदि तय की गयी दूरी x किमी. और किराया y रूपए है तो इसे समीकरण के रूप में व्यक्त कीजिये।
06:39View SolutionIf cos2θ+cos8θ=cos5θ then θ=
04:28View Solutionसिद्ध कीजिए की
sin8θcosθ−sin6θcos3θcos2θcosθ−sin3θsin4θ=tan2θ02:58View Solutionसिद्ध कीजिए की
1+sin2θ−cos2θ1+sin2θ+cos2θ=tanθ02:39View SolutionProve the following identities: 2(sin6θ+cos6θ)−3(sin4θ+cos4θ)+1=0 sin6θ+cos6θ+3sin2θcos2θ=1 (sin8θ−cos8θ)=(sin2θ−cos2θ)(1−2s∈2θcos2θ)
04:38View SolutionProve the following identities: sin8θ−cos8θ=(sin2θ−cos2θ)(1−2sin2θcos2θ)
02:16View SolutionProve: (1+ sin 2theta+cos 2theta)/(1+sin 2theta-cos 2theta)=cot theta
03:48View Solutionsin2θ+cos2θ=1 .
03:23View SolutionWhat is the value of abs([sin^2theta,cos^2theta,1],[cos^2theta,sin^2theta,1],[-10,12,2]) ?
03:13View SolutionEvaluate the following : ⎡⎢⎣sin2θcos2θ1cos2θsin2θ1−10122⎤⎥⎦
03:49View Solutioncos2θ+sin2θis
01:20View SolutionProve the following
sin4θ−cos4θ=sin2θ−cos2θ=1−2cos2θ=2sin2θ−101:46View Solution