If the radius of gyration of a solid disc of mass 10 kg about an axis is 0.40 m, then the moment of inertia of the disc about that axis is
1.6kg m2
3.2kg m2
6.4kg m2
9.5kg m2
The correct Answer is:A
To find the moment of inertia (I) of a solid disc about a given axis using the radius of gyration (k), we can use the formula:
I=m⋅k2
Where:
- I is the moment of inertia,
- m is the mass of the disc,
- k is the radius of gyration.
1. Identify the given values:
- Mass of the disc, m=10kg
- Radius of gyration, k=0.40m
2. Substitute the values into the formula:
I=m⋅k2
I=10kg⋅(0.40m)2
3. Calculate k2:
(0.40m)2=0.16m2
4. Multiply the mass by k2:
I=10kg⋅0.16m2=1.6kg⋅m2
5. Final Result:
The moment of inertia of the disc about the given axis is:
I=1.6kg⋅m2
Conclusion:
Thus, the moment of inertia of the solid disc about the specified axis is 1.6kg⋅m2.
---
- I is the moment of inertia,
- m is the mass of the disc,
- k is the radius of gyration.
- Mass of the disc, m=10kg
- Radius of gyration, k=0.40m
I=m⋅k2
I=10kg⋅(0.40m)2
(0.40m)2=0.16m2
I=10kg⋅0.16m2=1.6kg⋅m2
The moment of inertia of the disc about the given axis is:
I=1.6kg⋅m2
Thus, the moment of inertia of the solid disc about the specified axis is 1.6kg⋅m2.
Topper's Solved these Questions
- ROTATIONAL MOTION Book:MOTIONChapter:ROTATIONAL MOTION Exercise:Exercise - 3 ( Section-A )
Explore 46 Videos
- ROTATIONAL MOTION Book:MOTIONChapter:ROTATIONAL MOTION Exercise:Exercise - 3 ( Section-B )
Explore 24 Videos
- ROTATIONAL MOTION Book:MOTIONChapter:ROTATIONAL MOTION Exercise:Exercise - 1
Explore 90 Videos
- RAY OPTICSBook:MOTIONChapter:RAY OPTICSExercise:Exercise-4
Explore 38 Videos
- SEMI CONDUCTOR AND LOGIC GATESBook:MOTIONChapter:SEMI CONDUCTOR AND LOGIC GATESExercise:EXERCISE 3
Explore 34 Videos
Similar Questions
A solid sphere of mass M and radius R having moment of inertial I about its diameter is recast into a solid disc of radius r and thickness t .The moment of inertia of the disc about an axis passing the edge and perpendicular to the plane remains "I" .Then r=x√15R Find x=?
View SolutionThe moment of inertia of a then circular disc about an axis passing through its centre and perpendicular to its plane is I. Then, the moment of inertia of the disc about an axis parallel to its diameter and touching the edge of the rim is
View Solution
Knowledge Check
The radius of gyration of a disc about an axis coinciding with a tangent in its plane is
AR2BR√2C√2RD√52RMoment of inertia of a uniform circular disc about a diameter is I. Its moment of inertia about an axis perpendicular to its plane and passing through a point on its rim will be.
A6 IB4 IC2 ID8 IThe moment of inertia of a uniform circular disc of mass M and radius R about any of its diameters is 14MR2. What is the moment of inertia of the disc about an axis passing through its centre and normal to the disc?
AMR2B12MR2C32MR2D2MR2
The radius of gyration of a disc of radius 25 cm about a centroidal axis perpendicular to disc is
View SolutionFrom a circular disc of radius R and mass 9M, a small disc of radius R/3 is removed from the disc. The moment of inertia of the remaining disc about an axis perpendicular to the plane of the disc and passing through O is

View SolutionThe ratio of the radii of gyration of a circular disc about a tangential axis in the plane of the disc and a circular ring of the same radius about a tengential axis in the plane of the ring is
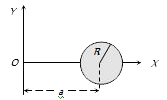
View SolutionThe adjoining figure shows a disc of mass M and radius R lying in the X-Y plane with its centre on X - axis at a distance a from the origin. Then the moment of inertia of the disc about the X-axis is
View SolutionForm a circular disc of radius R and mass 9M , a small disc of mass M and radius R/3 is removed concentrically . The moment of inertia of the remaining disc about an axis perpendicular to the the plane of the disc and passing its centre is :-
View Solution