A thin hollow cylinder
slides without rotating with a speed v.
Find the ratio of kinetic energies in the two cases.
To solve the problem of finding the ratio of kinetic energies in the two cases for a thin hollow cylinder sliding without rotating, we will break it down into clear steps.
1. Understanding the Kinetic Energy in Translational Motion:
- The kinetic energy (KE) of a body in translational motion is given by the formula:
KEtranslational=12mv2
- Here, m is the mass of the cylinder and v is its velocity.
2. Understanding the Kinetic Energy in Rotational Motion:
- The kinetic energy of a body in rotational motion is given by the formula:
KErotational=12Iω2
- Where I is the moment of inertia and ω is the angular velocity.
3. Moment of Inertia of a Thin Hollow Cylinder:
- The moment of inertia I for a thin hollow cylinder about its central axis is given by:
I=mr2
- Here, r is the radius of the cylinder.
4. Relating Linear Velocity and Angular Velocity:
- For a cylinder rolling without slipping, the relationship between linear velocity v and angular velocity ω is:
v=rω⟹ω=vr
5. Substituting Angular Velocity into Rotational Kinetic Energy:
- Now, substituting ω in the rotational kinetic energy formula:
KErotational=12I(vr)2=12(mr2)(v2r2)=12mv2
6. Finding the Ratio of Kinetic Energies:
- Now we can find the ratio of the translational kinetic energy to the rotational kinetic energy:
Ratio=KEtranslationalKErotational=12mv212mv2=1
Final Answer
The ratio of kinetic energies in the two cases (translational to rotational) is:
Ratio=1
- The kinetic energy (KE) of a body in translational motion is given by the formula:
KEtranslational=12mv2
- Here, m is the mass of the cylinder and v is its velocity.
- The kinetic energy of a body in rotational motion is given by the formula:
KErotational=12Iω2
- Where I is the moment of inertia and ω is the angular velocity.
- The moment of inertia I for a thin hollow cylinder about its central axis is given by:
I=mr2
- Here, r is the radius of the cylinder.
- For a cylinder rolling without slipping, the relationship between linear velocity v and angular velocity ω is:
v=rω⟹ω=vr
- Now, substituting ω in the rotational kinetic energy formula:
KErotational=12I(vr)2=12(mr2)(v2r2)=12mv2
- Now we can find the ratio of the translational kinetic energy to the rotational kinetic energy:
Ratio=KEtranslationalKErotational=12mv212mv2=1
The ratio of kinetic energies in the two cases (translational to rotational) is:
Ratio=1
Topper's Solved these Questions
- ROTATIONAL MOTION BOOK - MOTIONCHAPTER - ROTATIONAL MOTION EXERCISE - Exercise - 1
90 Videos
- ROTATIONAL MOTION BOOK - MOTIONCHAPTER - ROTATIONAL MOTION EXERCISE - Exercise - 2
40 Videos
- RAY OPTICSBOOK - MOTIONCHAPTER - RAY OPTICSEXERCISE - Exercise-4
38 Videos
- SEMI CONDUCTOR AND LOGIC GATESBOOK - MOTIONCHAPTER - SEMI CONDUCTOR AND LOGIC GATESEXERCISE - EXERCISE 3
34 Videos
Similar Questions
A thin hollow cylinder
rolls with the same speed without slipping.
Find the ratio of kinetic energies in the two cases.
View SolutionA hollow cylinder open at both ends slides without rotating, and then rolls without slipping with the same speed. The ratio of the kinetic energy in the two cases is (taken in order)
View Solution
Knowledge Check
A thin hollow cylinder open at both ends
(i) Slides without rotating
(ii) Rolls without slipping, with the same speed.
The ratio of kinetic energies in the two cases isA1:1B4:1C1:2D2:1A hollow cylinder open at both ends slides without rotating, and then rolls without slipping with the same speed. The ratio of the kinetic energy in the two cases is (taken in order)
A1:1B4:1C1:2D2:1A hollow sphere rolls without slipping on plane surface. The ratio of kinetic energy of rotation to the total kinetic energy is
A1/3B2/5C1/4D3/4
Portion AB of the wedge shown in figure is rough and BC is smooth. A solid cylinder rolld without slipping from A to B. Find the ratio of translational kinetic energy to rotationa linetic energy, when the cylinder reaches point C.
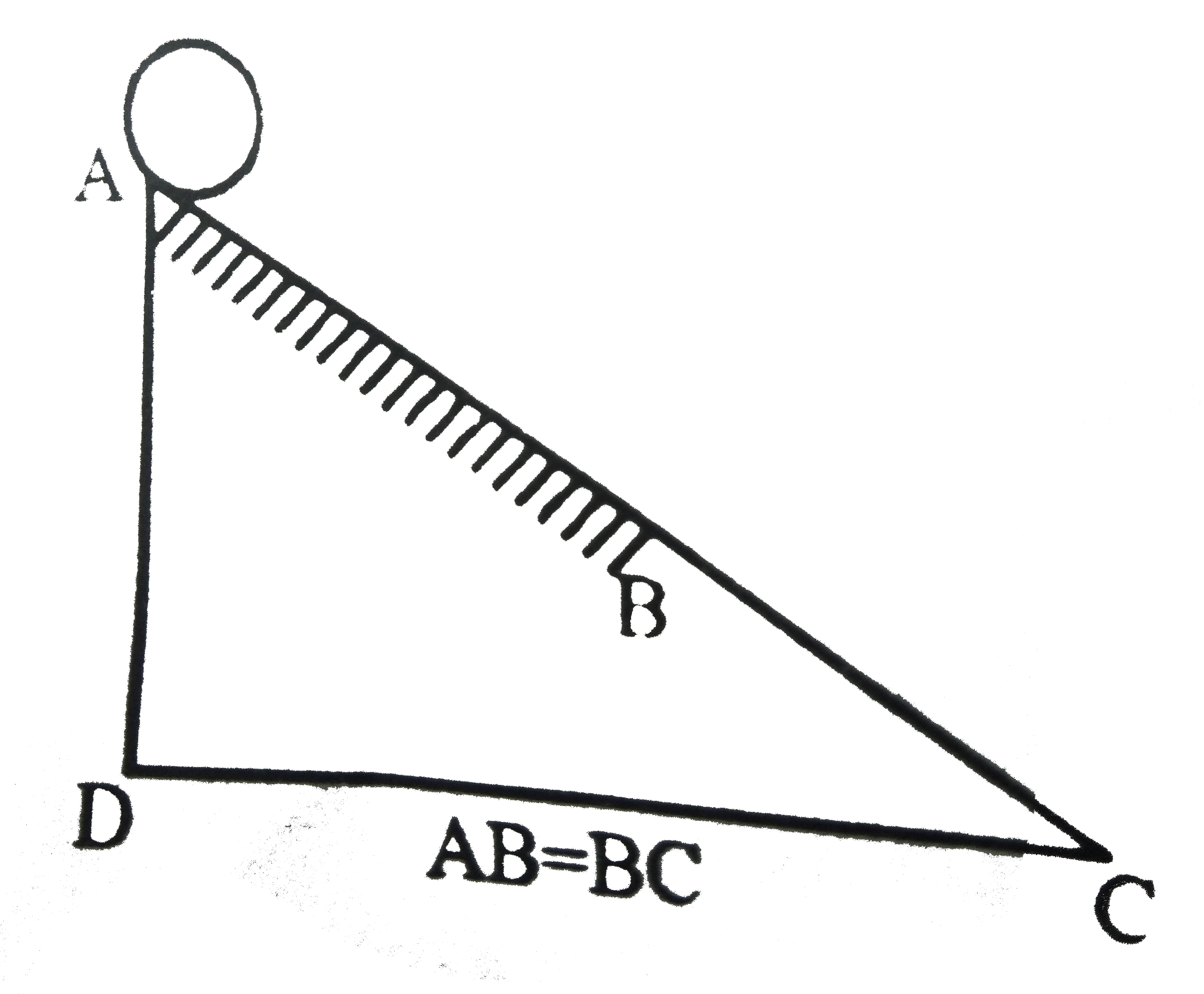 .
.
View SolutionWhen a solid cylinder rolls without slipping the ratio of kinetic energy of translation to its total kinetic energy is
View SolutionA cylinder has a mixture of hydrogen and oxygen gases in the ratio 1:3. the ratio of mean kinetic energies of the two gases is :
View SolutionA thin hollow cylinder, open at both ends and weighing 5kg (a) slides with a speed of 10m/s without rotating (b) rolls with the same speed without slipping. Compare the kinetic energies of the cylinder in the two cases.
View SolutionA hollow sphere (i) rolls and (ii) slides down an inclined plane. The ratio of the accelerations in the two cases is
View Solution
