A uniform wire of length l and mass M is bent in the shape of a semicircle of radius r as shown in figure. Calculate moment of intertia about the axis XX'
To find the moment of inertia of a uniform wire bent into the shape of a semicircle about the axis XX', we can follow these steps:
Step 1: Understand the Geometry
The wire has a total length L and is bent into a semicircular shape with radius r. The mass of the wire is M.
Step 2: Relate Length and Radius
Since the wire forms a semicircle, the length of the wire can be expressed as:
L=πr
From this, we can express the radius r in terms of the length L:
r=Lπ
Step 3: Consider the Complete Circle
If we consider a complete circle made from the wire, its mass would be 2M (since the semicircle is half of a complete circle). The moment of inertia Iz of a complete circular ring about an axis perpendicular to its plane (through its center) is given by:
Iz=Mr2
For the complete circle, substituting M with 2M:
Iz=2Mr2
Step 4: Apply the Perpendicular Axis Theorem
According to the perpendicular axis theorem, for a planar object:
Iz=Ixx′+Iyy′
Since the semicircle is symmetrical about the axis XX' and YY', we have:
Ixx′=Iyy′
Thus, we can write:
Iz=2Ixx′
From this, we can solve for Ixx′:
Ixx′=Iz2
Step 5: Calculate Moment of Inertia for the Complete Circle
Substituting Iz into the equation:
Ixx′=2Mr22=Mr2
Step 6: Substitute the Radius
Now, substituting r=Lπ into the moment of inertia:
Ixx′=M(Lπ)2=ML2π2
Final Result
Thus, the moment of inertia of the semicircular wire about the axis XX' is:
Ixx′=ML22π2
The wire has a total length L and is bent into a semicircular shape with radius r. The mass of the wire is M.
Since the wire forms a semicircle, the length of the wire can be expressed as:
L=πr
From this, we can express the radius r in terms of the length L:
r=Lπ
If we consider a complete circle made from the wire, its mass would be 2M (since the semicircle is half of a complete circle). The moment of inertia Iz of a complete circular ring about an axis perpendicular to its plane (through its center) is given by:
Iz=Mr2
For the complete circle, substituting M with 2M:
Iz=2Mr2
According to the perpendicular axis theorem, for a planar object:
Iz=Ixx′+Iyy′
Since the semicircle is symmetrical about the axis XX' and YY', we have:
Ixx′=Iyy′
Thus, we can write:
Iz=2Ixx′
From this, we can solve for Ixx′:
Ixx′=Iz2
Substituting Iz into the equation:
Ixx′=2Mr22=Mr2
Now, substituting r=Lπ into the moment of inertia:
Ixx′=M(Lπ)2=ML2π2
Thus, the moment of inertia of the semicircular wire about the axis XX' is:
Ixx′=ML22π2
Topper's Solved these Questions
- ROTATIONAL MOTION Book:MOTIONChapter:ROTATIONAL MOTION Exercise:Exercise - 1
Explore 90 Videos
- ROTATIONAL MOTION Book:MOTIONChapter:ROTATIONAL MOTION Exercise:Exercise - 2
Explore 40 Videos
- RAY OPTICSBook:MOTIONChapter:RAY OPTICSExercise:Exercise-4
Explore 38 Videos
- SEMI CONDUCTOR AND LOGIC GATESBook:MOTIONChapter:SEMI CONDUCTOR AND LOGIC GATESExercise:EXERCISE 3
Explore 34 Videos
Similar Questions
Knowledge Check
A thin wire of length l and mass m is bent in the form of a semicircle as shown in the figure. Its moment of inertia about an axis joining its free ends will be
 Aml2BzeroCml2π2Dml22π2
Aml2BzeroCml2π2Dml22π2A thin rod of length L of mass M is bent at the middle point O at an angle of 60∘. The moment of inertia of the rod about an axis passing through O and perpendicular to the plane of the rod will be
 AML26BML212CML224DML23
AML26BML212CML224DML23
A thin rod of length 4l, mass 4 m is bent at the point as shown in the figure. What is the moment of inertia of the rod about the axis passing through O and perpendicular to the plane of the paper?AMl23B10Ml23CMl212DMl224
A wire of length l and mass m is bent in the form of a semicircle. The gravitational field intensity at the centre of semicircle is

View SolutionA uniform rod of mass 2M is bent into four adjacent semicircles each of radius r, all lying in the same plane. The moment of inertia of the bent rod about an axis through one end A and perpendicular to plane of rod is

View SolutionA rod of lengthL and mass M0 is bent to form a semicircular ring as shown. The M.I. about X'X is

View SolutionA uniform wire of length l is bent into the shape of 'V' as shown. The distance of its centre of mass from the vertex A is :-
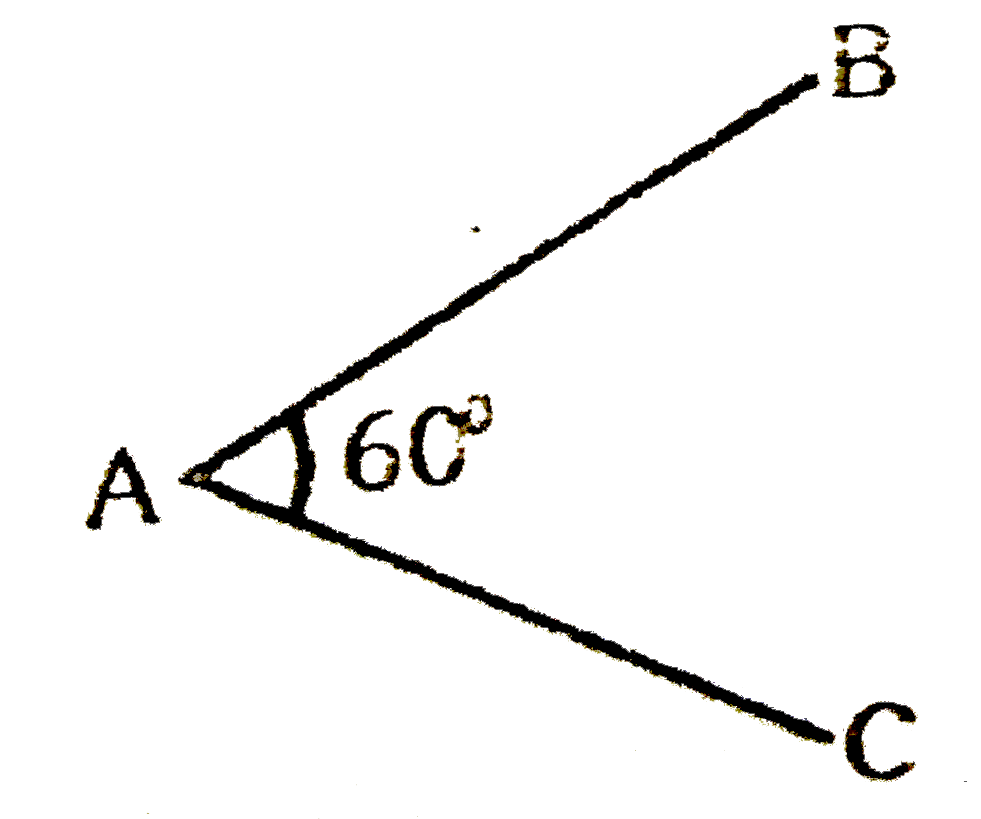
View Solution
A thin rod of length 4l, mass 4 m is bent at the point as shown in the figure. What is the moment of inertia of the rod about the axis passing through O and perpendicular to the plane of the paper?
View Solution