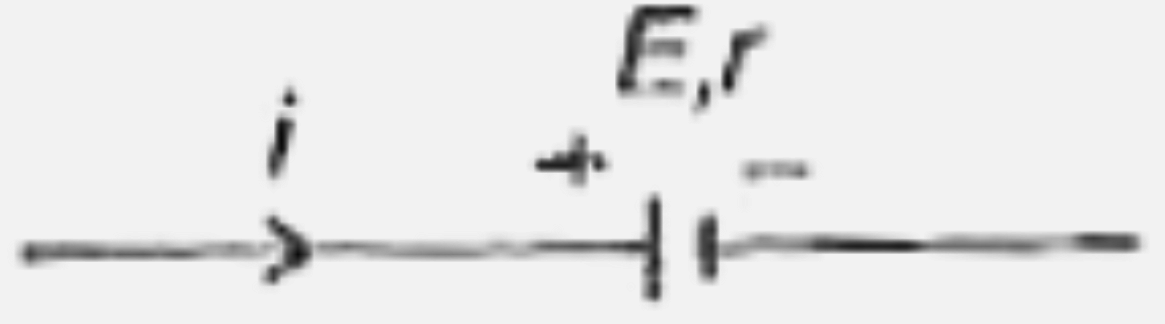If a cell of constant E.M.F. produces the same amount of the heat during the same time in two independent resistors R1 and R2, when they are separately connected across the terminals of the cell, one after the another, find the internal resistance of the cell.
√R1R2
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - MOTIONCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE -3 (Level -I) SECTION E- Electrical Power & Energy
15 Videos
- CURRENT ELECTRICITYBOOK - MOTIONCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE -3 (Level -II) SECTION A- Definition of Current , Current Density , Drift Velocity
1 Video
- CURRENT ELECTRICITYBOOK - MOTIONCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE -3 (Level -I) SECTION B- Resistance & resistivity ,Ohms law +VI (Volt -Curr.) Characteristics +Colour Code + Temp . Dependence
8 Videos
- CONSTRAINED MOTION BOOK - MOTIONCHAPTER - CONSTRAINED MOTION EXERCISE - EXAMPLES
12 Videos
- ELASTICITYBOOK - MOTIONCHAPTER - ELASTICITYEXERCISE - EXERCISE -3
60 Videos
Similar Questions
Knowledge Check
v31
A2(E−V)VrB2(E−V)rEC(E−V)rVD(E−V)rNull point in the galvanometer is obtained when a cell of emf E and internal resisance r is connncted across the length of 22 cm wire of the potentiometer . Now a resistane of 10Ω is connected across the terminals of the cell (by closing the key K) and null point is obtained against the length of cm. The internal resistance r of the cell is -
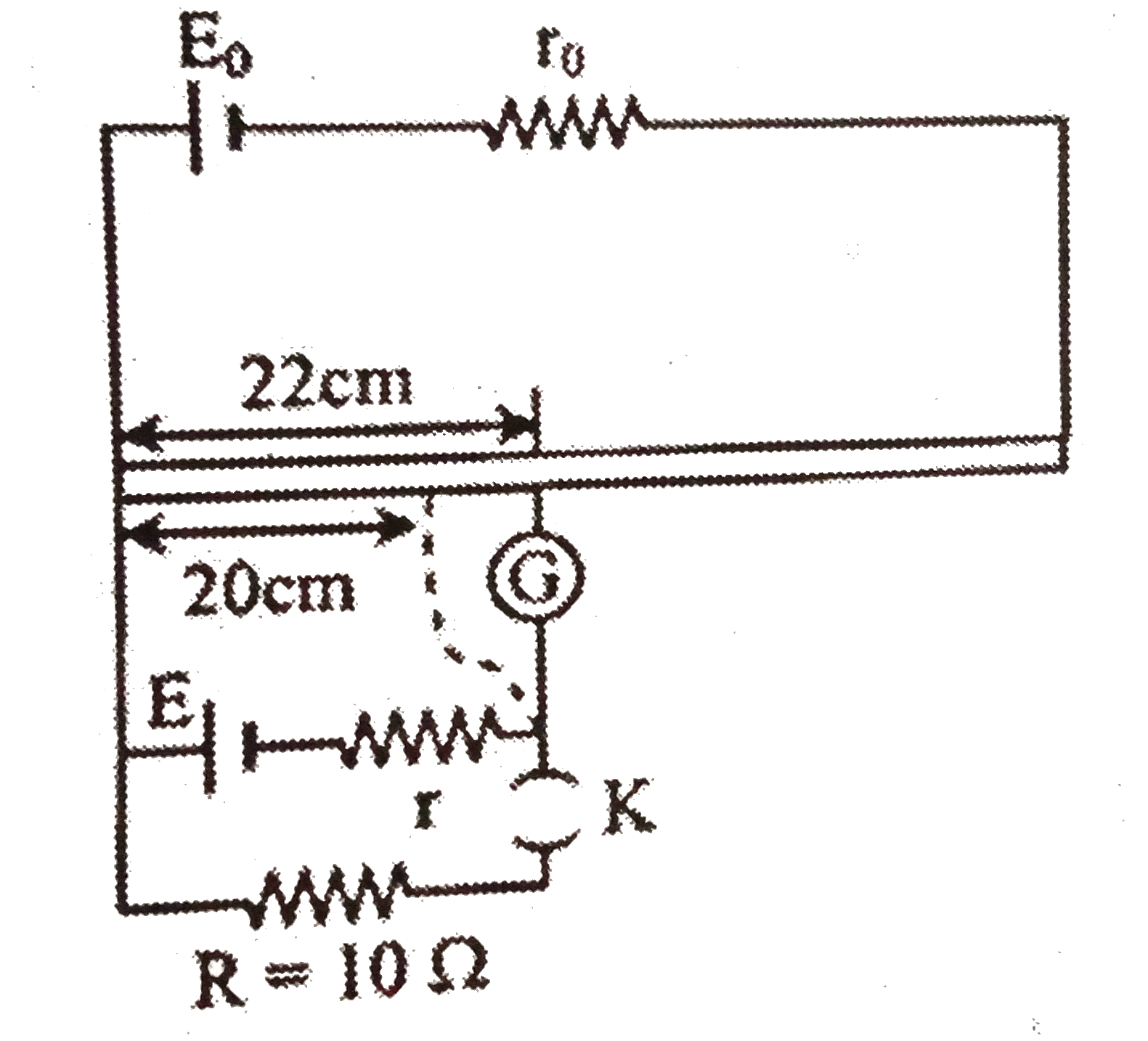 A0.5ΩB1ΩC1.5ΩD2Ω
A0.5ΩB1ΩC1.5ΩD2ΩA cell of e.m.f E is connected to a resistance R1 for time i and the amount of heat generated in it is H. If the resistance R1 is replaced by another resistance R2 and is connected to the cell for the same time i, the amount of heat generated in R2 is 4H. Then the internal resistance of the cell is
A2R1+R22B√R1R22√R2−√R1√R2−2√R1C√R1R2√R2−2√R12√R2−√R1D√R1R2√R2−2√R12√R2+√R1
A cell of emf 'E' is connected across a resistance R.The potential difference between the terminals of the cell is found to be "V" .The internal resistance of the cell must be:
View SolutionThe reading on a high resistance voltmeter. When a cell is connected across it is 2.2V. When the terminals of the cell are connected to a resistance of 5Ω the voltmeter reading drop to 1.8V. Find the internal resistance of the cell.
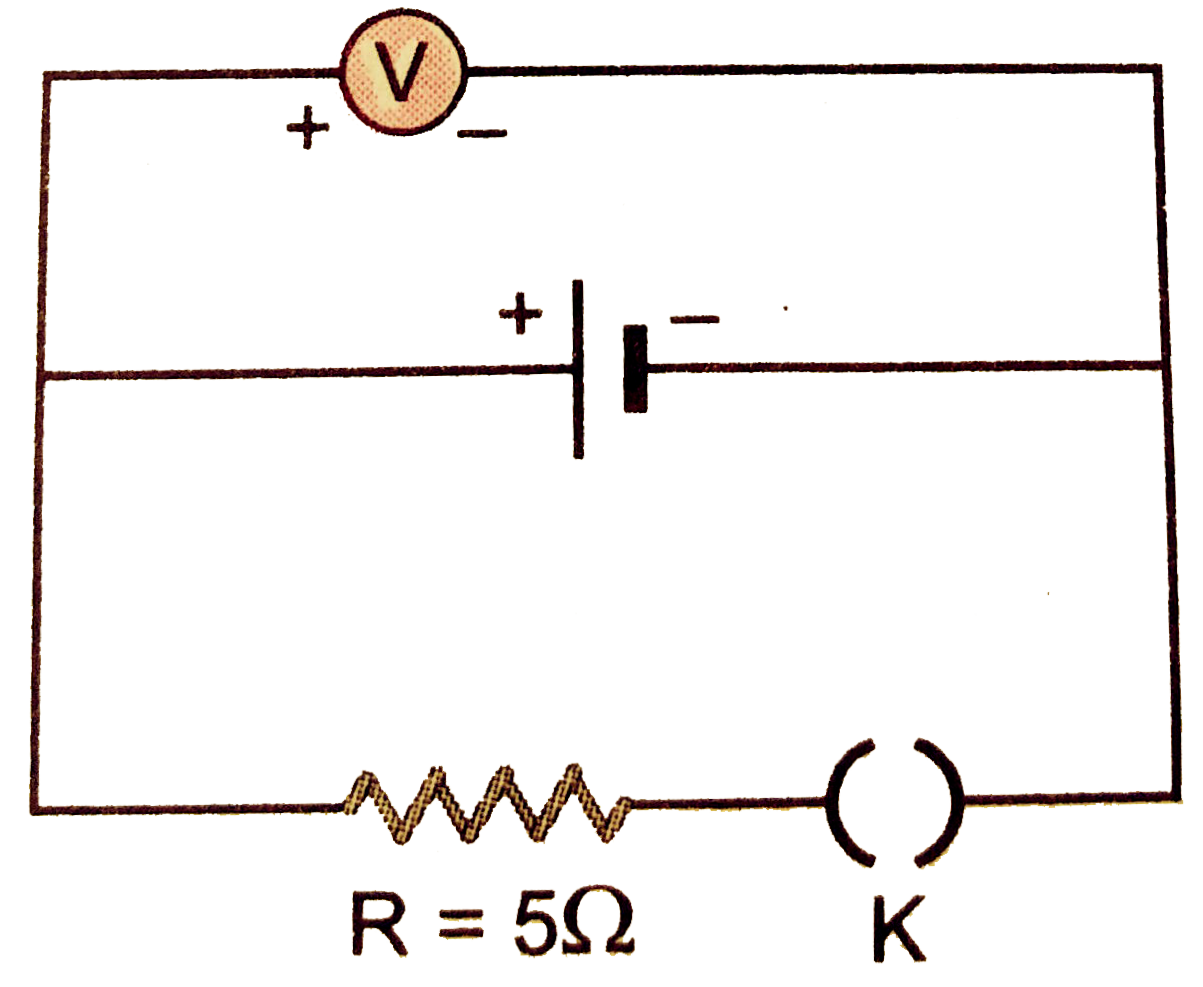
View SolutionThe reading on a high resistance voltmeter when a cell is connected across it is 3 V. When the terminals of the cell are also connected to a resistance of 4 Ω, then the voltmeter reading drops to 1.2 V. Find the internal resistance of the cell.
View SolutionA cell develops the same power across two resistances R1 and R2 separately. The internal resistance of the cell is
View SolutionA cell of constant emf first connected to a resistance R1 and then connected to resistance R2. If power deliverd in both cases is same, then the internal resistance of the cell is
View Solution