and are two vectors in a plane at an angle of with each other. is another vector perpendicular to the plane containing vectors . Which of the following relations is possible ?
The correct Answer is:C
If two vectors are added to get a third vector, then all three vectors will lie on the same plane. However, cross product of two vectors is perpendicular to the plane containing those two vectors.
Similar Questions
Knowledge Check
A and B are two vectors in a plane at an angle of 60∘ with each other. C is another vector perpendicular to the plane containing vector A and B. Which of the following releations is possible?
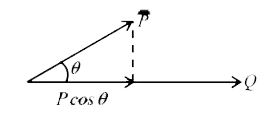
AA+B=CBA+C=BCA×B=CDA×C=BProjection of →P on →Q is :
A→P.ˆQB→P.ˆQC→P׈QD→P׈QLet →C=→A+→B then :
A∣∣→C∣∣ is always greater then ∣∣→A∣∣ and ∣∣→C∣∣<∣∣→B∣∣Bit is possible to haveC∣∣→C∣∣ is always equal to ∣∣→A+→B∣∣D∣∣→C∣∣ is never equal to ∣∣→A+→B∣∣
The magnitude of the vector product of two vectors and may be : →A and →B
View SolutionIf →A and →B are two vectors of non-zero magnitude, which of the following relations cannot be possible ?
View SolutionConsider a vector →F=4ˆi−3ˆj. Another vector that is perpendicular to →F is
View SolutionDot product of two vectors →A and →B is defined as →A.→B=aBcosϕ , where ϕ is angle between them when they are drawn with tails coinciding. For any two vectors . This means ovsert(→)A.→B=→B.→A that . The scalar product obeys the commutative law of multiplication, the order of the two vectors does not matter. The vector product of two vectors →A and →B also called the cross product, is denoted by →A×→B . As the name suggests, the vector product is itself a vector. →C=→A×→B then C=ABsinθ ,
For non zero vectors →A,→B,→C,∣∣(→A×→B).→C∣∣=∣∣→A∣∣∣∣→B∣∣∣∣→C∣∣ holds if and only if
View SolutionIf →A+→B+→C =0 and A = B + C, the angle between →A and →B is :
View Solution