A förce of6 N and another of 8 N can be applied together to produce the effect of asingle force oF (A) I N (B) 11 N (C) 15 N (D) 20 N
1 N
11 N
15 N
20 N
The correct Answer is:b
The magnitude of resultant lies between (8 – 6)N (if they are in opposites direction) and (8 + 6)N (When they are in same direction)
Similar Questions
A force of 6N and another of 8N can be applied together to produce the effect of a single force of
View SolutionForces of magnitudes 6N and 4N are acting on the body . Which of the following of the following can be the resultant of the two?
(a) 11N (b) 2N (c) 10N (d) 1N (e) 8N (f) 0N (g) 7N
View Solution
Knowledge Check
A force of 60 N acting perpendicular to a force of 80 N can produce
A20 NB70 NC100 ND140 NIf 4M37094267N is divisible by both 8 and 11, where M and N are single digit integers, then the values of M and N are :
A)M=5, N=6
B)M=5, N=4
C)M=5, N=2
D)M=2, N=5AM=5, N=6BM=5, N=4CM=5, N=2DM=2, N=5Forces are applied on a wheel of radius 20 cm as shown in the figure. The torque produced by the forces 4 N at A, 8N at B, 6 N at C and 9N at D at angles indicated is
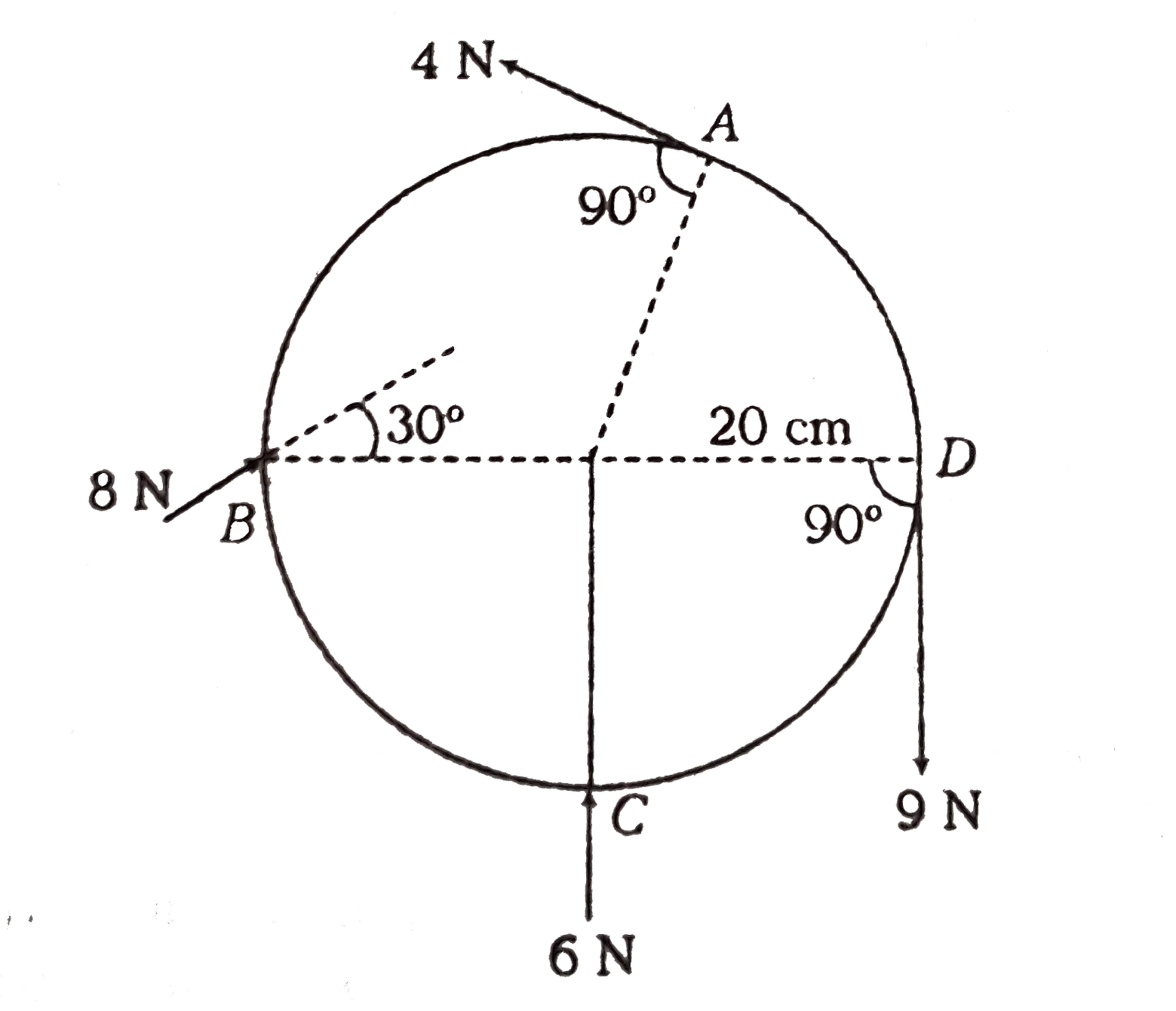 A5.4 N-m anti-clockwiseB1.80 N-m clockwiseC2.0 N-m clockwiseD3.6 N-m clockwise
A5.4 N-m anti-clockwiseB1.80 N-m clockwiseC2.0 N-m clockwiseD3.6 N-m clockwise
To make two bodies A and B experience an equal acceleration, forces 6 N and 4 N are applied, respectively. If the bodies are combined, then to produce the same acceleration, the force applied should be _______ N.
View SolutionA mechanic can loosen a nut by applying a 150-N force using a spanner of length 40 cm. What is the length of the spanner if he wants to loosen the nut by applying a force of 50 N to produce the same turning effect?
View SolutionWhat is the first value of n for which n2+n+41 is not a prime? 1 (b) 10 (c) 20 (d) 40
View SolutionA wheel of radius 20 cm has forces applied to it as shown in the figure. The torque produced by the forces 4N at A, 8N at B, 6N at C, and 9N at D at angles indicated is :
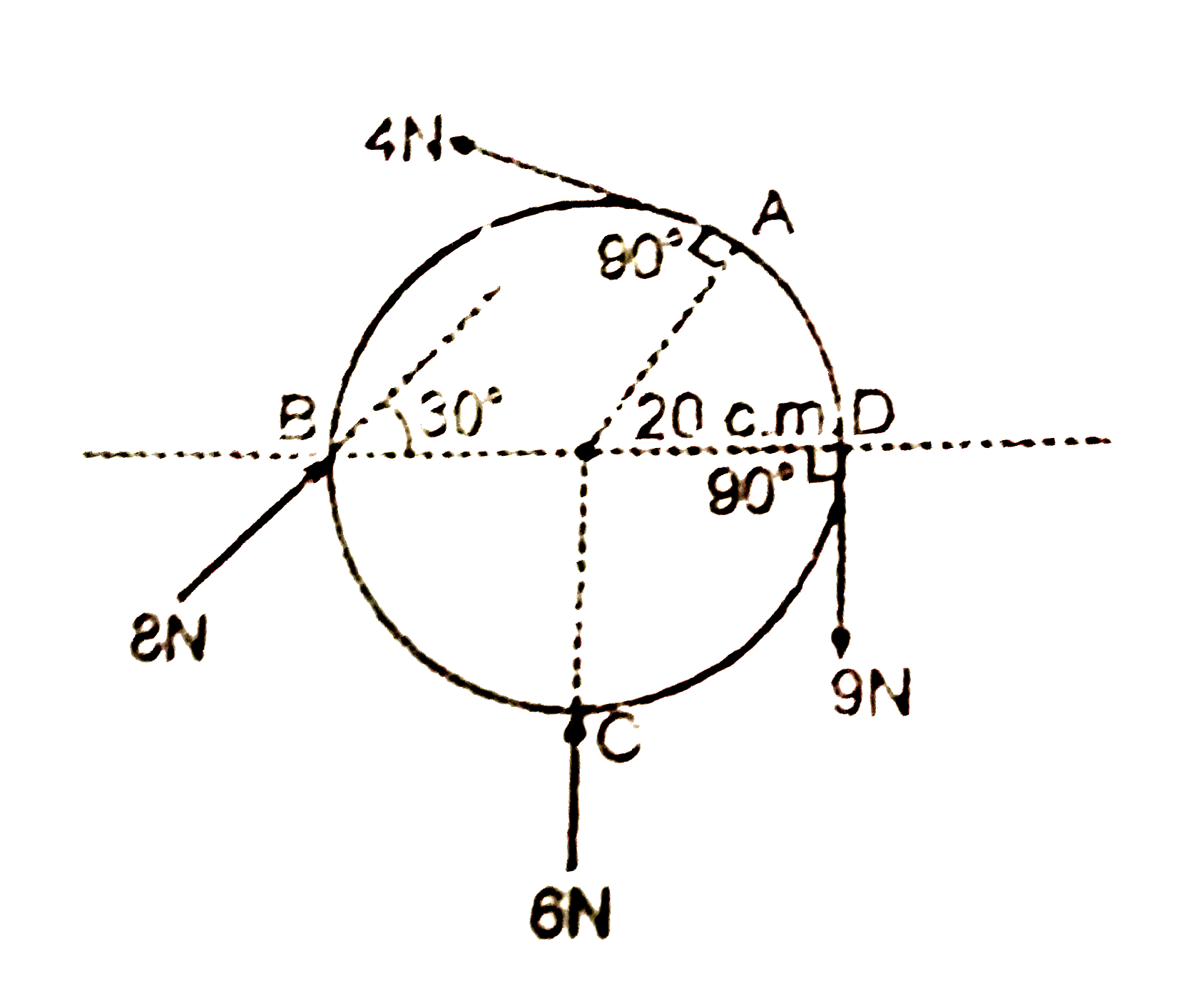
View SolutionIf n(A)=6 and n(B)=8 and n(A∩B)=4, then find n(AΔB).
The following are the step involved in solving the above problem . Arrange them in sequnential order.
(a) n(A∪B)=n(A)+n(B)−n(A∩B)
(b) n(A∪B)=10
(c )n(A∪B)=6+8−4
(d) n(AΔB)=10−4=6
(e) n(AΔB)=n(A∪B)−n(A∩B).
View Solution
