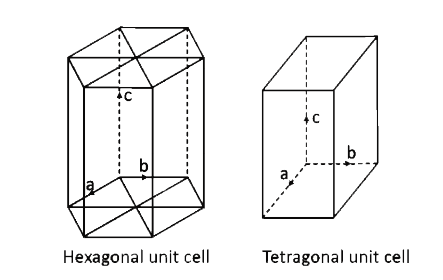
The ratio of the volume of a tetragonal lattice unit cell to that of a hexagonal lattice unit cell is (both having same respective lengths)
A
√32abc
B
22√3
C
2√3a2b c
D
1
Video Solution
Text Solution
Ratio=abc6×(√34a2)×c=23√3
Note : ca=2√2√3 for an ideal HPC (see illustration
|
Similar Questions
Knowledge Check
The ratio of the volume of a tetragonal lattice unit cell to that of a hexagonal lattice unit cell is (both having same respective lengths)
A2√3B√32abcC√3a22bcD(2a2c)√3bIn a Tetragonal unit cell :
Aa = b = c, α=β=γ≠90∘Ba = b ne c, α=β=γ=90∘Ca = b = c, α=β=γ=90∘Da = b ne c, α=β=90∘,γ=120∘In a tetragonal unit cell:
Aa = b = c, α=β=γ≠90∘Ba = b ne c, α=β=γ=90∘Ca = b = c, α=β=γ=90∘Da = b ne c, α=β=90∘,γ=120∘
