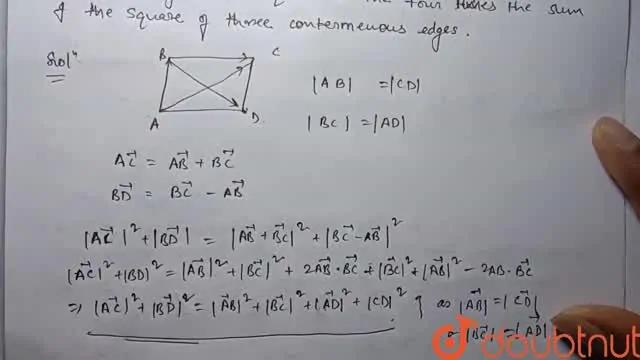
Prove that in a parallelogram, the sum of the squres of the diagonals is equal otthe four times the sum of the square of three conterminos edges.

Video Solution

Text Solution

Generated By DoubtnutGPT

To prove that in a parallelogram, the sum of the squares of the diagonals is equal to four times the sum of the squares of three contiguous edges, we can follow these steps:
Step 1: Define the Parallelogram
Let $A B C D$ be a parallelogram. The diagonals are $A C$ and $B D$ .
Step 2: Express the Diagonals in Vector Form
Using vector notation, we can express the diagonals as:
- $\to A C = \to A B + \to B C$
- $\to B D = \to B C - \to A B$
Step 3: Calculate the Squares of the Diagonals
Now, we calculate the squares of the diagonals:
1. For diagonal $A C$ :
$| \to A C |^{2} = | \to A B + \to B C |^{2} = | \to A B |^{2} + | \to B C |^{2} + 2 \to A B \cdot \to B C$
2. For diagonal $B D$ :
$| \to B D |^{2} = | \to B C - \to A B |^{2} = | \to B C |^{2} + | \to A B |^{2} - 2 \to A B \cdot \to B C$
Step 4: Sum the Squares of the Diagonals
Now, we sum the squares of the diagonals:
$| \to A C |^{2} + | \to B D |^{2} = (| \to A B |^{2} + | \to B C |^{2} + 2 \to A B \cdot \to B C) + (| \to B C |^{2} + | \to A B |^{2} - 2 \to A B \cdot \to B C)$
This simplifies to:
$| \to A C |^{2} + | \to B D |^{2} = 2 | \to A B |^{2} + 2 | \to B C |^{2}$
Step 5: Relate to the Contiguous Edges
In a parallelogram, the opposite sides are equal:
- $| \to A B | = | \to C D |$
- $| \to B C | = | \to A D |$
Thus, the sum of the squares of three contiguous edges $| \to A B |^{2} + | \to B C |^{2} + | \to A D |^{2}$ can be expressed as:
$| \to A B |^{2} + | \to B C |^{2} + | \to C D |^{2} = | \to A B |^{2} + | \to B C |^{2} + | \to A B |^{2} = 2 | \to A B |^{2} + | \to B C |^{2}$
Step 6: Final Equation
Now, we can relate the sum of the squares of the diagonals to four times the sum of the squares of the three contiguous edges:
$| \to A C |^{2} + | \to B D |^{2} = 2 | \to A B |^{2} + 2 | \to B C |^{2} = 4 (\frac{1}{2} (| \to A B |^{2} + | \to B C |^{2} + | \to A D |^{2}))$
Thus, we have proved that:
$| \to A C |^{2} + | \to B D |^{2} = 4 (| \to A B |^{2} + | \to B C |^{2} + | \to A D |^{2})$
Conclusion
Therefore, the sum of the squares of the diagonals in a parallelogram is equal to four times the sum of the squares of three contiguous edges.

|

Class 12 MATHS VECTOR ALGEBRA

Similar Questions

Knowledge Check

In a parallelopiped the ratio of the sum of the squares on the four diagonals to the sum of the squares on the three coterminous edges is
A2
B3
C4
D1

Recommended Questions

Home
Ask Doubt
Profile