If the graph of the function has a unique tangent at point (not vertical, non-horizontal) on the graph, then evaluate .
The correct Answer is:4
Step by step video, text & image solution for If the graph of the function y=f(x) has a unique tangent at point (e^(a),0) (not vertical, non-horizontal) on the graph, then evaluate lim_(xtoe^(a))((1 +9f(x))-"tan"(f(x)))/(2f(x)). by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
If the graph of the function y=f(x) is as shown :

The graph of y=1/2(|f(x)|−f(x)) isA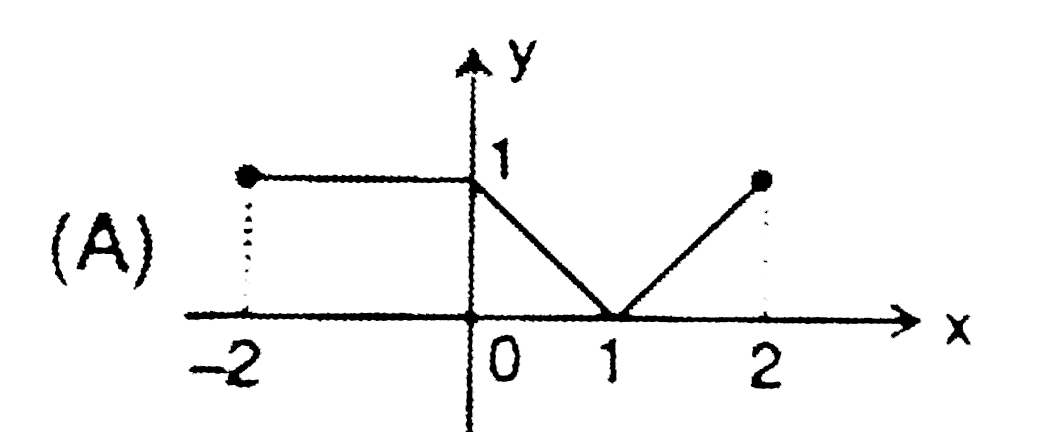 B
B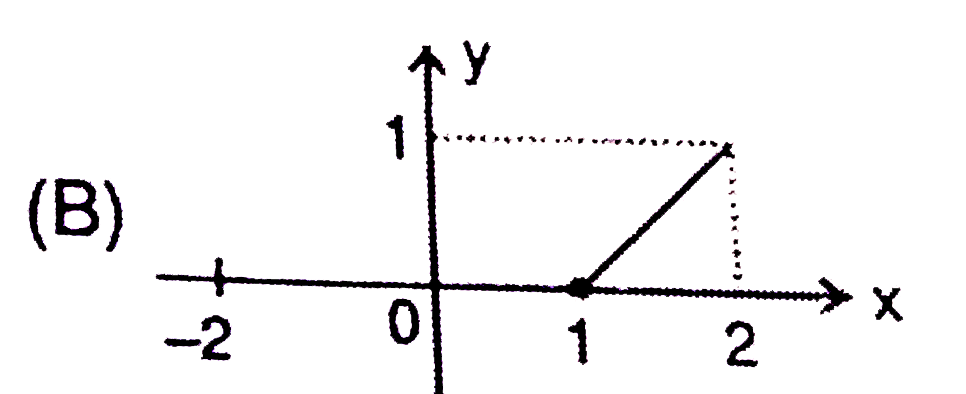 C
C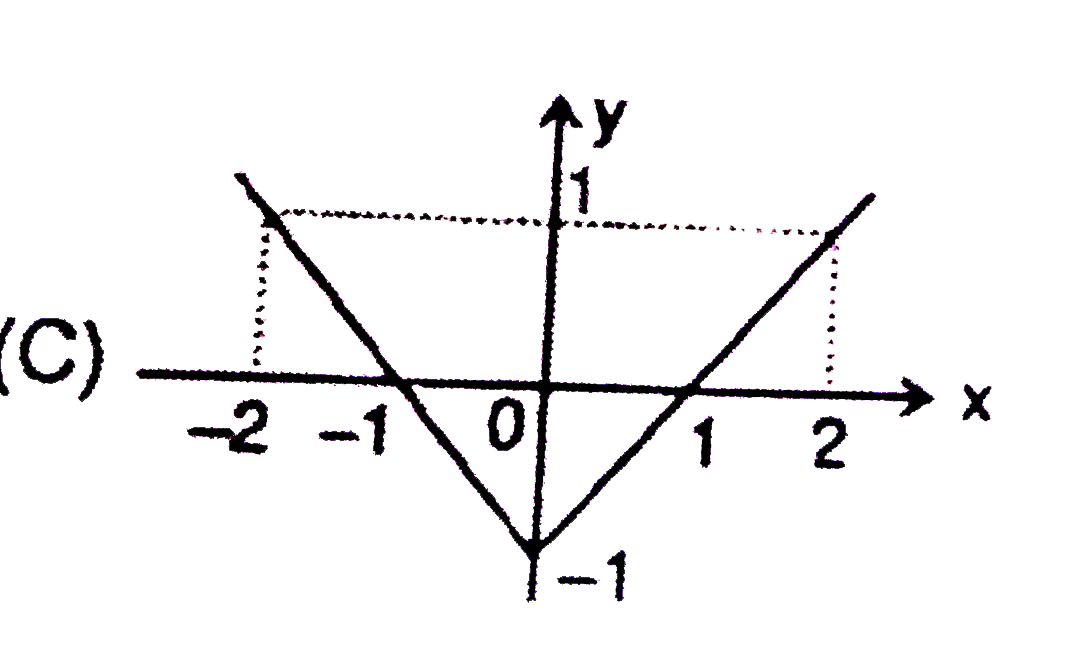 D
D
The graph of the function y=f(x) has a unique tangent at the point (ea,0) through which the graph passes then lim–––––(x→ea)loge{1+7f(x)}−sinf(x)3f(x)
View SolutionThe graph of the function y=f(x) has a unique tangent at the point (a, 0) through which the graph passes then, limx→a(ln(1+6f(x))3f(x)
View SolutionIf the graph of the function y=f(x) is as shown : the graph of y=12(|f(x)|−f(x)) is
View SolutionIf f'(2)=4 then, evaluate limx→0f(1+cosx)−f(2)tan2x
View SolutionStatement-1: If the graph of the function y=f(x) has a unique tangent at the point (a,0) through which limx→aloge(1+6(f(x)))3f(x)=2 Statement-2: Since the graph passes through (a,0). Therefore f(a)=0. When f(a)=0 given limit iszero by zero form so that it can be evaluate by using L-Hospital's rule.
View SolutionA horizontal line meets the graph of the function y=f(x) in two points is f one one?
View SolutionPlot the graph of the function f(x)=limt→0(2xπtan−1xt2)
View Solution
