Let ABCD be a square of side length 2 units. C2 is the circle through vertices A, B, C, D and C1 is the circle touching all the sides of the square ABCD. L is a line through A. 27. If P is a point on C1 and Q in another point on C2, then 2222 2222 PA PB PC PD QA QB QC QD +++ +++ is equal to (A) 0.75 (B) 1.25 (C) 1 (D) 0.5
0.75
1.25
1
0.5
The correct Answer is:A
Step by step video & text solution for Let ABCD be a square of side length 2 units. C2 is the circle through vertices A, B, C, D and C1 is the circle touching all the sides of the square ABCD. L is a line through A. 27. If P is a point on C1 and Q in another point on C2, then 2222 2222 PA PB PC PD QA QB QC QD +++ +++ is equal to (A) 0.75 (B) 1.25 (C) 1 (D) 0.5 by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
माना ABCD एक वर्ग है जिसकी भुजा की लम्बाई 2 इकाई है। वृत्त C2 शीर्ष A, B, C, D से गुजरता है तथा वृत्त C1 वर्ग ABCD की सभी भुजाओं को स्पर्श करता है। L, A से गुजरने वाली एक रेखा है।
यदि P,C1 पर एक बिन्दु है तथा Q,C2 पर दूसरा बिन्दु है, तो PA2+PB2+PC2+PD2QA2+QB2+QC2+QD2 है-
View Solutionमाना ABCD एक वर्ग है जिसकी भुजा की लम्बाई 2 इकाई है। वृत्त C2 शीर्ष A, B, C, D से गुजरता है तथा वृत्त C1 वर्ग ABCD की सभी भुजाओं को स्पर्श करता है। L, A से गुजरने वाली एक रेखा है।
यदि P,C1 पर एक बिन्दु है तथा Q,C2 पर दूसरा बिन्दु है, तो PA2+PB2+PC2+PD2QA2+QB2+QC2+QD2 है-
View SolutionLet ABCD be a square of side length 2 units. Let C1 be the incircle and C2 be the circumcircle of the square ABCD. If P is a point on C1 and Q is a point on C2, the value of PA2+PB2+PC2+PD2QA2+QB2+QC2+QD2 is
View SolutionLet ABCD be a square of side length 2 units. C2 is the circle through vertices A, B, C, D and C1 is the circle touching all the sides of the square ABCD. L is a line through A. . If P is a point on C1 and Q in another point on C2, then (PA^2+ PB^2+ PC^2+PD^2)/ (QA^2+ QB^2+ QC^2+ QD^2) is equal to (A) 0.75 (B) 1.25 (C) 1 (D) 0.5
View SolutionIn the given figure, PA and PB are tangent to the circle from an external point P. CD is another tangent touching the circle at Q. If PA=12cm and QC=QD=3cm, then find PC+PD.
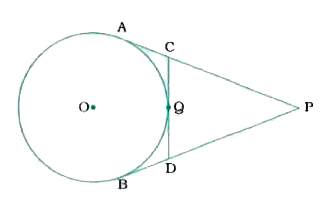
View SolutionC1 and C2 are two concentric circles, the radius of C2 being twice that of C1. From a point P on C2, tangents PA and PB are drawn to C1. Then the centroid of the triangle PAB (a) lies on C1 (b) lies outside C1 (c) lies inside C1 (d) may lie inside or outside C1 but never on C1
View SolutionConsider two curves C1:y^2=4x ; C2=x^2+y^2-6x+1=0 . Then, a. C1 and C2 touch each other at one point b. C1 and C2 touch each other exactly at two point c. C1 and C2 intersect(but do not touch) at exactly two point d. C1 and C2 neither intersect nor touch each other
View SolutionConsider two curves C1:y^2=4x ; C2=x^2+y^2-6x+1=0 . Then, (a) C1 and C2 touch each other at one point (b) C1 and C2 touch each other exactly at two point (c) C1 and C2 intersect(but do not touch) at exactly two point (d) C1 and C2 neither intersect nor touch each other
View Solution
