If the I.P. of Li+2is122.4 eV. Find out 6thI.P. of carbon -
122.4×4eV
122.4×2eV
122.4×3eV
122.4×5eV
The correct Answer is:A
To find the 6th ionization potential (I.P.) of carbon given the I.P. of Li+2 is 122.4 eV, we can follow these steps:
Step 1: Understand the Ionization Potential (I.P.)
The ionization potential (I.P.) is the energy required to remove an electron from an atom or ion in its gaseous state.
Step 2: Determine the Energy Levels
Using Bohr's model, the energy of the nth orbit for a hydrogen-like atom is given by:
\( E_n = -\frac{13.6 \, Z^2}{n^2} \, \text{eV} \)
where \( Z \) is the atomic number and \( n \) is the principal quantum number.
Step 3: Calculate the Energy for Li+2
For Li+2, the atomic number \( Z = 3 \). The first ionization potential corresponds to the transition from the first energy level (n=1) to infinity (n=∞):
- \( E_{\infty} = 0 \) eV (as n approaches infinity)
- \( E_1 = -\frac{13.6 \cdot 3^2}{1^2} = -\frac{13.6 \cdot 9}{1} = -122.4 \, \text{eV} \)
Thus, the first ionization energy (I.P.) is:
\( I.P. = E_{\infty} - E_1 = 0 - (-122.4) = 122.4 \, \text{eV} \)
Step 4: Determine the Electronic Configuration of Carbon
The electronic configuration of carbon (C) is \( 1s^2 \, 2s^2 \, 2p^2 \). When 5 electrons are removed, the configuration of \( C^{+5} \) will be \( 1s^1 \).
Step 5: Calculate the Energy for Carbon
For \( C^{+5} \) (where \( Z = 6 \)):
- The energy for the first level (n=1) is:
\( E_1 = -\frac{13.6 \cdot 6^2}{1^2} = -\frac{13.6 \cdot 36}{1} = -489.6 \, \text{eV} \)
Step 6: Calculate the 6th Ionization Potential
The 6th ionization potential corresponds to the energy required to remove the last electron from \( C^{+5} \):
\( I.P. = E_{\infty} - E_1 = 0 - (-489.6) = 489.6 \, \text{eV} \)
Final Answer
The 6th ionization potential of carbon is 489.6 eV.
---
The ionization potential (I.P.) is the energy required to remove an electron from an atom or ion in its gaseous state.
Using Bohr's model, the energy of the nth orbit for a hydrogen-like atom is given by:
\( E_n = -\frac{13.6 \, Z^2}{n^2} \, \text{eV} \)
where \( Z \) is the atomic number and \( n \) is the principal quantum number.
For Li+2, the atomic number \( Z = 3 \). The first ionization potential corresponds to the transition from the first energy level (n=1) to infinity (n=∞):
- \( E_{\infty} = 0 \) eV (as n approaches infinity)
- \( E_1 = -\frac{13.6 \cdot 3^2}{1^2} = -\frac{13.6 \cdot 9}{1} = -122.4 \, \text{eV} \)
\( I.P. = E_{\infty} - E_1 = 0 - (-122.4) = 122.4 \, \text{eV} \)
The electronic configuration of carbon (C) is \( 1s^2 \, 2s^2 \, 2p^2 \). When 5 electrons are removed, the configuration of \( C^{+5} \) will be \( 1s^1 \).
For \( C^{+5} \) (where \( Z = 6 \)):
- The energy for the first level (n=1) is:
\( E_1 = -\frac{13.6 \cdot 6^2}{1^2} = -\frac{13.6 \cdot 36}{1} = -489.6 \, \text{eV} \)
The 6th ionization potential corresponds to the energy required to remove the last electron from \( C^{+5} \):
\( I.P. = E_{\infty} - E_1 = 0 - (-489.6) = 489.6 \, \text{eV} \)
The 6th ionization potential of carbon is 489.6 eV.
Topper's Solved these Questions
- ATOMIC STRUCTURE Book:MOTIONChapter:ATOMIC STRUCTURE Exercise:EXERCISE-2 (LEVEL-I)
Explore 53 Videos
- ATOMIC STRUCTURE Book:MOTIONChapter:ATOMIC STRUCTURE Exercise:EXERCISE-2 (LEVEL-II)
Explore 31 Videos
- ATOMIC STRUCTURE Book:MOTIONChapter:ATOMIC STRUCTURE Exercise:EXERCISE-4 (LEVEL-II)
Explore 18 Videos
- AROMATIC COMPOUNDBook:MOTIONChapter:AROMATIC COMPOUNDExercise:Exercise - 4 | Level-II
Explore 16 Videos
- BIOMOLECULES & POLYMERSBook:MOTIONChapter:BIOMOLECULES & POLYMERSExercise:EXERCISE - 4 (LEVEL II)
Explore 17 Videos
Similar Questions
Knowledge Check
If the IP of Na is 5.48 eV, the ionsation potential of K will be
Asame as that of NaB4.34 eVC5.68 eVD10.88 eVIf 7th term of G.P. is 8 times the 4th term, find the common ratio of the G.P.
A2B3C1D4If pth term of an A. P. is q and the qth term is p. Find the first term of an A.P.
AoBqCpDp+q−1
The sum of the 4thand8th terms of an A.P. is 24 and the sum of the 6thand10th terms is 44. Find the first three terms of the A.P.
View SolutionThe first term of a G.P. is -3. If the 4th term of this G.P. is the square of the 2nd term, then find its 7th term.
View SolutionThe energy of separationof an of an electron is 30.6eV moving in an orbit of Li+2 Find out the number of waves made by the electron in one complete revolution in the orbit
View SolutionI.P (in KJ/mole) of Li and Be are given below:
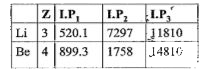
I.P2 of Li is much higher than that of in case of Be I.P3 is much higher than that I.P1 Explain.
View SolutionSupposing the I.P.(lonisation potential) of hydrogen like species is "960eV" .Find out the value of principal quantum number having the energy equal to -60eV:
View Solution