By what angle the mirror must be rotated such that the reflected ray becomes vertical. Let the light ray incident on the plane mirror with an angle of incidence theta with respect to horizontal
To solve the problem of determining the angle by which the mirror must be rotated so that the reflected ray becomes vertical, we can follow these steps:
1. Understand the Initial Setup:
- A plane mirror is positioned such that a light ray strikes it at an angle θ with respect to the horizontal.
- The angle of incidence i with respect to the normal is 90∘−θ.
2. Determine the Angle of Reflection:
- According to the law of reflection, the angle of reflection r is equal to the angle of incidence i. Therefore, r=90∘−θ.
3. Identify the Desired Condition:
- We want the reflected ray to become vertical. A vertical ray makes an angle of 90∘ with the horizontal.
4. Calculate the Required Change in Angle:
- The reflected ray currently makes an angle of 90∘−θ with the horizontal.
- To make it vertical, we need to change this angle to 90∘.
- The change in angle required is:
Δr=90∘−(90∘−θ)=θ
5. Relate Mirror Rotation to Ray Rotation:
- When the mirror is rotated by an angle ϕ, the reflected ray changes direction by 2ϕ (since the angle of reflection changes by twice the angle of rotation of the mirror).
6. Set Up the Equation:
- We need the change in the reflected ray's angle to equal the angle needed to make it vertical:
2ϕ=θ
7. Solve for the Mirror Rotation Angle:
- Rearranging gives:
ϕ=θ2
Conclusion:
The angle by which the mirror must be rotated to make the reflected ray vertical is θ2.
- A plane mirror is positioned such that a light ray strikes it at an angle θ with respect to the horizontal.
- The angle of incidence i with respect to the normal is 90∘−θ.
- According to the law of reflection, the angle of reflection r is equal to the angle of incidence i. Therefore, r=90∘−θ.
- We want the reflected ray to become vertical. A vertical ray makes an angle of 90∘ with the horizontal.
- The reflected ray currently makes an angle of 90∘−θ with the horizontal.
- To make it vertical, we need to change this angle to 90∘.
- The change in angle required is:
Δr=90∘−(90∘−θ)=θ
- When the mirror is rotated by an angle ϕ, the reflected ray changes direction by 2ϕ (since the angle of reflection changes by twice the angle of rotation of the mirror).
- We need the change in the reflected ray's angle to equal the angle needed to make it vertical:
2ϕ=θ
- Rearranging gives:
ϕ=θ2
The angle by which the mirror must be rotated to make the reflected ray vertical is θ2.
Topper's Solved these Questions
- RAY OPTICSBOOK - MOTIONCHAPTER - RAY OPTICSEXERCISE - Questions For Practice
29 Videos
- RAY OPTICSBOOK - MOTIONCHAPTER - RAY OPTICSEXERCISE - Exercise-1
102 Videos
- RADIOACTIVITY & NUCLEAR PHYSICSBOOK - MOTIONCHAPTER - RADIOACTIVITY & NUCLEAR PHYSICSEXERCISE - Exercise-3
81 Videos
- ROTATIONAL MOTION BOOK - MOTIONCHAPTER - ROTATIONAL MOTION EXERCISE - Exercise - 3 ( Section-B )
24 Videos
Similar Questions
Knowledge Check
A light ray is incident normally on a plane mirror. The angle of reflection will be
A120∘B90∘C45∘D0∘A ray of light is incident on a plane mirror at an angle of incidence 40∘. The ray, after reflection, is deviated through
A30∘B60∘C90∘D100∘Tile angle of deviation for an incident ray on a plane mirror at angle of incidence of 60* is-
A30∘B90∘C60∘D120∘
If a light ray incident on a plane mirror makes an angle 30∘ with it, find the angle of incidence and angle of reflection.
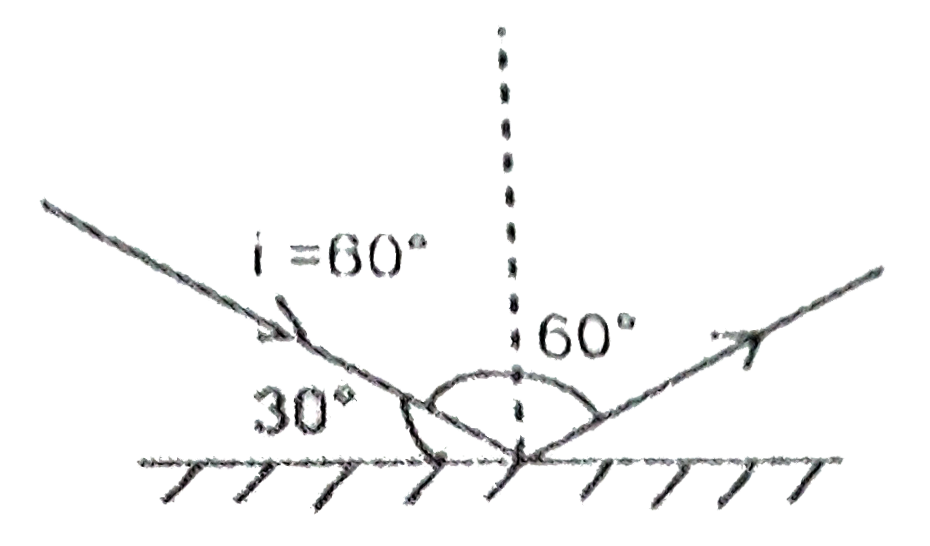
View SolutionA light ray inclined at an angle 30∘ with the horizontal falls on a plane mirror and after reflection light ray becomes vertical. At what angle the plane mirror is inclined with the horizontal?
View SolutionIf a ray of light is incident on a plane mirror at an angle of incidence of 30∘, then deviation produced by the mirror is
View SolutionA ray of light is incident on a plane mirror at an angle of incidence of 30∘. The deviation produced by the mirror is
View SolutionA ray of light is incident at an angle of 35∘ on a plane mirror. What is angle θ ?

View Solution
