The total length of a sonometer wire between fixed ends is 110 cm. To bridges are placed to divide the length of the wire in ratio 6 : 3 : 2. What is the minimum common frequency with which three parts can vibrate? Also calculate the ratio of number of loops formed in three parts. The tension in the wire is 400 N and the mass per unit length is 0.01kg/m .
To solve the problem step by step, we will follow these steps:
Step 1: Determine the lengths of the three parts
Given the total length of the sonometer wire is 110 cm and the ratio of the lengths is 6:3:2, we can express the lengths of the three parts as:
- Length of part 1 (L1) = 6k
- Length of part 2 (L2) = 3k
- Length of part 3 (L3) = 2k
Since the total length is 110 cm, we can write:
6k+3k+2k=110
11k=110
k=10 cm
Now, substituting k back into the expressions for lengths:
- L1 = 6k = 60 cm
- L2 = 3k = 30 cm
- L3 = 2k = 20 cm
Step 2: Calculate the wave velocity (v)
The wave velocity on the string can be calculated using the formula:
v=√Tμ
where:
- T = tension in the wire = 400 N
- μ = mass per unit length = 0.01 kg/m
Substituting the values:
v=√4000.01=√40000=200 m/s
Step 3: Calculate the frequencies of vibration for each part
The frequency of vibration for a string can be expressed as:
fn=nv2L
where:
- n = number of loops (harmonics)
- L = length of the string
Let’s denote the number of loops for each part as n1, n2, and n3.
Thus, we have:
- For part 1:
f1=n1v2L1=n1⋅2002⋅0.6=n1⋅2001.2=n1⋅2001.2
- For part 2:
f2=n2v2L2=n2⋅2002⋅0.3=n2⋅2000.6
- For part 3:
f3=n3v2L3=n3⋅2002⋅0.2=n3⋅2000.4
Step 4: Set the frequencies equal to find the common frequency
To find the minimum common frequency, we set the frequencies equal:
n1⋅2001.2=n2⋅2000.6=n3⋅2000.4
This simplifies to:
n11.2=n20.6=n30.4
Let’s denote this common ratio as k:
n1=1.2k,n2=0.6k,n3=0.4k
Step 5: Find the ratio of the number of loops
To express the number of loops in the simplest integer form, we can choose k such that all values are integers. The smallest integer k that satisfies this is 10:
- n1 = 1.2 * 10 = 12
- n2 = 0.6 * 10 = 6
- n3 = 0.4 * 10 = 4
Thus, the ratio of the number of loops formed in the three parts is:
n1:n2:n3=12:6:4
This can be simplified to:
3:1.5:1
Final Answer
The minimum common frequency with which the three parts can vibrate is:
f=n1⋅2001.2=12⋅2001.2=2000 Hz
And the ratio of the number of loops formed in the three parts is:
3:1.5:1
Given the total length of the sonometer wire is 110 cm and the ratio of the lengths is 6:3:2, we can express the lengths of the three parts as:
- Length of part 1 (L1) = 6k
- Length of part 2 (L2) = 3k
- Length of part 3 (L3) = 2k
6k+3k+2k=110
11k=110
k=10 cm
- L1 = 6k = 60 cm
- L2 = 3k = 30 cm
- L3 = 2k = 20 cm
The wave velocity on the string can be calculated using the formula:
v=√Tμ
where:
- T = tension in the wire = 400 N
- μ = mass per unit length = 0.01 kg/m
v=√4000.01=√40000=200 m/s
The frequency of vibration for a string can be expressed as:
fn=nv2L
where:
- n = number of loops (harmonics)
- L = length of the string
- For part 1:
f1=n1v2L1=n1⋅2002⋅0.6=n1⋅2001.2=n1⋅2001.2
- For part 2:
f2=n2v2L2=n2⋅2002⋅0.3=n2⋅2000.6
- For part 3:
f3=n3v2L3=n3⋅2002⋅0.2=n3⋅2000.4
To find the minimum common frequency, we set the frequencies equal:
n1⋅2001.2=n2⋅2000.6=n3⋅2000.4
n11.2=n20.6=n30.4
n1=1.2k,n2=0.6k,n3=0.4k
To express the number of loops in the simplest integer form, we can choose k such that all values are integers. The smallest integer k that satisfies this is 10:
- n1 = 1.2 * 10 = 12
- n2 = 0.6 * 10 = 6
- n3 = 0.4 * 10 = 4
n1:n2:n3=12:6:4
This can be simplified to:
3:1.5:1
The minimum common frequency with which the three parts can vibrate is:
f=n1⋅2001.2=12⋅2001.2=2000 Hz
And the ratio of the number of loops formed in the three parts is:
3:1.5:1
Topper's Solved these Questions
- SOUND WAVESBOOK - MOTIONCHAPTER - SOUND WAVESEXERCISE - Questions for practice
12 Videos
- SOUND WAVESBOOK - MOTIONCHAPTER - SOUND WAVESEXERCISE - Exercise - 1
82 Videos
- SOUND WAVESBOOK - MOTIONCHAPTER - SOUND WAVESEXERCISE - EXERCISE - 4 ( LEVEL II)
24 Videos
- SIMPLE HARONIC MOTION BOOK - MOTIONCHAPTER - SIMPLE HARONIC MOTION EXERCISE - EXERCISE -4 (Leve-II) [ JEE 2011]
11 Videos
- SURFACE TENSIONBOOK - MOTIONCHAPTER - SURFACE TENSIONEXERCISE - Exercise - 3 | Section - B Previous Year Problems | AIEEE
12 Videos
Similar Questions
The length of a sonometer wire between two fixed ends is 110cm. Where should the two bridges the placed so as to divide the wire into three segments, whose fundamental frequencies are in the ration1:2:3?
View SolutionThe length of a sonometer wire between two fixed ends is 110cm. Where should the two bridges the placed so as to divide the wire into three segments, whose fundamental frequencies are in the ration1:2:3?
View Solution
Knowledge Check
The total length of a sonometer wire fixed between two bridges is 110 cm. Now, two more bridges are placed to divide the length of the wire in the ratio 6:3:2. If the tension in the wire is 400 N and the mass per unit length of the wire is 0.01 kg m−1, then the minimum common frequency with which all the three parts can vibrate, is
A1000 HzB1100 HzC100 HzD110 HzThe length of a sonometer wire between two fixed ends is 110cm. Where should the two bridges the placed so as to divide the wire into three segments, whose fundamental frequencies are in the ration1:2:3?
A60 cm and 90 cmB30 cm and 60 cmC30 cm and 90 cmD40 cm and 80 cmThe length of a sonometer wire is 1m. Where should be the bridge placed to divide the wire into 2 segments, whose fundamental frequencies are in the ratio 1:2 ?
AAt 13 m from the left endBAt 23 m from the left endCAt the centreDAt 80 cm from the left end
Length of a sonometer wire is 1.21m. Find the length of the three segments for fundamental frequencies to be in the ratio 1:2:3.
View SolutionLength of a sonometer wire is 1.21m. Find the length of the three segments for fundamental frequencies to be in the ratio 1:2:3.
View SolutionA sonometer wire has a total length of 1m between the fixed ends. Where should the two bridges be placed below the wire so that the three segments of the wire have their fundamental frequencies in the ratio 1 : 2 : 3 ?
View SolutionThe length of sonometer wire is doubled and the tension is increases four times. The frequency will
View SolutionThe length of the wire shown in the figure between the pulleys is 1.5m and its mass is 12.0g. The frequency of vibration with which the wire vibrates in three loops forming antinode at the mid point of the wire is - (Take g=9.8m/s2)
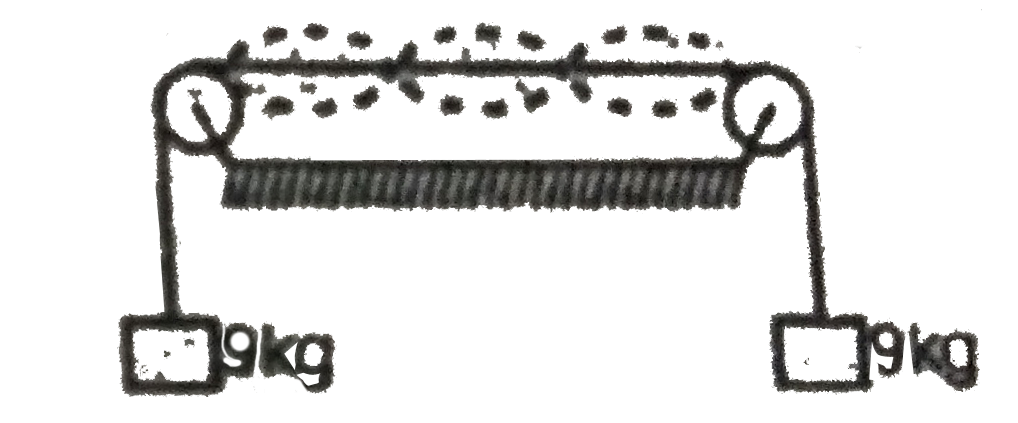
View Solution
