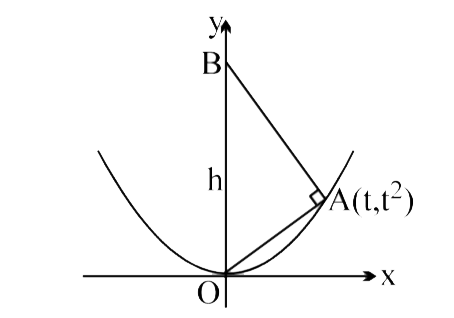
The figure shows a right triangle with its hypotenuse OB along the y-axis and its vertex A on the parabola y=x2. Let h represents the length of the hypotenuse which depends on the x- coordinate of the point A. The values of Limx→0(h) equals
0
1/2
1
2
The correct Answer is:C
Step by step video, text & image solution for The figure shows a right triangle with its hypotenuse OB along the y-axis and its vertex A on the parabola y =x^(2). Let h represents the length of the hypotenuse which depends on the x- coordinate of the point A. The values of underset( x rarr 0 ) ( "Lim") ( h ) equals by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LIMITBOOK - MOTIONCHAPTER - LIMITEXERCISE - EXERCISE -2( LEVEL-I)
16 Videos
- LIMITBOOK - MOTIONCHAPTER - LIMITEXERCISE - EXERCISE-2(LEVEL-2)
5 Videos
- LIMITBOOK - MOTIONCHAPTER - LIMITEXERCISE - EXERCISE-4
17 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - MOTIONCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise -4 Level -II
7 Videos
- MATRICES BOOK - MOTIONCHAPTER - MATRICES EXERCISE - Exercise - 4 (Level-II)
28 Videos
Similar Questions
The figure shows a right triangle with its hypotenuse OB along the Y-axis and its vertex A on the parabola y=x2.
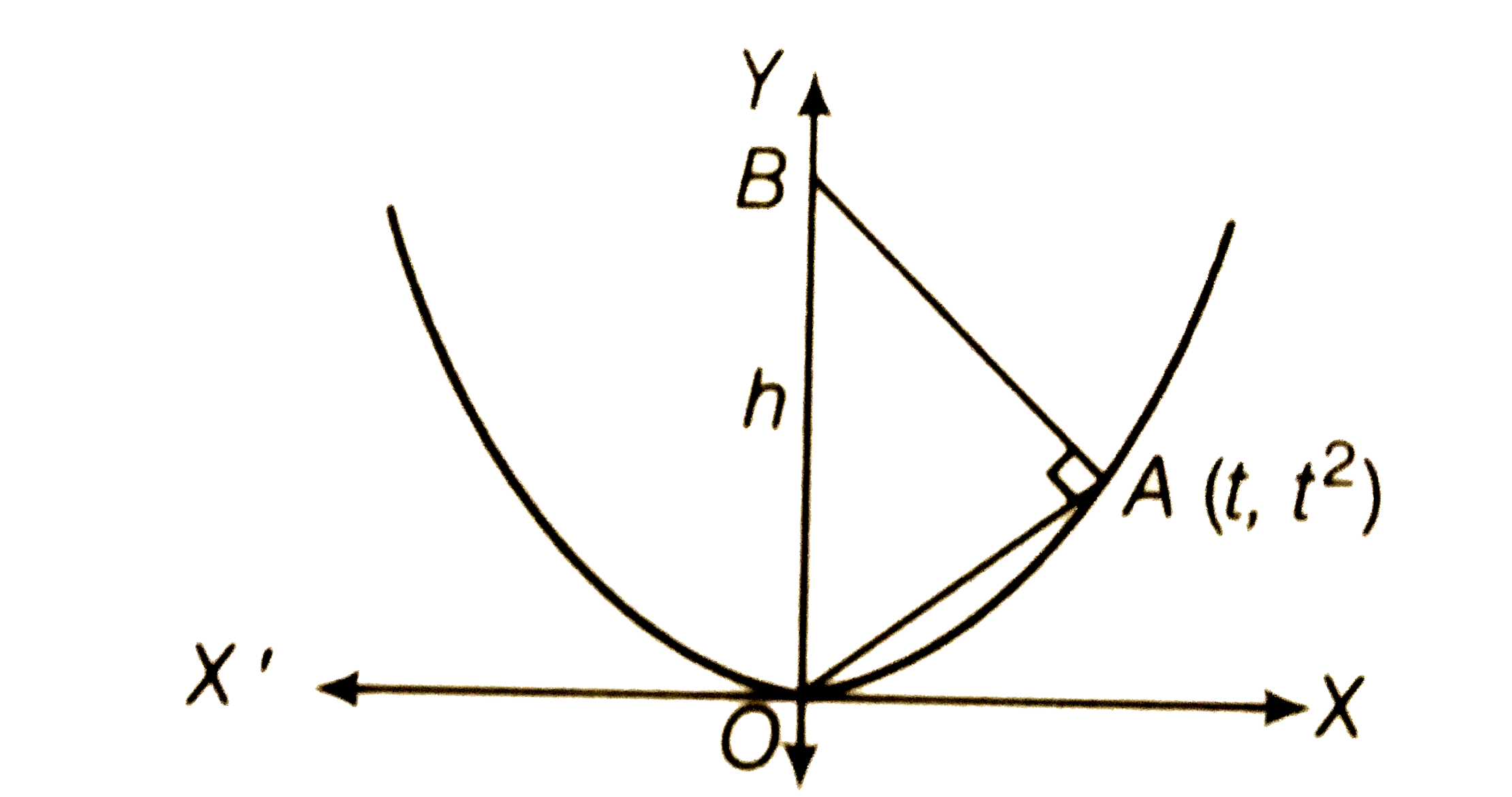
Let h represents the length of the hypotenuse which depends on the x-coordinate of the point A. The value of limt→00(h) is equal to
View SolutionThe hypotenuse of a right triangle with its legs of lengths 3x×4x is
View Solution
Knowledge Check
An isosceles right angled triange is in scribed in the circle x2+y2=r2. If the coordinates of an end of the hypotenuse are (a,b) the coordinates of the vertex are
A(−a,−b)B(b,−a)C(b,a)D(−b,−a)If the vertex of a parabola is (0,2), directrix on x-axis then its focus is :
A(0,0)B(0,4)C(4,0)DNone of these.
The area of a right angle triangle with its hypotenuse and perincter equal to x and y respectivelyis equal to
View SolutionFind the area of the triangle formed by the lines joining the vertex of the parabola x2=12y to the ends of its latus rectum
View SolutionFind the area of the triangle formed by the lines joining the vertex of the parabola x2=8y to the ends of its latus rectum.
View SolutionIf the area of the triangle whose one vertex is at the vertex of the parabola, y2+4(x−a2)=0 and the other two vertices are the points of intersection of the parabola and Y-axis, is 250 sq units, then a value of 'a' is
View SolutionThe triangle ABC, right angled at C, has median AD, BE and CF, AD lies along the line y=x+3, BE lies along the line y lies along the line y=2x+4. If the length of the hypotenuse is 60, fin the area of the triangle ABC (in sq units).
View SolutionFind the area of the triangle formed by the lines joining the vertex of the parabola x2=12y to the ends of its latus rectum.
View Solution
