The frequency distribution for x is an given below. What is the range of f? <br>{:(x,f),(0,1),(1,5),(2,4),(3,4):}
0
1
3
4
The correct Answer is:D
To find the range of the frequency distribution given by the pairs (x,f), we will follow these steps:
1. Identify the values of f: From the frequency distribution, we have the following values of f:
- For x=0, f=1
- For x=1, f=5
- For x=2, f=4
- For x=3, f=4
Therefore, the values of f are: 1,5,4,4.
2. Determine the maximum value of f: The maximum value from the set of f values is:
Maximum of f=5
3. Determine the minimum value of f: The minimum value from the set of f values is:
Minimum of f=1
4. Calculate the range of f: The range is calculated using the formula:
Range=Maximum−Minimum
Substituting the values we found:
Range=5−1=4
Thus, the range of f is 4.
- For x=0, f=1
- For x=1, f=5
- For x=2, f=4
- For x=3, f=4
Maximum of f=5
Minimum of f=1
Range=Maximum−Minimum
Substituting the values we found:
Range=5−1=4
Similar Questions
Find the missing frequencies in the following frequency distribution if it is known that the mean of the distribution is 1.46. No. of accident (x), 0, 1 , 2 , 3, 4, 5 , Total Frequency is 200 and no. of days (f): 46 , ? , ? , 25 , 10 , 5
View SolutionKind the range of the function f(x)=1x+4−2.
View Solution
Knowledge Check
What is the range of the function f(x)=3x−5−1?
AAll real numbersBAll real numbers except 5CAll real numbers except 0DAll real numbers except-1If a function f:[2,∞)→R is defined by f(x)=x2−4x+5, then the range of f is
ARB[1,∞)C[4,∞)D[5,∞)
Find the missing frequencies in the following frequency distribution if it is known that the mean of the distribution is 1.46. No of accident (x) 0 1 2 3 4 5 Total Frequency (f): 46 ? ? 25 10 5 200
View SolutionFind the missing frequencies in the following frequency distribution if it is known that the mean of the distribution is 1.46. No. of accident (x) 0 1 2 3 4 5 Total Frequency (f): 46 ? ? 25 10 5 200
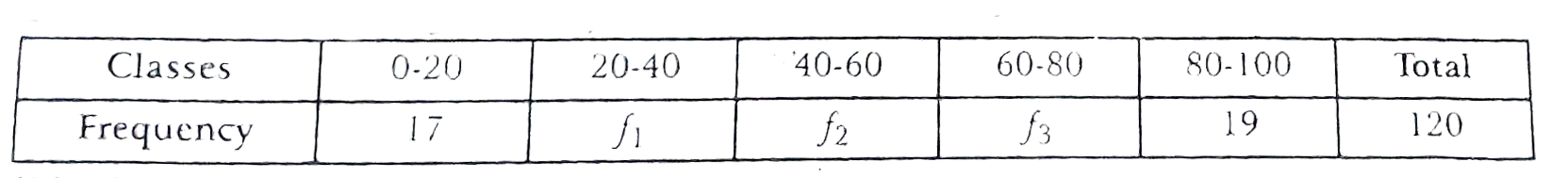
View SolutionThe mean of the following frequency distribution is 50 Find f1 f2 f3 if f2 : f3 = 4 : 3:
View SolutionIf the mean of the distribution is 2.6, then the value of y is
Variate x12345Frequency f of x45y12
View SolutionFind the range of the function f(x)=(1+x+x2)(1+x4)x3
View SolutionIf f(x) =4x−2x+1+5, then range of f(x) is
View Solution