Suppose x is divisible by 8 but not by 3. Then which of the following CANNOT be an integer?
x/2
x/4
x/6
x/8
The correct Answer is:C
To solve the problem, we need to analyze the conditions given: x is divisible by 8 but not by 3. We will evaluate the divisibility of x with respect to the options provided.
1. Understanding Divisibility by 8:
Since x is divisible by 8, we can express x as:
x=8k
for some integer k.
2. Divisibility by 2 and 4:
Because x is divisible by 8, it is also divisible by 2 and 4. Therefore:
x2=8k2=4k(which is an integer)
x4=8k4=2k(which is an integer)
3. Checking Divisibility by 3:
The problem states that x is not divisible by 3. Therefore, we need to check the expression x6:
x6=8k6=4k3
For 4k3 to be an integer, k must be divisible by 3. However, since x is not divisible by 3, k cannot be chosen such that 4k is divisible by 3. Hence, 4k3 is not guaranteed to be an integer.
4. Conclusion:
Since x is divisible by 8 but not by 3, x6 cannot be an integer. Thus, the answer is:
The expression that cannot be an integer is x6.
Final Answer:
The option that cannot be an integer is x6.
---
Since x is divisible by 8, we can express x as:
x=8k
for some integer k.
Because x is divisible by 8, it is also divisible by 2 and 4. Therefore:
x2=8k2=4k(which is an integer)
x4=8k4=2k(which is an integer)
The problem states that x is not divisible by 3. Therefore, we need to check the expression x6:
x6=8k6=4k3
For 4k3 to be an integer, k must be divisible by 3. However, since x is not divisible by 3, k cannot be chosen such that 4k is divisible by 3. Hence, 4k3 is not guaranteed to be an integer.
Since x is divisible by 8 but not by 3, x6 cannot be an integer. Thus, the answer is:
The expression that cannot be an integer is x6.
The option that cannot be an integer is x6.
Similar Questions
Knowledge Check
In n is an integer, which of the following CANNOT be an integer?
An−22B√1n2+2C2n+1D√n2+342x8 divisible by 4 ?
A3,1,6,8 and 9B1,2,5,7 and 8C3,4,6,8 and 9D0,2,4,6 and 8When positive integer x is divided by 5, the remainder is 2. When positive integer y is divided by 4, the remainder is 1. which of the following values CANNOT be the sum of x and y?
A12B13C14D16
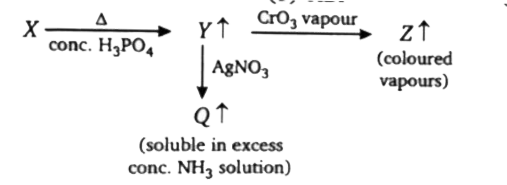
Which of the following anion cannot be in X?
View SolutionIf −3<2x+4<9, which of the following CANNOT be a possible value of x?
View SolutionWhich of the following are divisible by both 2 and 3?
View SolutionIf x3−9x=9−x2, which of the following CANNOT be the value of x ?
View SolutionIf x2−63x−64=0 and p and n are integers such that pn=x, which of the following CANNOT be a value for p?
View Solution
