If (a−b)(a+b)=7×13, then which of the following pairs could be the value of a and b. respectively?
−10,3
10,3
−3,−10
−10,−3
The correct Answer is:A, B, D
To solve the equation (a−b)(a+b)=7×13, we can follow these steps:
Step 1: Calculate the right-hand side
First, we calculate the right-hand side of the equation:
7×13=91
So, we rewrite the equation:
(a−b)(a+b)=91
Step 2: Identify possible pairs
Since 91 can be expressed as a product of pairs of factors, we can consider the following pairs:
1. (a−b,a+b)=(7,13)
2. (a−b,a+b)=(−7,−13)
3. (a−b,a+b)=(13,7)
4. (a−b,a+b)=(−13,−7)
Step 3: Solve each case
Case 1: a−b=7 and a+b=13
Add the two equations:
(a−b)+(a+b)=7+13⟹2a=20⟹a=10
Substituting a=10 into a+b=13:
10+b=13⟹b=3
So, one solution is (a,b)=(10,3).
Case 2: a−b=−7 and a+b=−13
Add the two equations:
(a−b)+(a+b)=−7−13⟹2a=−20⟹a=−10
Substituting a=−10 into a+b=−13:
−10+b=−13⟹b=−3
So, another solution is (a,b)=(−10,−3).
Case 3: a−b=13 and a+b=7
Add the two equations:
(a−b)+(a+b)=13+7⟹2a=20⟹a=10
Substituting a=10 into a+b=7:
10+b=7⟹b=−3
So, another solution is (a,b)=(10,−3).
Case 4: a−b=−13 and a+b=−7
Add the two equations:
(a−b)+(a+b)=−13−7⟹2a=−20⟹a=−10
Substituting a=−10 into a+b=−7:
−10+b=−7⟹b=3
So, another solution is (a,b)=(−10,3).
Summary of Solutions
The possible pairs of (a,b) are:
1. (10,3)
2. (−10,−3)
3. (10,−3)
4. (−10,3)
First, we calculate the right-hand side of the equation:
7×13=91
So, we rewrite the equation:
(a−b)(a+b)=91
Since 91 can be expressed as a product of pairs of factors, we can consider the following pairs:
1. (a−b,a+b)=(7,13)
2. (a−b,a+b)=(−7,−13)
3. (a−b,a+b)=(13,7)
4. (a−b,a+b)=(−13,−7)
Add the two equations:
(a−b)+(a+b)=7+13⟹2a=20⟹a=10
Substituting a=10 into a+b=13:
10+b=13⟹b=3
So, one solution is (a,b)=(10,3).
Add the two equations:
(a−b)+(a+b)=−7−13⟹2a=−20⟹a=−10
Substituting a=−10 into a+b=−13:
−10+b=−13⟹b=−3
So, another solution is (a,b)=(−10,−3).
Add the two equations:
(a−b)+(a+b)=13+7⟹2a=20⟹a=10
Substituting a=10 into a+b=7:
10+b=7⟹b=−3
So, another solution is (a,b)=(10,−3).
Add the two equations:
(a−b)+(a+b)=−13−7⟹2a=−20⟹a=−10
Substituting a=−10 into a+b=−7:
−10+b=−7⟹b=3
So, another solution is (a,b)=(−10,3).
The possible pairs of (a,b) are:
1. (10,3)
2. (−10,−3)
3. (10,−3)
4. (−10,3)
Similar Questions
Knowledge Check
From the figure, which of the following could be the value of b?
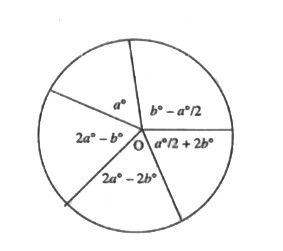 A20B40C50D70
A20B40C50D70If cos=xsiny, then which of the following pairs of angle measures could NOT be the values of x and y, respectively ?
Aπ4,π4Bπ6,π3Cπ8,3π8Dπ2,π2If b≠0and3a+bb=112, which of the following could be the value of ab ?
A32B72C92DIt is not possible to determine a value of ab.
If −4<a<4and−2<b<−1, which of the following could NOT be the value of ab?
View SolutionIf a−bb=37 , which of the following must also be true ?
View SolutionIf a+b=15,b+c=10, and a+c=13, which of the following is true ?
View Solution2x2−28x+98=a(x−b)2
In the expression above, a>1 and both a and b are constants. Which of the following could be the value of b ?
View Solution{(y=(1)/(2)x-2), (y=-x^(2)+1):}
If (a, b) is a solution to the system of equations above, which of the following could be the value of b?
View Solution