If |−3a+15|=6 and |−2b+12|=4, what is the greatest possible value of ab ?
The correct Answer is:56
To solve the problem, we need to find the values of a and b from the given equations involving absolute values, and then determine the greatest possible value of the product ab.
Step 1: Solve the first equation |−3a+15|=6
The absolute value equation can be split into two cases:
1. Case 1:
−3a+15=6
Rearranging gives:
−3a=6−15⟹−3a=−9⟹a=−9−3=3
2. Case 2:
−3a+15=−6
Rearranging gives:
−3a=−6−15⟹−3a=−21⟹a=−21−3=7
Thus, the possible values for a are 3 and 7.
Step 2: Solve the second equation |−2b+12|=4
Similarly, we split this absolute value equation into two cases:
1. Case 1:
−2b+12=4
Rearranging gives:
−2b=4−12⟹−2b=−8⟹b=−8−2=4
2. Case 2:
−2b+12=−4
Rearranging gives:
−2b=−4−12⟹−2b=−16⟹b=−16−2=8
Thus, the possible values for b are 4 and 8.
Step 3: Calculate the products ab
Now we have the possible pairs of (a,b):
- (3,4)
- (3,8)
- (7,4)
- (7,8)
Calculating the products:
1. ab=3×4=12
2. ab=3×8=24
3. ab=7×4=28
4. ab=7×8=56
Step 4: Determine the greatest possible value of ab
From the calculated products, the greatest possible value of ab is:
Greatest value of ab=56
Final Answer:
The greatest possible value of ab is 56.
---
−3a+15=6
Rearranging gives:
−3a=6−15⟹−3a=−9⟹a=−9−3=3
−3a+15=−6
Rearranging gives:
−3a=−6−15⟹−3a=−21⟹a=−21−3=7
−2b+12=4
Rearranging gives:
−2b=4−12⟹−2b=−8⟹b=−8−2=4
−2b+12=−4
Rearranging gives:
−2b=−4−12⟹−2b=−16⟹b=−16−2=8
- (3,4)
- (3,8)
- (7,4)
- (7,8)
1. ab=3×4=12
2. ab=3×8=24
3. ab=7×4=28
4. ab=7×8=56
Greatest value of ab=56
The greatest possible value of ab is 56.
Similar Questions
Knowledge Check
If −6<−4r+10≤2, what is the least possible value of 4r+3?
A2B5C8D11IF −207<−3z+6<−115, what is the greatest possible integer value of 9z-18?
A6B7C8D9a4−12aa2−72=0
Which of the following is the greatest possible value of a ?A√6+√3B√6(1+√3)C12D6(1+√3)
IF −1≤a≤2and−3≤b≤2, What is the greatest possible value of (a+b)(b-a)?
View SolutionIf −3x+4≤−11andx>0, what ist he greatest possible value for x?
View Solution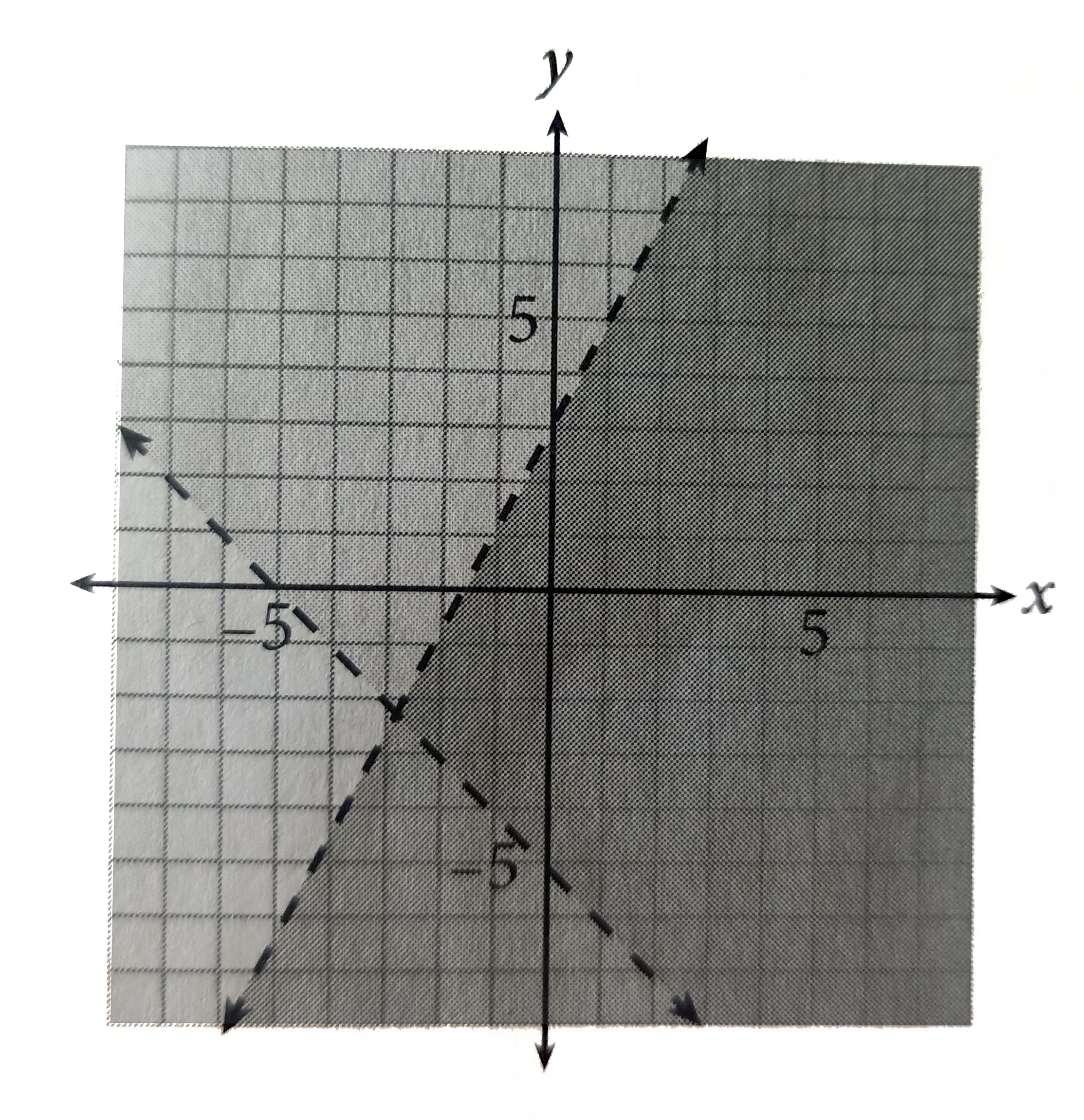
{y>−x−5y<2x+3
The figure above shows the solution for the system of inequalities shown. Suppose (a,b) is a solution to the system. If a=0, what is the greatest possible interger value of b ?
View SolutionIf a and b are integers and −4≤a≤3and−4≤b≤5, what is the maximum possible value for ab?
View SolutionThe value of a certain recangular solid is 12x. If the dimensions of the solid are the intigers x,y,andz, what is the greatest possible value of z?
View Solution
