Let f:R→R be a continuous function defined by f(x)=1ex+2e−x
Statement -1 : f(x)=13, for some c∈R.
Statement -2, 0<f(x)≤12√2∀x∈R.Solution in Telugu
Statement-1 is true, Statement-2 is true, Statement -2 is a correct explanation for statement-1
Statement-1 is true, Statement-2 is true, Statement -2 is not a correct explanation for statement-1
Statement-1 is true, Statement-2 is false
Statement-1 is false, Statement- is true
The correct Answer is:A
Step by step video, text & image solution for Let f: R to R be a continuous function defined by f(x)=(1)/(e^(x)+2e^(-x)) Statement -1 : f(x)=(1)/(3), for some c in R. Statement -2, 0 ltf(x) le (1)/(2sqrt(2))AAx in R. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LIMITS AND CONTINUITYBOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - LIMITS AND CONTINUITYEXERCISE - SET-3Match The Following
2 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - EXERCISE 2 (SPECIAL TYPE QUESTIONS) (SET - 4)
5 Videos
- LINEAR PROGRAMMING [APPENDIX-2]BOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - LINEAR PROGRAMMING [APPENDIX-2]EXERCISE - Exercise -1
33 Videos
Similar Questions
Match the following
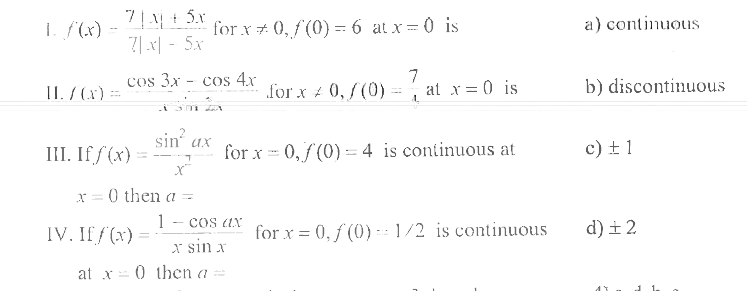
View SolutionA : The maximum value of f(x)=Sin−1x+Cos−1x−Tan−1x is 3π/4
R : Sin−1x+Cos−1x=π/2∀x∈R
View SolutionIf 2x+y≥10,x+2y≥10, then the lesat value of F=x+y is
View SolutionLet f: R R be a continuous function defined by f(x)=1ex+2e−x . Statement-1: f(c)=13, for some c∈R . Statement-2: 0<f(x)≤12√2, for all x∈R . (1) Statement-1 is true, Statement-2 is true; Statement-2 is not the correct explanation for Statement-1 (2) Statement-1 is true, Statement-2 is false (3) Statement-1 is false, Statement-2 is true (4) Statement-1 is true, Statement-2 is true; Statement-2 is the correct explanation for Statement-1
View Solutionlet f:R rarr R be a continuous function defined by f(x)=(1)/(e^(x)+2e^(-x))
View SolutionLet f : R → R be a function defined by f ( x ) = 2 x − 5 ∀ x ∈ R . Then Write f−1.
View SolutionLet f : R → R be a function defined by f ( x ) = 4 x − 3 ∀ x ∈ R . Then Write f−1.
View SolutionStatement-1 : f:R→R,f(x)=x2log(|x|+1) is an into function.
and
Statement-2 : f(x)=x2log(|x|+1) is a continuous even function.
View SolutionLet the function f:R→R be defined byf(x)=x3−x2+(x−1)sinxandletg:R→R be an arbitrary function Let fg:R→R be the function defined by (fg)(x)=f(x)g(x). Then which of the folloiwng statements is/are TRUE ?
View SolutionLet f:R−{1}→R be defined by
f(x)=1+x1−x∀x∈R−{1} then for x≠±1,1+f(x)2f(x)f(x2)=………….
View SolutionLet f:R→R be a function defined by f(x+1)=f(x)−5f(x)−3∀x∈R. Then which of the following statement(s) is/are true?
View SolutionLet f:R→R be a continuous function defined by f(x)=1ex+2e−x . Statement-1: f(c)=13, for some c∈R . Statement-2: 0<f(x)≤12√2, for all x∈R . (1) Statement-1 is true, Statement-2 is true; Statement-2 is not the correct explanation for Statement-1 (2) Statement-1 is true, Statement-2 is false (3) Statement-1 is false, Statement-2 is true (4) Statement-1 is true, Statement-2 is true; Statement-2 is the correct explanation for Statement-1
View Solutionagreed f:RtoR The continuous function is defined by f(x)=1/(e^(x)+2e^(-x)) Statement - 1 c epsilon R for f(c)=1/3 Statement - 2 all x epsilon R for 0ltf(x)le1/(2sqrt(2))
View SolutionStatement - 1 x epsilon R If for |f(x)|le|x| Is then |f|,x=0 But continuous. Statement - 2: If f is continuous, |f| Will also be continuous.
View SolutionLet R→R be a continuous function define by f(x ) =1ex+2e
statement 1 : f(c)=13 for some c ∈ R
statement 2 0<f(x)≤12√2 for all x ∈ R
View Solution
