If :[−2,2]→R is defined by
f(x)=⎧⎨⎩√1+cx−√1−cxx for−2≤x<0x+3x+1 for 0≤x≤2
continuous on [ -2, 2] then c is equal to Solution in Telugu
3
32
3√2
2√3
The correct Answer is:B
Step by step video, text & image solution for If : [ -2 ,2 ] to R is defined by f(x) = {: { ((sqrt(1+cx) -sqrt(1-cx))/x , " for" -2 le x lt 0), ((x+3)/(x +1) , " for " 0 le x le 2):} continuous on [ -2, 2] then c is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LIMITS AND CONTINUITYBOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - LIMITS AND CONTINUITYEXERCISE - Exercise 2(Special Type Questions)
10 Videos
- LIMITS AND CONTINUITYBOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - LIMITS AND CONTINUITYEXERCISE - SET-3Match The Following
2 Videos
- LIMITS AND CONTINUITYBOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - LIMITS AND CONTINUITYEXERCISE - Exercise 1B( One Sided Limits)
71 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - EXERCISE 2 (SPECIAL TYPE QUESTIONS) (SET - 4)
5 Videos
- LINEAR PROGRAMMING [APPENDIX-2]BOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - LINEAR PROGRAMMING [APPENDIX-2]EXERCISE - Exercise -1
33 Videos
Similar Questions
I. Ltx→0(1+x)2−(1−x)2−2(1+x)3−(1−x)3−3
II. If f(x)=4−7x3x+4andLtx→2f(x)=l,Ltx→0f(x)=m then the equation whose roots are 1/l, 1/m is x2−1=0
View SolutionMatch the following
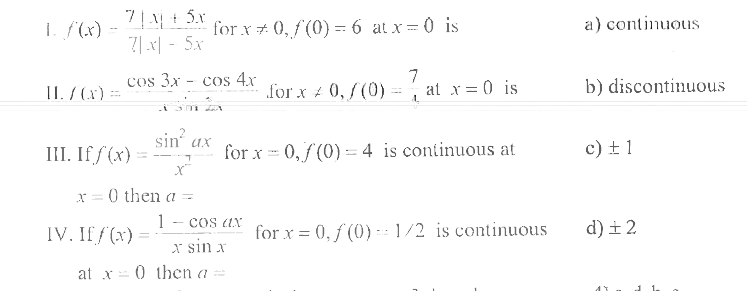
View SolutionLtx→0√x=
View SolutionA : The maximum value of f(x)=Sin−1x+Cos−1x−Tan−1x is 3π/4
R : Sin−1x+Cos−1x=π/2∀x∈R
View SolutionIf 2x+y≥10,x+2y≥10, then the lesat value of F=x+y is
View SolutionIf f(x)=⎧⎨⎩√1+px−√1−pxx,−1≤x<02x+1x−2,0≤x≤1 is continuous in [-1,1] then p is equal to
View SolutionIf f(x)={√1+kx−√1−kxx, if −12≤x<02x2+3x−2, if 0≤x≤1 is continuous at x = 0 , then k = ….
View SolutionIf f(x) =√1+px−√1−pxx, if −12≤x<0=3x2+2x−2, if 0≤x<1 is continuous on its domain , then : p =
View SolutionIf f(x)={20≤x<1c−2x1≤x≤2 is continuous at x = 1 , then c equals
View SolutionIf f(x)={√1+kx−√1−kxxfor−1≤x02x2+3x−2for0≤x≤1 is continuous at x = 0 then find k.
View SolutionIf f(x)=⎧⎪⎨⎪⎩√1+ax−√1−axx,−1≤x<0x2+2x−2,0≤x≤1
continuous on [−1,1], then a =
View Solution" If "f:[-2,2]rarr R" is defined by "f(x)={[(sqrt(1+cx)-sqrt(1-cx))/(x),,,-2<=x<0],[(x+3)/(x+1),0<=x<=2]}" is continuous "m[-2,2]" then "
View SolutionIf :[−2,2]→R is defined by
f(x)=⎧⎨⎩√1+cx−√1−cxx for−2≤x<0x+3x+1 for 0≤x≤2
continuous on [ -2, 2] then c is equal to
View SolutionIf f(x) = {:{((sqrt(1+ + kx ) - sqrt(1-kx))/x , " for" -1 le x lt 0) , ( 2x^(2) + 3x -2, " for " 0 le x le 1):}
continuous at x = 0 , then k is equal to
View SolutionIf a function f(x) defined by
f(x)=⎧⎪⎨⎪⎩aeX+be−x−1≤x<1cx21≤x≤3ax2+2cx3<x≤4
be continuous for some a,b,c ∈ R and f'(0)+F'(2)=e then the value of a is :
View Solution
